Sine Law - Ambiguous case - applet

The ambiguous case of the sine law, in solving triangle problems, is explored interactively using an applet.

Review The sine law is given by sin(a)/a = sin(b)/b = sin(g)/c Let us solve the triangle problem when we are given sides a and b and angle a, with a acute. 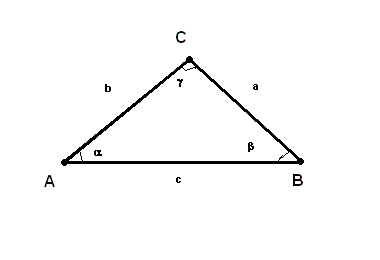The algebraic solution to this problem starts by finding sin(b) which is given by sin(b)=b*sin(a)/a Then find angles b and g and side c. For the triangle to exist sin(b) must be less than or equal to 1. There is also a GEOMETRIC solution to this problem which we describe below. step 1: draw a line (l) and draw point A on this line. step 2: draw a segment AC that makes an angle a with line (l). Segment AC has length b. step 3: Draw a circle with center at C and radius equal to side a and locate the points of intersection of the circle and line (l). Three cases are possible.    case 1: if a is smaller than h ,the circle does not intersect line (l) and the problem has no solution.    case 2: if a is equal to h, the circle touches line (l) at 1 point and the problem has one solution. Triangle ABC is a right triangle.    case 3: if a is larger than h, but smaller than b, the circle intersects line (l) at 2 points and the problem has 2 solutions: Triangle ABC and Triangle AB'C. This is called the ambiguous case.    case 4: if a is larger than or equal to b, the problem has one solution. step 4: draw the third side of the triangle(s) by joining C and B, and C and B'. The figures below show the case with two solutions. 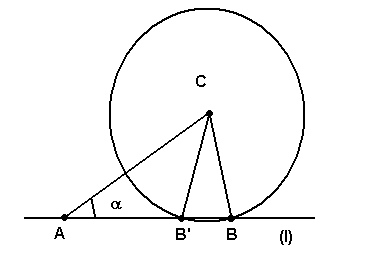Interactive Tutorial Your browser is completely ignoring the <APPLET> tag! click on the button above "click here to start" and MAXIMIZE the window obtained. Use the sliders to set parameters b and alpha to 3 and 30 degrees respectively if they are not already. Use the red point on the circle to increase or decrease the radius a of the circle. Find a value of a that correspond to a case with no solution as described above and check by calculations. Find a value of a that correspond to a case with 1 solution as described above and check by calculations.(this may be a little difficult to do , but a close value should be enough). Find a value of a that correspond to a case with 2 solutions as described above and check by calculations. Find a value of a that correspond to a case with 1 solution as described above and check by calculations. Set b and alpha to other values and explore further. More on sine law and triangle problems Sine Law Problems Sine Law Calculator and Solver. Triangle Problems