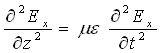
This tutorial explores wave propagation. Whether used in radio frequency systems, microwave systems, optical system or other, electromagnetic waves propagation obey Maxwell's equations. Here we try to examine one of the simplest solution to Maxwell's equation and understand its meaning. An electromagnetic field with only on component E x and independent of x and y is solution to the differential equation
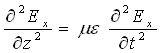
The above equation has many solutions. However one of the simplest and most useful is the one where time and z variations are sinusoidal and is given by.
The electric field component E x is a function of two variables: t and z. To study this function we will change time t in steps and plot E x as a function of z. This is done in the applet below.