Find Range of Sine Functions

Find the range of sine functions; examples and matched problems with their answers at the bottom of the page.

Graphical Analysis of Range of Sine Functions

The range of a function y = f(x) is the set of values y takes for all values of x within the domain of f.
What is the range of y = f(x) = sin(x)?
The domain of f above is the set of all values of x in the interval ( -∞ , +∞)
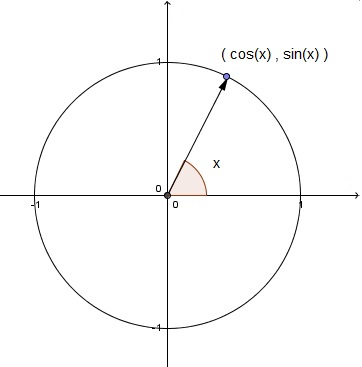
As x takes values from -∞ to +∞, sin(x) takes all values between -1 and 1 as shown in the unit circle below. Hence
-1 ≤ sin(x) ≤ 1 or -1 ≤ y ≤ 1
In general the range of any sine function of the form y = sin (b x + c) is given by
-1 ≤ sin(b x + c) ≤ 1 or -1 ≤ y ≤ 1

Examples with Solutions

Example 1:

Find the range of function f defined by
f(x) = - sin (x)

Solution to Example 1

Start with the range of the basic sine function (see discussion above) and write
- 1 ≤ sin(x) ≤ 1
Multiply all terms of the above inequality by -1 and change symbols of inequality to obtain
1 ≥ sin(x) ≥ - 1
which may also be written as
- 1 ≤ - sin(x) ≤ 1
Hence the range of - sin(x) is also given by the interval
[ - 1 , 1 ]

Matched Problem 1:

Find the range of function f defined by
f(x) = - sin (2 x)

Example 2

Find the range of function f defined by
f(x) = 2 sin (-3 x - π/6)

Solution to Example 2

The range of sin (-3 x - π/6) is given by
- 1 ≤ sin (-3 x - π/6) ≤ 1
Multiply all terms of the above inequality by 2 to obtain the inequality
- 2 ≤ 2 sin (-3 x - π/6) ≤ 2
The range of the given function f is written above in inequality form and may also be written in interval form as follows
[ -2 , 2 ]

Matched Problem 2:

Find the range of function f defined by
f(x) = - (1 / 5) sin ( x / π + π)

Example 3

Find the range of function f defined by
f(x) = 0.1 sin ( x / π + π) - 2

Solution to Example 3

The range of sin ( x / π + π) is given by
- 1 ≤ sin ( x / π + π) ≤ 1
Multiply all terms of the inequality by 0.1 to obtain
- 0.1 ≤ 0.1 sin ( x / π + π) ≤ 0.1
Add -2 to all terms of the above inequality to obtain
- 2.1 ≤ 0.1 sin ( x / π + π) -2 ≤ 1.9
The range of values of 0.1 sin ( x / π + π) -2 may also be written in interval form as follows
[ -2.1 , 1.9]

Matched Problem 3:

Find the range of function f defined by
f(x) = - 4 sin ( x / π + π) + 3

Answers to the Above Matched Problems

1) [-1 , 1]

2) [ - 1/5 , 1/5]

3) [-1 , 7]

Free Mathematics Tutorials