|
Problem : The square ABCD is inscribed inside the larger circle C1 and the smaller circle C2 is inscribed inside the same square. If A1 is the area of the large circle and A2 is the area of the small circle, what is the ratio A1 / A2?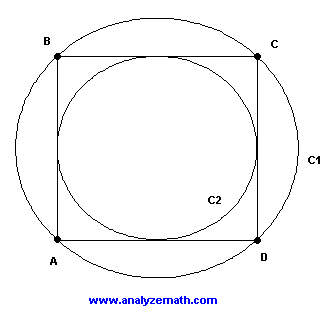
Solution to Problem :
- If x is the size of one side of the square, the small circle has a diameter of size d = x and its radius has a size of x / 2, hence its area A2 is given by
A2 = Pi (x/2) 2 = Pi x 2 / 4
- The radius R of the large circle is equal to D / 2 where D is the diagonal of the square. Pythagora's theorem gives
D 2 = x 2 + x 2
D = x sqrt (2)
R = x sqrt(2) / 2
- The area A1 of the large circle A1 is given by.
A1 = Pi (x sqrt(2) / 2) = Pi x 2 / 2
- Hence
A1 / A2 = (Pi x 2 / 2) / (Pi x 2 / 4) = 2
More references to geometry problems.
Geometry Tutorials, Problems and Interactive Applets.
|