
The semicircle (Thales) theorem [1] states that for a triangle inscribed in a semicircle of diameter \( AB \), as shown in the figure below, angle \( AOB \) has a size of \( 90^{\circ} \).

Note that none of the figures below is drawn to scales.
Question 1
In the figure below, \( A\), \( B \) and \( D \) are points on the circle and \( AB \) passes through the center \( O \) of the circle whose radius \( r = 10 \).
1) Find all angles and sides of triangle \( ADB \).
2) Find the size of angle \( \angle AOD \).

Solution
1)
According to the semicircle theorem above, \( \angle ADB = 90^{\circ} \) and therefore \( \angle DBA = 90^{\circ} - 63^{\circ} = 27^{\circ} \)
\( AB = 2 r = 20 \) ,
\( ADB \) is a right triangle and therefore \( \quad \cos 63^{\circ} = \dfrac{AD}{AB} = \dfrac{AD}{20} \) and \( \quad \sin 63^{\circ} = \dfrac{DB}{AB} = \dfrac{DB}{20} \)
The above gives: \( \quad AD = 20 \cos 63^{\circ} \) and \( \quad DB = 20 \sin 63^{\circ} \)
2)
\( \angle AOD \) is a central angle intercepting the same arc as the inscribed angle \( \angle DBA \), hence \( \angle AOD = 2 \angle DBA = 54^{\circ}\)
Question 2
In the figure below, \( A\), \( B \), \( C \) and \( D \) are points on a circle and \( AC \) is its diameter.
1) Find \( x \) given the lengths of segments \( \quad DA = x-4 , DC = x+2, BA = x-2 \) and \( BC = x + 1 \).
2) Find the radius of the circle.
2) Find the area of the quadrilateral \( ABCD\).
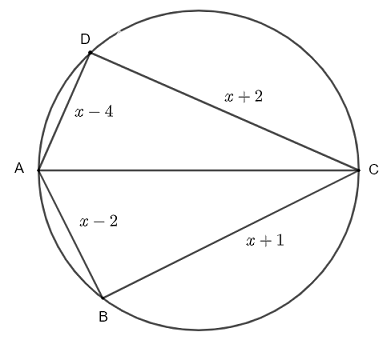
Solution
1)
According to the semicircle theorem above, \( CAB \) and \( CAD \) are right triangles with a common hypotenuse. Use the Pythagorean theorem to write:
\( (x-4)^2 + (x+2)^2 = AC^2\) (I)
and \( (x-2)^2 + (x+1)^2 = AC^2\) (II)
Combining (I) and (II) above, we obtain the equation: \( \quad (x-4)^2 + (x+2)^2 = (x-2)^2 + (x+1)^2 \)
Expand: \( 2x^2-4x+20 = 2x^2-2x+5 \)
Group like terms and simplify to rewrite the above equation as: \( \quad -2x+15 = 0 \)
Solve for \( x \): \( \quad x = \dfrac{15}{2} \)
2)
The radius \( r \) of the circle is equal to half the diameter \( AC \)
Substitute \( x \) by its value in (I) to write: \( \quad AC^2 = (\dfrac{15}{2}-4)^2 + (\dfrac{15}{2}+2)^2 = \dfrac{205}{2} \)
\( AC = \sqrt {\dfrac{205}{2}} \) , hence \( \quad r = \dfrac{1}{2} \sqrt {\dfrac{205}{2}} \)
3)
The area \( A_1 \) of the right triangle triangle \( CAB \) is given by: \( \quad A_1 = \dfrac{1}{2} \times BA \times BC = \dfrac{1}{2} (x-2)(x+1) \)
The area \( A_2 \) of the right triangle triangle \( CAD \) is given by: \( \quad A_2 = \dfrac{1}{2} \times DA \times DC = \dfrac{1}{2} (x-4)(x+2) \)
The area \( A \) of the quadrilateral \( ABCD\) is the sum of the areas \( A_1 \) and \( A_2 \) of triangles \( CAB \) and \( CAD \)
\( A = A_1 + A_2 = \dfrac{1}{2} ( (x-2)(x+1) + (x-4)(x+2) ) \)
Substitute \( x \) by its value found above: \( A = \dfrac{1}{2} ( (\dfrac{15}{2}-2)(\dfrac{15}{2}+1) + (\dfrac{15}{2}-4)(\dfrac{15}{2}+2) ) = 40 \) square units.
Question 3
In the figure below, \( A\), \( B \) and \( C \) are points on a semicircle whose diameter is \( AB = 50\). The sides \( CA \) and \( CB \) of triangle \( ABC \) are congruent.
1) Find the lengths of \( CA \) and \( CB \)
2) Find the area of the shaded (blue) surface.
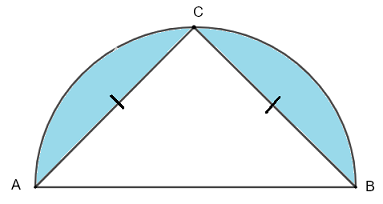
Solution
1)
According to the semicircle theorem above, \( ACB \) is a right triangle. Use the Pythagorean theorem to write: \( \quad CA^2 + CB^2 = AB^2 \)
Given that \( AB = 50\) and \( CA \) and \( CB \) are congruent, the above gives the equation: \( \quad 2 CA^2 = 50^2 \)
Solve for \( CA \) to obtain: \( \quad CA = \dfrac{50}{\sqrt 2} = CB \)
2)
Let \( A_s \) be the area of the semicircle, \( A_t \) the area of triangle \( ACB \) and \( r \) the radius of the semicircle.
\( A_s = \dfrac{1}{2} \pi r^2 = \dfrac{1}{2} \pi \left(\dfrac{AB}{2}\right)^2 = \dfrac{25^2}{2} \pi \)
\( A_t = \dfrac{1}{2} \times CA \times CB = \dfrac{1}{2} \times \dfrac{50^2}{ 2 } \)
The shaded area \( A \) is the area of the triangle \( A_t \) subtracted from the area of the semicircle \( A_s \).
\( A = \dfrac{25^2}{2} \pi - \dfrac{1}{2} \times \dfrac{50^2}{ 2 } = \dfrac{625\pi }{2}-625 \approx 356.75\) square units.
Question 4
\( C \) is a point on the circle whose diameter is \( AB = 100 \) and center \( O \).
1) Find angles \( \angle COB \) and \( \angle COA \)
2) Find the areas of the triangles \( COA \) and \( COB \).
3) Find the length of \( CA \) and \( CB \).
4) Find the area of triangle \( ACB \)
5) Compare the area of triangle \( ACB \) found in part 2) to the sum of the areas found in part 2).

Solution
1)
Point \( A \), \( C \) and \( B \) are on the circle of center \( O \) and therefore \( OA = OB = OC \). Hence \( AOC \) and \( COB \) are isosceles triangles and therefore
\( \angle OCB = \angle CBO = 62^{\circ} \) which gives \( \angle COB = 180 - 62 - 62 = 56^{\circ} \)
\( \angle COB \) and \( \angle COA \) make a straight angle; hence \( \angle COA = 180 - \angle COB = 124^{\circ}\)
2)
Use the sine rule formula to find the area \( A_1 \) of triangle \( COA \): \( \quad A_1 = \dfrac{1}{2} \sin \angle COA \times OC \times OA
\\
= \dfrac{1}{2} \sin 124^{\circ} \times 50 \times 50 = 1250 \sin 124^{\circ} \approx = 1036.30\) square units
In a similar way, the area \( A_2 \) of triangle \( COB \) is given by: \( \quad A_2 = \dfrac{1}{2} \sin \angle COB \times OC \times OB
\\
= \dfrac{1}{2} \sin 56^{\circ} \times 50 \times 50 = 1250 \sin 56^{\circ} \approx 1036.30 \) square units
3)
According to the semicircle theorem , \( ACB \) is a right traingle with \( AB \) as the hypotenuse. Hence \( \sin 62^{\circ} = \dfrac{CA}{AB} \) and \( \cos 62^{\circ} = \dfrac{CB}{AB} \)
The above gives \( CA = 100 \sin 62^{\circ} \) and \( CB = 100 \cos 62^{\circ} \)
4)
The area \( A \) of the right triangle \( ACB \) is given by: \( A = \dfrac{1}{2} \times CA \times CB = \dfrac{1}{2} 100^2 \sin 62^{\circ} \cos 62^{\circ} \approx 2072.59\) square units
5)
The sum of the ares in part 2) is: \( \quad A_1 + A_2 = 1036.30 + 1036.30 \approx 2072.60 \) is very close to \( A \approx 2072.59\). The small difference is due the rounding errors in the calculations.
In fact the sum of the areas \( COA \) and \( COB \) is equal to the area of triangle \( ACB \).