Simplify Radicals
Questions with Solutions for Grade 10

Grade 10 questions on how to simplify radicals expressions with solutions are presented.

In order to simplify radical expressions, you need to be aware of the following rules and properties of radicals

1) From definition of n th root(s) and principal root
equation 1
Examples

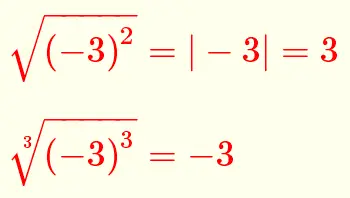
equation 3
More examples on Roots of Real Numbers and Radicals .
2) Product (Multiplication) formula of radicals with equal indices is given by
equation 4

More examples on how to Multiply Radical Expressions .

3) Quotient (Division) formula of radicals with equal indices is given by
equation 5

More examples on how to Divide Radical Expressions .

4) You may add or subtract like radicals only
Example
equation 6
More examples on how to Add Radical Expressions .

5) You may rewrite expressions without radicals (to rationalize denominators) as follows
A) Example 1:
equation 7
B) Example 2:
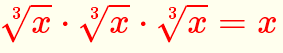
equation 9
C) Example 3:
equation 9
More examples on how to Rationalize Denominators of Radical Expressions .

Examples
Rationalize and simplify the given expressions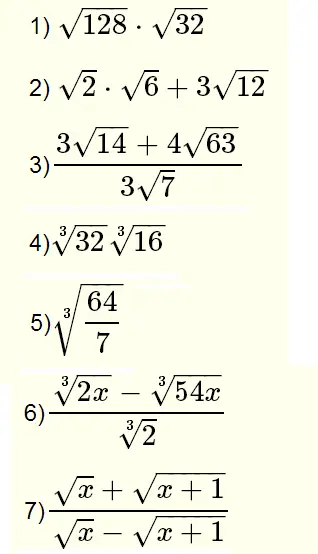
equation 10

Answers to the above examples

1)
Write 128 and 32 as product/powers of prime factors: 128 = 2 7 , 32 = 2 5 hence
equation 11

2)
Use product rule to write that √2 √6 = √12
equation 12

3)
Write 14 and 63 as products of prime numbers 14 = 2 × 7 , 63 = 3 2 × 7 and substitute
equation 13

4)
Write 32 and 16 as products of prime numbers 32 = 2 5 , 16 = 2 4 and substitute

equation 14

5)
Write 64 as products of prime numbers 64 = 2 6 and substitute
equation 15
Rationalize the denominator by multiplying numerator and denominator by ( 3 √7) 2
equation 16

6)
Write 54 as products of prime numbers 54 = 2 × 3 3 and substitute

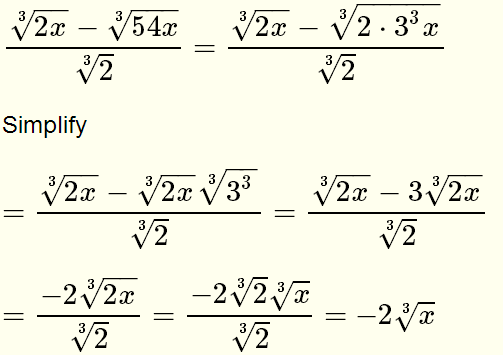
equation 17

7)
Multiply the denominator and numerator by the conjugate of the denominator
equation 18
Expand and simplify
equation 19
More Questions With Answers
Use all the rules and properties of radicals to rationalize and simplify the following expressions.
equation 20

Solutions to the Above Questions

  1. Write 25 and 125 as the product of prime factors: 25 = 52 and 125 = 53, hence
    solution 1
  2. Write 64 and 16 as the product of prime factors: 64 = 26 and 16 = 24, hence
    solution 2
  3. Use product rule
    solution 3
  4. Convert the mixed number under the radical into a fraction and substitute
    solution 42
    Use the division formula for radicals
    solution 43
    Write 64 and 27 as product of prime factors, substitute and simplify
    solution 44
  5. Use the product formula and write 34 as the product of prime factors
    solution 51
    Simplify
    solution 52
    For √(17 x) and √(34 x) to be real numbers, x must be positive hence |x| = x
    solution 51
  6. Write the radicand as a square and simplify
    solution 6
  7. Write the radicand as the product of $2$ and a square and simplify
    solution 7
  8. Simplify the radicand
    solution 81
    Write as the product of prime factors and simplify
    solution 82
  9. Since n is a positive integer, then N = 2 n + 1 is an odd integer. Hence
    solution 9
  10. Since n is a positive integer, then N = 2 n is an even integer. Hence
    solution 10
  11. solution 11
  12. Use division rule and simplify the radicand
    solution 12
  13. Multiply numerator and denominator by the conjugate of the denominator
    solution 131
    Expand and simplify
    solution 132

More High School Math (Grades 10, 11 and 12) - Free Questions and Problems With Answers
More Middle School Math (Grades 6, 7, 8, 9) - Free Questions and Problems With Answers
More Primary Math (Grades 4 and 5) with Free Questions and Problems With Answers
Author -
Home Page