Algebra Questions with Answers and Solutions - Grade 12
Grade 12
algebra questions with answers and solutions are presented. Some of these questions may be challenging; you need to spend time on them as these are the ones that make you think and learn how to solve problems. Also group work on challenging questions is an excellent opportunity to interact with others and learn from them. Let me know of any other possible solutions to any of the questions below.
Free Practice for SAT, ACT
and Compass Maths tests
-
Order from greatest to least
a) 25100
b) 2300
c) 3400
d) 4200
e) 2600
-
Find all rational zeros of P(x) = x3 - 7x + 6.
-
Round all real zeros in the graph to the nearest integer and find a polynomial function P of lowest degree, with the absolute value of the leading coefficient equal to 1, that has the indicated graph.
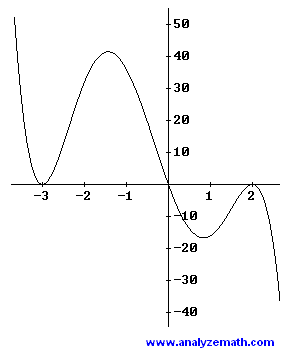 .
.
-
2 - i, where i is the imaginary unit, is a zero of P(x) = x4 - 4x3 + 3x2 + 8x - 10. Find all zeros of P.
-
Find a, b and c so that the graph of the quadratic function f(x) = ax2 + bx + c has a vertex at (-2 , 1) and passes through the point (0 , -3).
-
f(x) is a quadratic function such that f(1) = 3 and f(5) = 3. Find the x coordinate of the vertex of the graph of f.
-
Find a and b so that the rational function f(x) = (ax4 + bx3 + 3) / (x3 - 2) has an oblique asymptote given by y = 2x - 3
-
Solve for x the equation log9 (x3) = log2(8)
-
Find the value of logy (x4) if logx (y3) = 2
-
Solve for x the equation logx (8e3) = 3
-
If 16x + 16x - 1 = 10, find 22x.
-
If a2 - b2 = 8 and a×b = 2, find a4 + b4.
-
What are the maximum value and minimum values of f(x) = |2sin(2x - ?/3) - 5| + 3
-
If x < -7, simplify |4 - |3 + x||
-
A car travels from A to B at an average speed of 50 km/hour. At what average speed would it have to travel from B to A to average 60 km/hour for the whole trip?
-
If x2 - y2 = -12 and x + y = 6, find x and y.
-
f(x) is a function such that f(x) + 3 f(8 - x) = x for all real numbers x. Find the value of f(2).
-
f(x) is a function such that f(2x + 1) = 2 f(x) + 1 for all real numbers x and f(0) = 2. Find the value of f(3).
-
Find b so that the line y = 2 x + b is tangent to the circle x2 + y2 = 4.
-
What is the remainder of the division (x100 - x99 - x + 1) / (x2 - 3x + 2)
-
Evaluate the number represented by the infinite series √(1/3 + √(1/3 + √(1/3 + ...))).
-
Show that the 3 by 3 system of equations given below has no solutions.
2 x + y - 3z = 5
-5 x + 3 y + 2 z = 7
3 x - 4 y + z = 8
Solutions to the Above Problems
-
25100
2300 = (23)100 = 8100
3400 = (34)100 = 81100
4200 = (42)100 = 16100
2600 = (26)100 = 64100
from greatest to least: 3400 , 2600, 25100 , 4200 , 2300
-
P(x) = x3 - 7x + 6 : given
leading coefficient 1 and its factors are : +1,-1
constant term is 6 and its factors are : +1,-1,+2,-2,+3,-3,+6,-6
possible rational zeros : +1,-1,+2,-2,+3,-3,+6,-6
test : P(1) = 0, P(2) = 0 and P(-3) = 0
x = 1, x = 2 and x = -3 are the zeros of P(x).
From the graph, x = -3 is a zero of multiplicity 2, x = 0 is a zero of multiplicity 1 and x = 2 is a zero of multiplicity 2.
P(x) = -x (x + 3)2(x - 2)2 : polynomial with real zeros hence with lowest degree.
- )
if 2 - i is a zero and the coefficients of the polynomial are real then 2 + i (the conjugate) is also a solution.
P(x) = (x - (2 - i))(x - (2 + i))×q(x) = ((x - 2)2 + 1)×q(x)
q(x) = P(x)/((x - 2)2 + 1) = (x2 - 2)
x = 2 - i , x = 2 + i , x = √2 and x = - √2 are the 4 zeros of P(x).
-
f(x) = a (x + 2)2 + 1 : equation of parabola in vertex form
f(0) = -3 = 4 a + 1
a = -1 : solve for a
f(x) = -(x + 2)2 + 1 = -x2 - 4x - 3
a = -1 , b = -4 and c = -3 : identify coefficients
-
f(x) = a x2 + b x + c
f(1) = 3 which give 3 = a + b + c
f(5) = 3 which gives 3 = 25a + 5b + c
24 a + 4 b = 0 : subtract equation B from equation C
x coordinate of vertex = -b/2a = 3 : from above equation
-
The oblique asymptote is the quotient resulting from the long division of ax4 + bx3 + 3 by x3 - 2
The quotient obtained is ax + b
a x + b = 2 x - 3
a = 2 and b = -3 : for two polynomials to be equal, the corresponding coefficients has to be equal.
-
log9 (x3) = log2(8) : given
log2 (23) = 3 : simplify right hand side of given equation.
log9 (x3) = 3 : rewrite the above equation
log9 (x3) = log9(93) : rewite 3 as a log base 9.
x3 = 93 : obtain algebraic equation from eqaution D.
x = 9 : solve above for x.
-
logx (y3) = 2 : given
x2 = y3 : rewrite in exponential form
x4 = y6 : square both sides
x4 = y6 : rewrite the above using the log base y
logy(x4) = logy(y6) = 6
-
logx (8 e3) = 3 : given
x3 = 8 e3 = (2e)3
x = 2 e
-
16x + 16x - 1 = 10 : given
42x + 42x / 16 = 10
42x = 160/17 : solve for 42x
4x = 4 √(10) / √(17) : extract the square root
22x = 4x = 4 √(10) / √(17)
-
a2 - b2 = 8 : given
a4 + b4 - 2a2b2 = 82 : square both sides and expand.
a×b = 2 : given
a2b2 = 22 : square both sides.
a4 + b4 - 2(4) = 82 : substitute
a4 + b4 = 72
-
-1 ? sin(2x - ?/3) ? 1 : range of a sine function
-2 ? 2sin(2x - ?/3) ? 2 : multiply all terms of the double inequality by 2
-2 - 5 ? 2sin(2x - ?/3) - 5 ? 2 - 5 : add -5 to all terms of the inequality.
-7 ? 2sin(2x - ?/3) - 5 ? -3
3 ? |2sin(2x - ?/3) - 5| ? 7 : change the above using absolute value.
3 + 3 ? |2sin(2x - ?/3) - 5| + 3 ? 7 + 3 : add 3 to all terms of the double inequality.
The maximum value of f(x) is equal to 10 and the minimum value of f(x) is equal to 6.
-
If x < -7 then x < - 3 and x + 3 < 0 and |3 + x| = -(3 + x)
|4 - |3 + x|| = |4 + 3 + x| = |x + 7| = - (x + 7) = - x - 7 : since x + 7 < 0
-
Let d be the distance between A and B
T1 = d / 50 : travel time from A to B
Let S be the speed from B to A
T2 = d/S : travel time from B to A
60 = 2d/(T1 + T2) : average speed for the whole trip
60 = 2d/(d/50 + d/S) : substitute T1 and T2
S = 75 km/hour : solve the above equation for S.
-
x2 - y2 = (x - y)(x + y) = -12 : given
6(x - y) = -12 : substitute x + y by 6
(x - y) = -2 : solve for x - y
(x - y) = -2 and x + y = 6 : 2 by 2 system.
x = 2 , y = 4 : solve above system.
-
f(x) + 3f(8 - x) = x : given
f(2) + 3f(6) = 2 : x = 2 above
f(6) + 3f(2) = 6 : x = 6 above
f(6) = 6 - 3f(2) : solve equation C for f(6)
f(2) + 3(6 - 3f(2)) = 2 : substitute
f(2) = 2 : solve above equation.
-
f(2x + 1) = 2f(x) + 1 : given
f(3) = 2f(1) + 1 : x = 1 in A
f(1) = 2f(0) + 1 : x = 0 in A
f(3) = 11 : substitute
-
x2 + y2 = 4 : given
x2 + (2x + b)2 = 4 : substitute y by 2x + b
5x2 + 4bx + b2 - 4 = 0
The number of points of intersection is given by the number of solutions of the above equation. The line and circle are tangent if the above quadratic equation has only one solution which means that the discriminant is equal to zero. Find the discriminant as a function of b and solve.
b = √2 and b = -√2 : 2 solutions.
(x100 - x99 - x + 1) / (x2 - 3x + 2)
Let P(x) = x100 - x99 - x + 1 , D(x) = x2 - 3x + 2
The division of the two polynomials may be written as
P(x) = D(x) Q(x) + r(x) , where Q(x) is the quotient and r(x) is the remainder that will have a degree equal to one or lower. r(x) = a x + b
We now need to find a and b that define the remainder.
Note that D(x) may be factored as follows: D(x) = x2 - 3x + 2 = (x - 1)(x - 2)
Hence: P(x) = (x - 1)(x - 2) Q(x) + a x + b
Using the zeros of D(x) to write:
P(1) = (1 - 1)(1 - 2) Q(1) + a (1) + b gives a + b = P(1)
P(2) = (2 - 1)(2 - 2) Q(2) + a (2) + b gives 2 a + b = P(2)
We now need to evaluate P(1) and P(2)
P(1) = 1100 - 199 - (1) + 1 = 0
First rewrite P(x) = x99(x - 1) - x + 1 ; Hence P(2) = 299(2 - 1) - 2 + 1 = 299 - 1
We now have a system of equations to solve and find a and b.
a + b = 0 and 2 a + b = 299 - 1
a = 299 - 1 and b = 1 - 299
remainder: r(x) = (299 - 1) x + 1 - 299
Let y = √(1/3 + √(1/3 + √(1/3 + ...))).
square both sides to obtain: y 2 = 1/3 + √(1/3 + √(1/3 + √(1/3 + ...)))
We can write: y 2 = 1/3 + y
Solve the above quadratic equation to obtain: y = (3 + √(21)) / 6 and y = (3 - √21) / 6
y is positive hence the solution: √(1/3 + √(1/3 + √(1/3 + ...))) = y = (3 + √(21)) / 6
More References and links
High School Math (Grades 10, 11 and 12) - Free Questions and Problems With Answers
Middle School Math (Grades 6, 7, 8, 9) - Free Questions and Problems With Answers
Primary Math (Grades 4 and 5) with Free Questions and Problems With Answers
Home Page
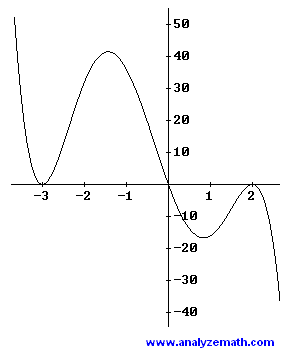 .
.
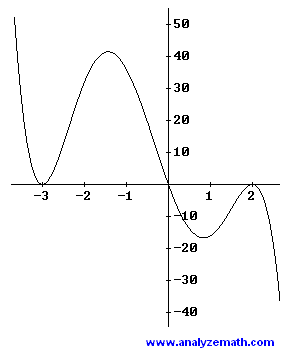 .
.