Below is shown the graph of f(x) = 2 x 3 - 1
 .
.
1) Sketch the graph of the inverse of f in the same system of axes.
2) Find the inverse of and check your answer using some points.
Solution
1) Locate few points on the graph of f. Here is a list of points whose coordinates (a , b) can easily be determined from the graph:
(1 , 1) , (0 , -1) , (-1 , -3)
On the graph of the inverse function, the above points will have coordinates (b , a) as follows:
(1 , 1) , (-1 , 0) , (-3 , -1)
Plot the above points and sketch the graph of the inverse of f so that the two graphs are reflection of each other on the line y = x as shown below.
 .
.
2) Write the given function f(x) = 2 x 3 - 1 as an equation in two unknowns.
y = 2 x 3 - 1
Solve the above for x.
2 x 3 = y + 1
x 3 = (y + 1) / 2

Interchange x and y and write the equation of inverse function f -1:
 .
.
We now verify that the points (1 , 1) , (-1 , 0) and (-3 , -1) used above to sketch the graph of the inverse function are on the graph of f -1.
 .
.
Let f(x) = x 2 - 4 x + 5, x ? 2.
1) Find the inverse function of f.
2) Find the domain and the range of f -1.
Solution
1) We are given a quadratic function with a restricted domain. We first write the given function in vertex form (may be done by completing the square):
f(x) = x 2 - 4 x + 5 = (x - 2) 2 + 1 , x ? 2
The graph of function f is that of the left half of a parabola with vertex at (2 , 1) as shown below.
 .
.
We now write the given function as an equation.
y =(x - 2) 2 + 1
Solve the above for x.
y =(x - 2) 2 + 1
(x - 2) 2 = y - 1
Two solutions for x - 2: x - 2 = +√(y - 1) or x - 2 = - √(y - 1)
x = √(y - 1) + 2 or x = - √(y - 1) + 2
Since x ? 2 (domain of f), we select the solution
x = - √(y - 1) + 2
Interchange x and y to write the inverse of function f as follows.
y = f -1(x) = - √(x - 1) + 2
The domain and range of f -1 are the range and domain of f.
Domain of f -1 is the range of f: [1 , +?) (from graph)
Range of f -1 is the domain of f: (-? , 2] (given)
Below is shown the graph of f(x) = √(2 x - 3).
 .
.
1) Sketch the inverse of f in the same graph.
2) Find the inverse of and check your answer using some points.
Solution
1) Locate few points on the graph of f. A possible list of points whose coordinates (a , b) is as follows:
(1.5 , 0) , (2 , 1) , (6 , 3)
On the graph of the inverse function, the above points will have coordinates (b , a) as follows:
(0 , 1.5) , (1 , 2) , (3 , 6)
Plot the above points and sketch the graph of the inverse of f so that the two graphs are reflection of each other on the line y = x as shown below.
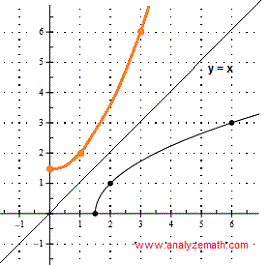 .
.
2) Write the given function f(x) = √(2 x - 3) as an equation in two unknowns.
y = √(2 x - 3)
Solve the above for x. First square both sides
2 x - 3 = y 2
2 x = y 2 + 3
x = (y 2 + 3) / 2
Interchange x and y and write the equation of the inverse function f -1; and write the domain of the inverse.
y = (x 2 + 3) / 2
f -1 (x) = (x 2 + 3) / 2 , x ? 0 (domain which is the range of f from its graph above)
We now verify that the points (0 , 1.5) , (1 , 2) and (3 , 6) used to sketch the graph of the inverse function are on the graph of f -1.
f -1(0) = (0 2 + 3) / 2 = 1.5
f -1(1) = (1 2 + 3) / 2 = 2
f -1(3) = (3 2 + 3) / 2 = 6
Sketch the graph of f -1 using the graph of y = f(x) shown below and find f -1(x).
 .
.
Solution
1) Use the graph to find points on the graph of f. A possible list of points whose coordinates (a , b) is as follows:
(0 , 3) , (2 , -1) , (5 , - 3)
On the graph of the inverse function, the above points will have coordinates (b , a) as follows:
(3 , 0) , (- 1 , 2) , (- 3 , 5)
Plot the above points and sketch the graph of the inverse of f so that the two graphs are reflection of each other on the line y = x as shown below.
 .
.
2) We now determine f -1(x). For -3 ? x ? - 1 , f -1(x) has a linear expression with slope m1 through the points (- 1 , 2) , (- 3 , 5) given by
m1 = (5 - 2) / (-3 - (-1)) = - 3 / 2
For -3 ? x ? - 1, f -1(x) is given by:
f -1(x) = - (3 / 2)(x - (-1)) + 2 = - (3 / 2)(x + 1) + 2
For - 1 < x ? 3 , f -1(x) has a linear expression with slope through the points (- 1 , 2) , (3 , 0) given by
m2 = (0 - 2) / (3 - (-1)) = - 1 / 2
For - 1 < x ? 3, f -1(x) is given by:
f -1(x) = - (1 / 2)(x - (-1)) + 2 = - (1 / 2)(x + 1) + 2
The one to one function


1) What is the domain and range of f?
2) Sketch the graph of f -1.
3) Find f -1(x) (include domain).
Solution
1) f(x) is defined as a real number if the radicand 2 / x - 1 is greater than or equal to 0. Hence we need to solve the inequality:
2 / x - 1 ? 0
(2 - x) / x ? 0
The expression on the left of the inequality changes sign at the zeros of the numerator and denominator which are x = 2 and x = 0. See table below.
 .
.
Domain: (0 , 2]
Range: (-? , 0]
2) Points on the graph of f
(2 , 0) , (1 , -1)
The above points on the graph of the inverse function, will have coordinates (b , a) as follows:
(0 , 2) , (- 1 , 1)
Plot the above points and sketch the graph of the inverse of f so that the two graphs are reflection of each other on the line y = x as shown below.
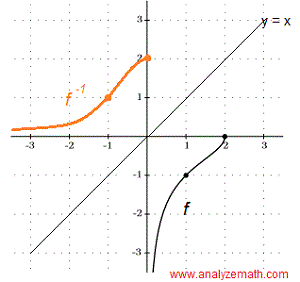 .
.
3) Write f(x) as an equation in y and x.
\( y = -\sqrt{\dfrac{2}{x}-1} \)
Solve the above equation for x. Square both sides of the above equation
\( y^2 = \dfrac{2}{x}-1 \)
\( \dfrac{2}{x} = y^2 + 1 \)
\( x = \dfrac{2}{y^2 + 1} \)
Interchange x and y and write the inverse function
\( y = \dfrac{2}{x^2 + 1} \)
\( f^{-1}(x) = \dfrac{2}{x^2 + 1} \)
Domain and range of f-1 are the range and domain of f . Hence
Domain of f -1: (-? , 0]
Range of f -1: (0 , 2]
Below are shown the graph of 6 functions. Sketch the graph of the inverse of each function.
 .
.
Solution
For each graph, select points whose coordinates are easy to determine. Use these points and also the reflection of the graph of function f and its inverse on the line y = x to skectch to sketch the inverse functions as shown below
 .
.
Find the inverse of f(x) = Log4(x + 2) - 5, its domain and range.
Solution
Write the given function as an equation in x and y as follows:
y = Log4(x + 2) - 5
Solve the above equation for x.
Log4(x + 2) = y + 5
x + 2 = 4 (y + 5)
x = 4 (y + 5) - 2
Interchange x and y.
y = 4 (x + 5) - 2
Write the inverse function with its domain and range.
f-1(x) = 4 (x + 5) - 2 , Domain: (-? , +?) , Range: (-2 , +?)
If f(x) = ln(x) + 4 x - 8, what is the value of f -1(- 4)?
Solution
Let a = f -1(- 4). Then
f(a) = f(f -1(- 4)) = - 4 (Using the property f(f -1(x)) = x of the inverse function).
We now need to find a such that f(a) = - 4 hence the equation to solve.
ln(a) + 4 a - 8 = - 4
ln(a) = 4 - 4 a
The above equation cannot be solved analytically but its solution may be approximated graphically as the x coordinate of the point of intersection of the graphs of y = ln(x) and y = 4 - 4x as shown below.
 .
.
The intersection of the two graphs is close to x = 1 which can easily be checked that it is the exact solution to the equation ln(x) = 4 - 4 x. Hence
f-1( - 4) = 1