
The latitude and longitude coordinate system, used in GPS systems, is presented with examples.
An online calculator to calculate the >distance between two Points on earth is also included.
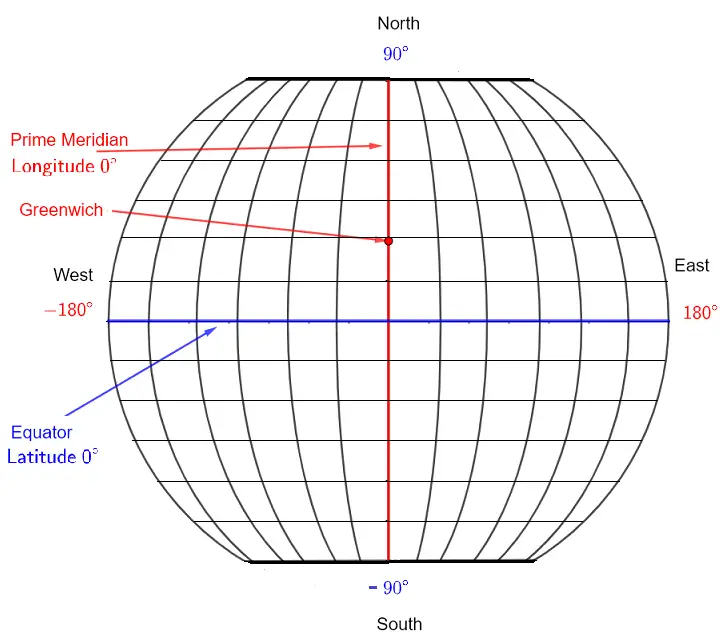
It is internationally agreed that the prime meridian is an imaginary great circle that divides the earth, supposed to be a sphere, into two hemisphere
(half the sphere Earth): the eastern and western parts as shown below in figures 1 and 2 below. The prime meridian circle runs through Greenwich in England.
The equator is also an imaginary circle that is equidistant from the North and South poles and divides the earth, supposed to be a sphere, into two hemisphere: the northen and southern parts as shown below in figures 1 and 2 below.


The latitude of the Equator is equal to zero. The longitude of the prime meridian (through Greenwich) is equal to zero.
The longitude takes values from \( 0^{\circ} \) to \( 180^{\circ} \) East and \( 180^{\circ} \) West.
Negative and positive signs may also be used to express the longitude such that longitude to the east is positive and a longitude to the west is negative
The range of longitudes may be written as: \( [-180^{\circ} , 180^{\circ} ] \)
The latitude takes values from \( 0^{\circ} \) to \( 90^{\circ} \) North and \( 90^{\circ} \) South.
Negative and positive signs may also be used to express the latitude such that latitude to the North is positive and a latitude to the South is negative
The range of latitudes may be written as: \( [-90^{\circ} , 90^{\circ} ] \)
We give some examples of latitude and longitude using either the negative/positive signs and South/North (S/N) for latitude and east/west (E/W) for longitude.
The latitude and the longitude are separated by a comma.
Washington DC(latitude and longitude): 38.8938672 , -77.0846157 or 38.8938672 N , 77.0846157 W
Algiers(latitude and longitude): 36.7391355 , 3.0692912 or 36.7391355 N , 3.0692912 E
London UK(latitude and longitude): 51.5287718 , -0.2416802 or 51.5287718 N , 0.2416802 W
Moscow (latitude and longitude): 55.5815245 , 36.8251409 or 55.5815245 N , 36.8251409 E
Tokyo (latitude and longitude): 35.5090627 , 139.2093892 or 35.5090627 N , 139.2093892 E
Beijing (latitude and longitude): 39.9390731 , 116.1172792 or 39.9390731 N , 116.1172792 E
Cape Town (latitude and longitude): -33.914651 , 18.3758812 or 33.914651 S , 18.3758812 E
Kuala Lumpur (latitude and longitude): 3.138675 , 101.6169495 or 3.138675 N , 101.6169495 E
Kampala latitude and longitude: 0.3132008 , 32.5290854 or 0.3132008 N , 32.5290854 E
Being angles, the latitude and longitude may be written into one of the following forms.
1 - Decimal degrees (DD) format
Example 3
3.138675 \(^{\circ}\) , 101.6169495 \(^{\circ}\) or 3.138675 \(^{\circ}\) N , 101.6169495 \(^{\circ}\) E
2 - Degrees, minutes and seconds (DMS)
Example 4
3\(^{\circ}\) 34' 45.3'' , 23\(^{\circ}\) 55' 23.7'' or 3\(^{\circ}\) 34' 45.3'' N , 23\(^{\circ}\) 55' 23.7'' E
3 - Degrees and decimal minutes (DDM)
Example 5
-32\(^{\circ}\) 34.67' , - 43\(^{\circ}\) 55.67' or 32\(^{\circ}\) 34.67' S , 43\(^{\circ}\) 55.67' W
Knowing the rates of conversion
1 degree = 60 minutes or 1 minute = (1/60) degree
1 minute = 60 seconds or 1 second = (1/60) minute = (1/3600) degree
conversion from DD to DMS to DDM and vice versa can be done using simple calculations.
Example 6
a) Convert 3.138675 \(^{\circ}\) to DDM and DMS
b) Convert 45 \(^{\circ}\) 55' 23.7'' to DD and DDM
Solution
a) 3.138675 \(^{\circ}\) = 3 \(^{\circ}\) + 0.138675 × 60 minutes = 3 \(^{\circ}\) 8.3205' DDM
= 3 \(^{\circ}\) + 8' + 0.3205 × 60 seconds = 3 \(^{\circ}\) + 8' + 19.23 '' = 3 \(^{\circ}\) 8' 19.23 '' DMS
b)
From the rate given above, we can write that 1' = (1/60)\(^{\circ}\) \) and 1'' = (1/3600)\(^{\circ}\) \)
Hence
45 \(^{\circ}\) 55' 23.7'' = 45\(^{\circ}\) + 55' + 23.7'' = 45\(^{\circ}\) + 55 (1/60)\(^{\circ}\) + 23.7 (1/3600)\(^{\circ}\) = 45.92325 \(^{\circ}\) DD
45.92325 \(^{\circ}\) = 45\(^{\circ}\) + 0.92325 \(^{\circ}\) = 45\(^{\circ}\) + 0.92325 × 60' = 45\(^{\circ}\) 55.395' DDM