Matrices with Examples and Questions with Solutions

Examples and questions on matrices along with their solutions are presented .

Page Content

Definition of a Matrix
Matrix entry (or element)
Square Matrix
Identity Matrix
Diagonal Matrix
Triangular Matrix
Transpose of a Matrix
Symmetric Matrix
Questions on Matrices: Part A
Questions on Matrices: Part B
Solutions to the Questions in Part A
Solutions to the Questions in Part B
References

Definition of a Matrix

The following are examples of matrices (plural of matrix).

An m × n (read 'm by n') matrix is an arrangement of numbers (or algebraic expressions ) in m rows and n columns. Each number in a given matrix is called an element or entry.
A zero matrix has all its elements equal to zero.

Example 1
The following matrix has 3 rows and 6 columns.

The order (or dimensions or size) of a matrix indicates the number of rows and the number of columns of the matrix. In this example, the order of the matrix is 3 × 6 (read '3 by 6').

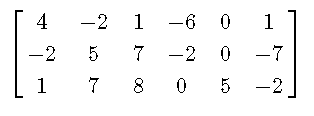
Matrix entry (or element)

The entry (or element) in a row i and column j of a matrix A (capital letter A) is denoted by the symbol $(A)_{i j}$ or $a_{i j}$ (small letter a).

Example 2

In the matrix A shown below, $a_{11} = 5$ , $a_{12} = 2$ , etc ... or $(A)_{11} = 5$ , $(A)_{12} = 2$ , etc ... $A = [\begin{matrix} 5 & 2 & 7 & - 3 \\ - 9 & - 2 & - 7 & 11 \end{matrix}] = [\begin{matrix} a_{11} & a_{12} & a_{13} & a_{14} \\ a_{21} & a_{22} & a_{23} & a_{24} \end{matrix}]$

Square Matrix

A square matrix has the number of rows equal to the number of columns.

Example 3

For each matrix below, determine the order and state whether it is a square matrix.
$a) [\begin{matrix} - 1 & 1 & 0 & 3 \\ 4 & - 3 & - 7 & - 9 \end{matrix}] b) [\begin{matrix} - 6 & 2 & 0 \\ 3 & - 3 & 4 \\ - 5 & - 11 & 9 \end{matrix}] c) [\begin{matrix} 1 & - 2 & 5 & - 2 \end{matrix}] d) [\begin{matrix} - 2 & 0 \\ 0 & - 3 \end{matrix}] e) [\begin{matrix} 3 \end{matrix}]$
Solutions
a) order: 2 × 4. Number of rows and columns are not equal therefore not a square matrix.
b) order: 3 × 3. Number of rows and columns are equal therefore this matrix is a square matrix.
c) order: 1 × 4. Number of rows and columns are not equal therefore not a square matrix. A matrix with one row is called a row matrix (or a row vector).
d) order: 2 × 2. Number of rows and columns are equal therefore this is square matrix.
e) order: 1 × 1. Number of rows and columns are equal therefore this matrix is a square matrix.

Identity Matrix

An identity matrix I_n is an n×n square matrix with all its element in the diagonal equal to 1 and all other elements equal to zero.
Example 4
The following are all identity matrices. $I_{1} = [\begin{matrix} 1 \end{matrix}], I_{2} = [\begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix}], I_{3} = [\begin{matrix} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{matrix}]$

Diagonal Matrix

A diagonal matrix is a square matrix with all its elements (entries) equal to zero except the elements in the main diagonal from top left to bottom right. $A = [\begin{matrix} 6 & 0 & 0 \\ 0 & - 2 & 0 \\ 0 & 0 & 2 \end{matrix}]$

Triangular Matrix

An upper triangular matrix is a square matrix with all its elements below the main diagonal equal to zero. Matrix U shown below is an example of an upper triangular matrix. A lower triangular matrix is a square matrix with all its elements above the main diagonal equal to zero. Matrix L shown below is an example of a lower triangular matrix.
$U = [\begin{matrix} 6 & 2 & - 5 \\ 0 & - 2 & 7 \\ 0 & 0 & 2 \end{matrix}] L = [\begin{matrix} 6 & 0 & 0 \\ - 2 & - 2 & 0 \\ 10 & 9 & 2 \end{matrix}]$

Transpose of a Matrix

The transpose of an m×n matrix $A$ is denoted $A^{T}$ with order n×m and defined by $(A^{T})_{i j} = (A)_{j i}$ Matrix $A^{T}$ is obtained by transposing (exchanging) the rows and columns of matrix $A$ .
Example 5
${[\begin{matrix} 6 & 0 \\ - 2 & - 2 \\ 10 & 9 \end{matrix}]}^{T} = [\begin{matrix} 6 & - 2 & 10 \\ 0 & - 2 & 9 \end{matrix}]$ Transpose a matrix an even number of times and you get the original matrix: $((A)^{T})^{T} = A$ . Transpose matrix an odd number of times and you get the transpose matrix: $(((A)^{T})^{T})^{T} = A^{T}$ .
The transpose of any square diagonal matrix is the matrix itself. ${[\begin{matrix} 3 & 0 & 0 \\ 0 & - 2 & 0 \\ 0 & 0 & 6 \end{matrix}]}^{T} = [\begin{matrix} 3 & 0 & 0 \\ 0 & - 2 & 0 \\ 0 & 0 & 6 \end{matrix}]$

Symmetric Matrix

A square matrix is symmetric if its elements are such that $A_{i j} = A_{j i}$ in other words $A$ is symmetric if $A = A^{T}$ .
Example 6
Symmetric matrices $[\begin{matrix} 4 & - 2 & 1 \\ - 2 & 5 & 7 \\ 1 & 7 & 8 \end{matrix}]$

Questions on Matrices: Part A

Given the matrices: $A = [\begin{matrix} - 1 & 23 & 10 \\ 0 & - 2 & - 11 \end{matrix}], B = [\begin{matrix} - 6 & 2 & 10 \\ 3 & - 3 & 4 \\ - 5 & - 11 & 9 \\ 1 & - 1 & 9 \end{matrix}], C = [\begin{matrix} - 3 & 2 & 9 & - 5 & 7 \end{matrix}] D = [\begin{matrix} - 2 & 6 \\ - 5 & 2 \end{matrix}], E = [\begin{matrix} 3 \end{matrix}], F = [\begin{matrix} 3 \\ 5 \\ - 11 \\ 7 \end{matrix}], G = [\begin{matrix} - 6 & - 4 & 23 \\ - 4 & - 3 & 4 \\ 23 & 4 & 9 \end{matrix}]$
a) What is the dimension of each matrix?
b) Which matrices are square?
c) Which matrices are symmetric?
d) Which matrix has the entry at row 3 and column 2 equal to -11?
e) Which matrices has the entry at row 1 and column 3 equal to 10?
f) Which are column matrices?
g) Which are row matrices?
h) Find $A^{T}, C^{T}, E^{T}, G^{T}$ .

Questions on Matrices: Part B

1) Given the matrices: $A = [\begin{matrix} 23 & 10 \\ 0 & - 11 \end{matrix}], B = [\begin{matrix} - 6 & 0 & 0 \\ - 1 & - 3 & 0 \\ - 5 & 3 & - 9 \end{matrix}], C = [\begin{matrix} - 3 & 0 \\ 0 & 2 \end{matrix}], D = [\begin{matrix} - 7 & 3 & 2 \\ 0 & 2 & 4 \\ 0 & 0 & 9 \end{matrix}], E = [\begin{matrix} 12 & 0 & 0 \\ 0 & 23 & 0 \\ 0 & 0 & - 19 \end{matrix}]$
a) Which of the above matrices are diagonal?
b) Which of the above matrices are lower triangular?
c) Which of the above matrices are upper triangular?

Solutions to the Questions in Part A

a) A: 2 × 3, B: 4 × 3, C: 1 × 5, D: 2 × 2, E: 1 × 1, F: 4 × 1, G: 3 × 3,
b) D, E and G
c) E and G
d) B
e) A and B
f) E and F
g) E and C
h) $A^{T} = [\begin{matrix} - 1 & 0 \\ 23 & - 2 \\ 10 & - 11 \end{matrix}], C^{T} = [\begin{matrix} - 3 \\ 2 \\ 9 \\ - 5 \\ 7 \end{matrix}], E^{T} = [\begin{matrix} 3 \end{matrix}], G^{T} = [\begin{matrix} - 6 & - 4 & 23 \\ - 4 & - 3 & 4 \\ 23 & 4 & 9 \end{matrix}]$

Solutions to the Questions in Part B

a) C and E
b) B
c) A and D