Orthogonal Vectors in \( R^n \) - Examples with Solutions

Orthogonal vectors are defined and examples are presented along with their detailed solutions.

Orthogonal Vectors Definition

Two vectors \( \textbf x = \begin{bmatrix} x_1 \\ x_2 \\ . \\ . \\ . \\ x_n \end{bmatrix} \) and \( \textbf y = \begin{bmatrix} y_1 \\ y_2 \\ . \\ . \\ . \\ y_n \end{bmatrix} \) are orthogonal if and only if their inner product \( \textbf x \cdot \textbf y \) is equal to zero.
Hence

Vectors \( \textbf x \) and \( \textbf y \) are orthogonal \( \iff \) \( \textbf x \cdot \textbf y = x_1 y_1 + x_2 y_2 + ... + x_n y_n = 0 \)

Geometric Interpretation of Orthogonal Vectors

Below are shown two 2-dimensional vetors \( \textbf x = \begin{bmatrix} 3 \\ 2 \end{bmatrix} \) and \( \textbf y = \begin{bmatrix} -2 \\ 3 \end{bmatrix} \) whose inner product is given by \( \textbf x \cdot \textbf y = (3)(-2)+(-2)(3) = 6 - 6 = 0 \) and therefore are orthogonal. They make and angle of \( 90^{\circ} \) or \( \dfrac{\pi}{2} \)


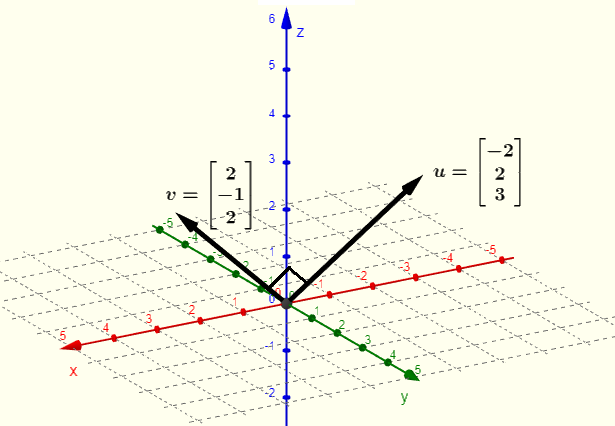
Below are shown two 3-dimensional vetors \( \textbf u = \begin{bmatrix} -2 \\ 2 \\ 3 \end{bmatrix} \) and \( \textbf v = \begin{bmatrix} 2\\ -1 \\ 2 \end{bmatrix} \) whose inner product is given by \( \textbf x \cdot \textbf y = (-2)(2)+(2)(-1) +(3)(2) = -4 - 2 + 6 = 0 \) and therefore are orthogonal. They make and angle of \( 90^{\circ} \) or \( \dfrac{\pi}{2} \)

Examples With Solutions

Example 1
a) Are the vectors \( \textbf x = \begin{bmatrix} -1 \\ 3 \\ 1 \end{bmatrix} \) and \( y = \begin{bmatrix} -2 \\ 3 \\ -11 \end{bmatrix} \) orthogonal?

b) Are the vectors \( \textbf u = \begin{bmatrix} 0 \\ -3 \\ 1 \\ 8 \end{bmatrix} \) and \( \textbf v = \begin{bmatrix} 3 \\ 5 \\ -2 \\ 2 \end{bmatrix} \) orthogonal?


Solution to Example 1
a)
The inner product of vactors \( \textbf x \) and \( \textbf y \) is given by
\( \textbf x \cdot \textbf y = = (-1)(-2)+(3)(3)+(1)(-11) = 2 +9 -11 = 0 \)
The inner product is equal to zero and therefore the two vectors \( \textbf x \) and \( \textbf y \) are orthogonal.
b)
The inner product of vactors \( \textbf u \) and \( \textbf v \) is given by
\( \textbf u \cdot \textbf v = = (0)(3) + (-3)(5) + (1)(-2) + (8)(2) = 0 -15 -2 + 16 = -1 \)
The inner product is NOT equal to zero and therefore the two vectors \( \textbf x \) and \( \textbf y \) are NOT orthogonal.

Example 2
Let vectors \( \textbf u = \begin{bmatrix} 1\\ 0 \\ -1 \end{bmatrix} \) , \( \textbf v = \begin{bmatrix} -2 \\ 1 \\ -2 \end{bmatrix} \) . Find a vector \( \textbf w \) that is orthogonal to vectors \( \textbf u \) and \( \textbf v \).
Solution to Example 2
Using determinants of matrices, the cross product of vectors \( \textbf u = \begin{bmatrix} u_x \\ u_y \\ u_z \end{bmatrix} \) and \( \textbf v = \begin{bmatrix} v_x \\ v_y \\ v_z \end{bmatrix} \) is a vector defined by:

Example 3
Find the constants \( a \) and \( b \) such that the vectors \( \textbf u \begin{bmatrix} a\\ 4 \\ -b \end{bmatrix} \) and \( \textbf u \begin{bmatrix} a\\ 1 \\ b \end{bmatrix} \) are orthogonal and \( a = b + 1\).
Solution to Example 3
Vectors \( \textbf u \) and \( \textbf v \) are orthogonal, hence their inner product is equal to zero
\( a^2 + 4 - b^2 = 0 \)
Given that \( a = b + 1\),substitute \( a \) by \( b + 1\) in the above equation
\( (b+1)^2 + 4 - b^2 = 0 \)
Expand the above equation and simplify
\( 2 b + 5 = 0 \)
Solve for \( b \)
\( b = -\dfrac{5}{2} \)
Find \( a \)
\( a = b + 1 = -\dfrac{5}{2} + 1 = -\dfrac{3}{2} \)
Hence vectors \( \textbf u \) and \( \textbf v \) defined above are orthogonal for \( a = -\dfrac{3}{2} \) and \( b = -\dfrac{5}{2} \) .

Example 4
\( \textbf u \), \( \textbf v \) and \( \textbf w \) are vectors in \( R^n \) such that \[ \textbf w = \textbf v - 4 \alpha \textbf u \] where \( \alpha \) is a real number.
Find \( \alpha \) if \( \textbf u \) and \( \textbf w \) are orthogonal, the norm of \( \textbf u \) is equal to 5 and \( \textbf u^T \textbf v = 3 \).
Solution to Example 4
Since \( \textbf u \) and \( \textbf w \) are orthogonal, their inner product is equal to zero; hence
\( \textbf u \cdot \textbf w = 0 \)
Given that \( \textbf w = \textbf v - 4 \alpha \textbf u \), substitute \( \textbf w \) by \( \textbf v - 4 \alpha \textbf u \) in the above equation
\( \textbf u \cdot (\textbf v - 4 \alpha \textbf u) = 0 \)
Use distributivity and associativity of inner product to expand the left side of the above equation
\( \textbf u \cdot \textbf v - 4 \alpha \textbf u \cdot \textbf u = 0 \)         (I)
The expression \( \textbf u^T \textbf v \) is another form in writing the inner product of \( \textbf u \) and \( \textbf v \) ; hence
\( \textbf u \cdot \textbf v = \textbf u^T \textbf v = 3 \)
The norm of a vector is defined as
\( ||\textbf u|| = \sqrt {\textbf u \cdot \textbf u} \)
Given that the norm of \( \textbf u \) written as \( ||\textbf u|| \) is given and equal to 5, we have
\( \textbf u \cdot \textbf u = ||\textbf u||^2 = 5^2 \)
Substitute \( \textbf u \cdot \textbf v \) and \( \textbf u \cdot \textbf u \) by their values in equation (I) above to obtain
\( 3 - 4 \alpha (25) = 0 \)
Solve the above equation for \( \alpha \) to obtain
\( \alpha = \dfrac{3}{100} \)

Example 5
Find all values of \( \theta \) such that the vectors \( \textbf u \begin{bmatrix} \sin(\theta) \\ \cos(\theta) \end{bmatrix} \) and \( \textbf v \begin{bmatrix} -1 \\ 1 \end{bmatrix} \) are orthognal.
Solution to Example 5
For the vectors \( \textbf u \) and \( \textbf v \) to be orthogonal, the inner product must be equal to 0. Hence
\( \sin(\theta) (-1) + \cos(\theta) (1) = 0 \)
The above equations may be written as
\( \sin(\theta) = \cos(\theta) \)
Divide both sides of the above equation by \( \cos(\theta) \)
\( \dfrac{\sin(\theta)}{\cos(\theta)} = \dfrac{\cos(\theta)}{\cos(\theta)} \)
Simplify and rewrite as
\( \tan (\theta ) = 1 \)
Solve for \( \theta \)
\( \theta = \dfrac{\pi}{4} + n \pi \)

Example 6
Let \( \textbf u = \begin{bmatrix} \sin(\theta) \cos (\phi) \\ \sin(\theta) \sin (\phi) \\ \cos (\theta) \end{bmatrix} \) , \( \textbf v = \begin{bmatrix} \cos(\theta) \cos (\phi) \\ \cos(\theta) \sin (\phi) \\ -\sin (\theta) \end{bmatrix} \) and \( \textbf w = \begin{bmatrix} -\sin (\phi) \\ \cos (\phi) \\ 0 \end{bmatrix} \). Proove that each of these vectors is orthogonal to the other two vectors.
Solution to Example 7
We calculate the inner product of each pair of vectors
\( \textbf u \cdot \textbf v = [\sin(\theta) \cos (\phi)] \; [\cos(\theta) \cos (\phi)] + [\sin(\theta) \sin (\phi)] \; [\cos(\theta) \sin (\phi)] + [\cos (\theta)] \; [-\sin (\theta)] \)
Factor \( \sin(\theta) \cos(\theta) \) out of the first and second terms
\( = [\sin(\theta) \cos(\theta) ] \; [\cos^2 (\phi) + \sin^2 (\phi)] - \cos (\theta) \sin (\theta) \)
Use the identity \( \cos^2 (\phi) + \sin^2 (\phi) = 1 \) to simplify
\( = \sin(\theta) \cos(\theta) - \cos (\theta) \sin (\theta) = 0 \)

\( \textbf u \cdot \textbf w = [\sin(\theta) \cos (\phi)] \; [-\sin (\phi)] + [\sin(\theta) \sin (\phi)] \; [\cos (\phi)] +[\cos (\theta)][0] \)
Factor \( \sin(\theta) \cos(\phi) \) out of the first and second terms and simplify
\( = [ \sin(\theta) \cos(\phi) ] \; [ -\sin (\phi) + \sin (\phi)] + 0 = 0 \)

\( \textbf v \cdot \textbf w = [\cos(\theta) \cos (\phi)] \; [-\sin (\phi)] + [\cos(\theta) \sin (\phi)] \; [\cos (\phi)] +[-\sin (\theta)] \; [0] \)
Factor \( \cos(\theta) \cos(\phi) \) out of the first and second terms and simplify
\( = [ \cos(\theta) \cos(\phi) ] \; [ -\sin (\phi) + \sin (\phi)] + 0 = 0 \)
The inner product of each pair of vectors is equal to zero and therefore each vector is orthogonal to the other two vectors.

More References and links

1. Vector Spaces - Questions with Solutions
2. Linear Algebra Done Right - third edition, 2015 - Sheldon Axler
3. Linear Algebra with Applications - 2012 - Gareth Williams
4. Linear Algebra and its Applications - 5 th Edition - David C. Lay , Steven R. Lay , Judi J. McDonald
5. Elementary Linear Algebra - 7 th Edition - Howard Anton and Chris Rorres