Focus of Parabolic Reflector Calculator

Formula for the Focal Distance of a Parabolic Reflector Given its Depth and Diameter

The equation of a parabola with vertical axis and vertex at the origin is given by
\( y = \dfrac{1}{4f} x^2 \)
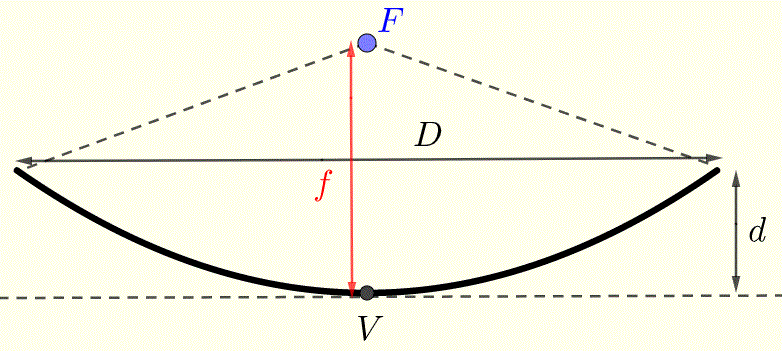
where \( f \) is the focal distance which is the distance between the vertex \( V \) and the focus \( F \).
Let \( D \) be the diameter and \( d \) the depth of the parabolic reflector. Using the diameter \( D \) and the depth \( d \), the point with coordinates (D/2 , d) is on the graph of the parabolic reflector and therefore we can write the equation
\( d = \dfrac{1}{4f} D^2 \)
Solve for \( f \) to obtain
\( f = \dfrac{D^2}{16 d} \)

How to Use the Focal Distance Calculator

Enter the depth d and the diamter D as positive real number and click on "Calcualte". The answer is the focal distance f.
Note that \( D \) and \( d \) must be of the same unit. Both meters, or centimeters, or feet...
The default values are in centimeters.

More References and Links to Parabola