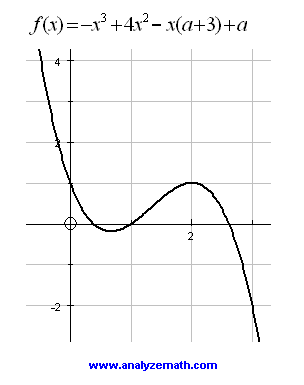The graphs of several third degree polynomials are shown along with questions and answers at the bottom of the page.

1. Polynomial of the third degree: cuts the x axis at one point.
   Question 1: Why does the graph cut the x axis at one point only?
   
   Figure 1: Graph of the third degree polynomial.
   
2. Polynomial of the third degree: 3 x-intercepts.
   Question 2: If the graph cuts the x axis at x = -2, what are the coordinates of the two other x-intercpets?
   
   Figure 2: Graph of a third degree polynomial
   
3. Polynomial of the third degree: 3 x intercepts and parameter a to determine.
   Question 3: The graph below cuts the x axis at x = 1 and has a y-intercpet at y = 1. What are the coordinates of the two other x intercpets?
   
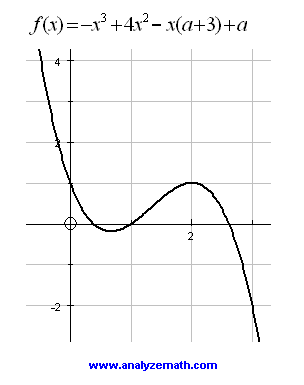
   Figure 3: Graph of a third degree polynomial
   
4. Polynomial of a third degree polynomial: one x-intercepts.
   Question 4: The graph below cuts the x axis at x = -1. Why does the graph of this polynomial have one x intercept only?
   
   Figure 4: Graph of a third degree polynomial, one intercpet.
   

1. Since x = 0 is a repeated zero or zero of multiplicity 3, then the the graph cuts the x axis at one point.
   
   
2. An x intercept at x = -2 means that Since x + 2 is a factor of the given polynomial. Hence the given polynomial can be written as: f(x) = (x + 2)(x² + 3x + 1). Find the other zero, which give the two other x intercpets, by solving the equation x² + 3x + 1 = 0. The solutions are: x = -3/2 + SQRT(5) / 2 and x = -3/2 - SQRT(5) / 2.
   
   
3. Use the y intercept to find a = 1 and then proceed in the same way as was done in question 2 above to find the other 2 x intercepts: 3/2 - SQRT(5) / 2 and 3/2 + SQRT(5) / 2
   
   
4. Factor f as follows: f(x) = (x + 1)(x² + x + 1). In solving the equation x² + x + 1 = 0, the zeros are complex numbers and therefore do not show as x intercepts.

More References and Links to Polynomial Functions