- Solution to Question 1
Given the equation \( 4 = -(x +2)(x-1) \).
Expand the right side of the equation.
\( 4 = -x^2-x+2 \)
Rewrite the equation with right side equal to zero.
\(x^2 + x + 2 = 0 \)
The equation is quadratic with coefficients
\( a = 1 , b = 1 , c = 2 \)
Calculate the discriminant
\( \Delta = b^2 - 4 a c = 1^2 - 4(1)(2) = -7\)
The discriminant is negative and therefore the equation has 2 complex solutions given by
\( x_1 = \dfrac{-b - \sqrt{\Delta}}{2 a} = \dfrac{ - 1 - \sqrt{-7}}{2} = \dfrac{-1}{2} -i \dfrac{\sqrt{7}}{2} \)
\( x_2 = \dfrac{-b + \sqrt{\Delta}}{2 a} = \dfrac{ - 1 + \sqrt{-7}}{2} = \dfrac{-1}{2} + i \dfrac{\sqrt{7}}{2} \)
NOTE \( \sqrt{-7} = \sqrt{-1} \sqrt{7} = i \sqrt{7} \) , in complex numbers \( i = \sqrt{-1} \)
Answer: D
- Solution to Question 2
Given the equations \( 9^{-x\left(-x+5\right)}\:= \dfrac{1}{3^{-12}} \).
One way of solving the above equation is to rewrite the exponentials in the two sides of the equation to the same base.
We have two bases \( 9 \) and \( 3 \). It is easier to work with the small base as follows.
\( 9 = 3^2 \)
Rewrite the above equation replacing \( 9 \) by \(3^2 \)
\( (3^2)^{-x\left(-x+5\right)}\:= \dfrac{1}{3^{-12}} \).
Use rule of exponents \( (a^m)^n = a^{m \cdot n}\) in the left side.
\( 3^{2(-x\left(-x+5\right))}\:= \dfrac{1}{3^{-12}} \)
Use rule of exponents \( \dfrac {1}{a^{-n}} = a^n \) in the right side of the equation.
\( 3^{2(-x\left(-x+5\right))}\:= 3^{12} \)
Since the bases are equal, we conclude that the exponents are also equal, hence the equation
\( 2(-x\left(-x+5\right)) = 12 \)
Expand the left side of the equation
\( 2x^2-10x = 12 \)
Rewrite the equation with right side equal to zero.
\( 2x^2 - 10x - 12 = 0 \)
Solve the above equation for \( x \) using any method to obtain.
\( x = - 1 \) and \( x = 6 \)
Answer: C
- Solution to Question 3
Given the expression \( \dfrac{1}{3 + \sqrt{-4}} \).
First rewrite \( \sqrt{-4} \) as
\( \sqrt{-4} = \sqrt{-1} \sqrt {4} \)
By definition \( \sqrt{-1} \) is the imaginary unit \( i \), hence
\( \sqrt{-4} = \sqrt{-1} \sqrt {4} = (i)(2) = 2 i \)
The given expression may be rewritten as
\( \dfrac{1}{3 + \sqrt{-4}} = \dfrac{1}{3 + 2 i} \)
Multiply numerator and denominator by \( 3 - 2 i \) which is the conjugate of the denominator \( 3 + 2 i \).
\( = \dfrac{1}{3 + 2 i} \times \color{red}{\dfrac{3-2i}{3-2i}} \)
Simplify
\( = \dfrac{3-2i}{9+4} = \dfrac{3-2i}{13} = \dfrac{3}{13} - i \dfrac{2}{13} \)
Answer: A
- Solution to Question 4
Given the expression \( -2(4 - i) \).
Distribute the \( - 2 \) to rewrite the given expression as
\( -2(4 - i) = -8 + 2 i \)
Real part \( -8 \) is negative and the imaginary part \( 2 \) is positive. The graph as a complex number of the given expression is in quadrant II
Answer: D
- Solution to Question 5
Let \( x \) be the distance traveled in kilometers that needs to be calculated.
Ahmed paid 261 AED which includes:
1) a fixed fee of 80 AED per day. For two days he paid \( 2 \times 80 \)
2) for each kilometer he paid 20 fils = 0.20 AED. For \( x \) kilometers he paid \( 0.20 \times x \)
Hence the total of 261 AED he paid may be written as the sum of the two parts 1) and 2) as described above.
\( 261 = 2 \times 80 + 0.20 \times x \)
We now solve for \( x \)
\( x = \dfrac{261 - 2\times 80}{0.20} = 505 \) kilometers
Answer: B
- Solution to Question 6
Given the system of equations \[ \begin{cases} \dfrac{x}{2}+\dfrac{y}{2}=1\\\\ \dfrac{x}{3}+\dfrac{y}{4}=2 \end{cases} \]
One way to solve the above system is to rewrite it without fractions
Multiply all terms of the first equation by the denominator \( 2 \) and all terms of the the second equation by the product of the two denominators \( 3 \times 4 \)
\[ \begin{cases} \color{red}{2}\dfrac{x}{2}+\color{red}{2} \dfrac{y}{2} = \color{red}{2} (1)\\\\ \color{red}{3 \times 4}\dfrac{x}{3}+\color{red}{3 \times 4} \dfrac{y}{4} = \color{red}{3 \times 4} (2) \end{cases} \]
Simplify
\[ \begin{cases} x + y =2\\\\ 4 x + 3 y = 24 \end{cases} \]
You may use any method to solve the above system. Let us use the method of elimination.
Multiply all terms of the first equation in the above system by \( - 3 \) to obtain
\[ \begin{cases} - 3 x - 3 y = - 6\\\\ 4 x + 3 y = 24 \end{cases} \]
Add the first and second equation and put the answer in the first row of the system
\[ \begin{cases} x = 18\\\\ 4 x + 3 y = 24 \end{cases} \]
Substitute \( x \) by \( 18 \) in the equation \( x + y = 2 \) and solve for \( y \)
\( 18 + y = 2 \)
\( y = - 16\)
The solution may be written as an ordered pair \( (x,y) \)
\( (18,-16) \)
Answer: C
- Solution to Question 7
Given \( \dfrac{x^2 - 4y^2}{2y - x} \)
We first need to factor numerator and denominator in order to be able to simplify the given expression.
The numerator has the form of the difference of two squares which may be factored as follows: \( a^2 - b^2 = (a - b)(a +b) \). Hence
\( \dfrac{x^2 - 4y^2}{2y - x} = \dfrac{(x - 2y)(x+2y)}{2y - x} \)
The denominator \( 2y - x \) may be written as
\( 2y - x = - (x - 2y) \)
Use the above to rewrite the given expression as
\( = \dfrac{(x - 2y)(x+2y)}{-(x - 2y)} \)
Divide numerator and denominator by the common factor \( x - 2y \) to obtain
\( = - (x + 2 y ) = - x - 2 y \)
- Solution to Question 8
Given the expression \( \sqrt{200} + \sqrt{32} \).
To group radicals, we need to have the same number under the radicals; it is called the radicand. We also need to express \( 200 \) and \( 32 \) as the product of perfect squares
\( 200 = 2 \times 100 = 2 \times 10^2 \)
\( 32 = 2 \times 16 = 2 \times 4^2 \)
The given expression may be rewritten as
\( \sqrt{200} + \sqrt{32} = \sqrt{2 \times 10^2} + \sqrt{2 \times 4^2} \)
Use the rule \( \sqrt {a \times b} = \sqrt{a} \times \sqrt{b} \)
\( = \sqrt{2} \times \sqrt{10^2} + \sqrt{2} \times \sqrt{4^2} \)
Simplify and group
\( = 10 \sqrt 2 + 4 \sqrt 2 = 14 \sqrt 2 \)
Answer: B
- Solution to Question 9
Given point \( (a , - 3) \) and equation of the curve \( y = x(x-2) \).
For a point to be on the graph of a curve, its coordinates must satisfy the equation.
Substitute the \( x \) and \( y \) coordinates of the given point in the equation of the curve
\( -3 = a(a-2) \)
Expand the right side of the above equation
\( - 3 = a^2 - a \)
Rewrite with right term equal to zero.
\( a^2 - a + 3 = 0 \)
It is a quadratic equation with discriminant
\( \Delta = (-1)^2 - 4(1)(3) = -11\)
Since the discriminant is negative, the above equation has no real solution and therefore no value of \( a \) exists for which the \( (a , - 3) \) is on the graph of the curve.
Answer: C
- Solution to Question 10
Given the equation \( \log_4(x) - \log_4(x+10) = -\log_4(x-2)\).
Rewrite the equation as
\( \log_4(x) + \log_4(x-2) = \log_4(x+10) \)
Use the logarithmic rule \( \log_b(A) + \log_b(B) = \log_b(A \cdot B) \) on the right side to rewrite the equation as
\( log_4 (x(x-2)) = \log_4(x+10) \)
Which gives the algebraic equation
\( x(x-2) = (x+10) \)
Expand the left side and rewrite with right side equal to 0.
\( x^2-3x-10 = 0 \)
Solve using any method to obtain two solutions
\( x_1 = - 2 \) and \( x_1 = 5 \)
NOTE We need to check the solutions in the GIVEN equation because of the domain of the logarithmic functions.
Check x = -2
Left side of the equation
\( \log_4(-2) - \log_4(-2+10) \) ; x = - 2 cannot be a solution because the logarithm of a real number is not real.
Check x = 5
Left side of the equation : \( \log_4(5) - \log_4(5+10) \)
Simplify
\( = \log_4(5) - \log_4(15) \)
Group using the formula \( \log_b(A) - \log_b(B) = \log_b(A / B) \)
\( = \log_4(5/15) \)
Simplify
\( = \log_4(1/3) = \log_4(1) - \log_4(3) = 0 - \log_4(3) = - \log_4(3) \)
right side: \( -\log_4(5-2) = - \log_4(3)\).
Both sides are equal and therefore \(x = 5 \) is a solution to the given equation.
Answer: D
- Solution to Question 11
Given the equation \( 2 - \dfrac{1}{x(x+1)} = \dfrac{3}{x+1} \).
None of the solutions can be equal to \( 0 \) or \( -1 \) because these values make the denominators equal zero which is not allowed in mathematics.
Multiply all terms of the equation by \( x(x+1) \)
\( 2 \cdot \color{red} {x(x+1)} - \dfrac{1}{x(x+1) } \cdot \color{red} {x(x+1) } = \dfrac{3}{x+1} \cdot \color{red} {x(x+1)} \)
Simplify common factors
\( 2 x(x+1) - 1 = 3 x \)
Expand and group to rewrite equations as
\( 2x^2-x-1 = 0 \)
Solve the above by any method to obtain two solutions
\( x_1 = -1/2 \) and \( x_2 = 1 \)
Answer: C
- Solution to Question 12
Given \( f(x) = \sqrt{x + 2} - 4 \).
Let \( a = f^{-1}(-2) \) and find \( a \).
From the definition of the inverse functions, \( a = f^{-1}(-2) \) is equivalent to \( f(a) = - 2 \)
Which means we need to solve the equation \( \sqrt{a + 2} - 4 = - 2 \) in order to find \( a \)
Rewrite the equation as
\( \sqrt{a + 2} = 2 \)
Square both sides
\( (\sqrt{a + 2})^2 = 2^2 \)
Simplify
\( a + 2 = 4 \)
Solve for \( a \)
\( a = 2 \)
\( f^{-1}(-2) = 2 \)
Answer: A
- Solution to Question 13
Given the expression \( \sin(4x) \).
Use the trigonometric identity \( \sin(2x) = 2 \sin x \cos x \) to rewrite the given expression as
\( \sin(4x) = \sin( 2(2x)) \\\\ = 2 \sin(2x) \cos(2x) \)
Use the above identity a second time on the term \( \sin(2x) \)
\( = 2 \cdot 2 \sin(x) \cos(x) \cos(2x) \\= 4 \sin(x) \cos(x) \cos(2x) \)
Answer: B
- Solution to Question 14
Given \( \overrightarrow{v_1} = <1,-2> \) and \( \overrightarrow{v_2} = <-2,4> \)?
\( \overrightarrow v = 2 \overrightarrow{v_1} - 3 \overrightarrow {v_2} \)
Substitute \( \overrightarrow{v_1} \) and \( \overrightarrow{v_1} \) by their components, multiply and simplify
\( \overrightarrow v = 2<1 , -2> - 3<-2 , 4> \\ = <2 , -4> + <6 , -12> \\ = <8 , -16>\)
The magitude of \( \overrightarrow v \) is given by
\( | \overrightarrow v | = \sqrt{8^2+(-16)^2} = 8 \sqrt{5}\)
Answer: C
- Solution to Question 15
Let \( P(t) \) be the production as a function of time \( t \) in years. Since the production varies linearly with time , it can be written as
\( P(t) = m t + b \)
with \( t = 0 \) corresponding to two years ago when 2000 toys were produced or \( P(0) = 2000 \)
This year corresponds to \( t = 2 \) and the production is 2400 or \( P(2) = 2400\)
The slope \( m \) of the linear function may be calculated as follows
\( m = \dfrac{2400 - 2000} {2 - 0} = 200 \) toys per year
We find parameter \( b \) using either \( P(0) = 2000 \) or \( P(2) = 2400\).
\( P(0) = m(0) + b = b = 2000 \)
\( P(t) = 200 t + 2000 \)
In four years time, \( t = 6 \) ; hence
\( P(6) = 200(6) + 2000 = 3200 \) toys
Answer: A
- Solution to Question 16
Given the inequality \( \quad x + 4 \le \dfrac{3}{x+2} \).
Rewrite the inequality with right side equal to zero.
\( \quad x + 4 - \dfrac{3}{x+2} \le 0 \)
Multiply and divide \( x + 4 \) by \( x + 2 \)
\( \quad (x + 4) \cdot \color{red}{\dfrac{x + 2}{x + 2}} - \dfrac{3}{x+2} \le 0 \)
The two rational expressions on the left have common denominator and may grouped as follows
\( \quad \dfrac{(x + 4)(x + 2)- 3 }{x + 2} \le 0 \)
Expand numerator and group like terms
\( \quad \dfrac{x^2+6x+5}{x + 2} \le 0 \)
Factor numerator
\( \quad \dfrac{(x+1)(x+5)}{x + 2} \le 0 \)
The numerator has two zeros: \( x = - 1 \) and \( x = - 5 \) and the denominator has one zero \( x = - 2 \) which are all used in the table of signs below.

The solution set is given by the intervals where the expression on the right side of the inequality is less than or equal to zero.
The solution set is given by : \( (-\infty ,-5] \cup (-2,-1] \)
Answer: C
- Solution to Question 17
Given \( (-x+2)(x-1) - ( x^2 - 2x +1) \)
Exapnd
\( (-x+2)(x-1) - ( x^2 - 2x +1) = -x^2 + x + 2x - 2 - x^2 + 2x -1 \)
Group like terms
\( = -2x^2 + 5x - 3 \)
Answer: D
- Solution to Question 18
Factor \( 3x^2 + 4x - 4 \).
Write \(4x\) as \(6x - 2x\)
\( 3x^2 + 4x - 4 = 3x^2 + 6x -2x - 4 \)
Group
\( = (3x^2 + 6x) - (2x+4) \)
Factor each term
\( = 3x(x + 2) - 2(x + 2) \)
Factor \( x +2 \) out
\( = (x+2)(3x-2) \)
- Solution to Question 19
Let \( x \) and \( y \) be the two numbers with \( x \gt y \)
We are given the difference is equal to 2, hence
\( x - y = 2 \) (eq 1)
We are given the product equal to 99, hence
\( x y = 99 \) (eq 2)
Equation (1) gives
\( y = x - 2 \)
Substitute \( y \) by \( x - 2 \) in equation (2) to obtain
\( x (x - 2) = 99 \)
Expand and rewrite with right side equal to zero.
\( x^2 - 2 x - 99 = 0 \)
Solve to obtain two solutions
\( x = 11 \) and \( x = - 9 \)
we are looking for positive numbers; hence we select \( x = 11 \)
\( y = x - 2 = 11 - 2 = 9 \)
The two numbers are \( 9 \) and \( 11 \).
- Solution to Question 20
Given \( \quad x y = \dfrac{y - 1}{x - 1} \).
Multiply all terms in the two sides by \( x - 1 \)
\( x y \cdot \color{red}{(x - 1 )} = \dfrac{y - 1}{x - 1} \cdot \color{red}{(x -1)}\).
Simplify
\( x y (x - 1) = y - 1 \)
Expand
\( x^2 y - x y = y - 1 \)
Rewrite with all terms with \( y \) on the left
\( x^2 y - x y - y = - 1 \)
Factor \( y \) out
\( y(x^2 - x - 1) = - 1 \)
Divide both sides by \( x^2 - x - 1 \)
\( \dfrac{y(x^2 - x - 1)}{x^2 - x - 1} = - \dfrac{1}{x^2 - x - 1} \)
Simplify
\( y = - \dfrac{1}{x^2 - x - 1} = \dfrac{1}{-x^2 + x + 1} \)
- Solution to Question 21
Use the sum and difference of z and y to write the equations
\( z + y = 74\)
\( z - y = 12\)
Add sides of the above equations to eliminate \( y \)
\( 2z = 86 \)
Solve for \( z \)
\( z = 43 \)
Substitute \( z \) by \( 43 \) in the equation \( z + y = 74\) to obtain
\( 43 + y = 74\)
Solve for \( y \)
\( y = 31 \)
The sum of \( x , y \) and \( z \) is equal to 96 may be written as the equation
\( x + y + z = 96\)
Substitute \( y \) and \( z \) by their values to obtain the equation
\( x + 31 + 43 = 96\)
Solve for \( x \)
\( x = 22 \)
The three numbers are
\( 22 , 31\) and \( 43 \)
- Solution to Question 22
An x-intercept on the graph is a zero of the polynomial and the zeros gives the factors. Hence the three x - intercepts in the graphs give the factors:
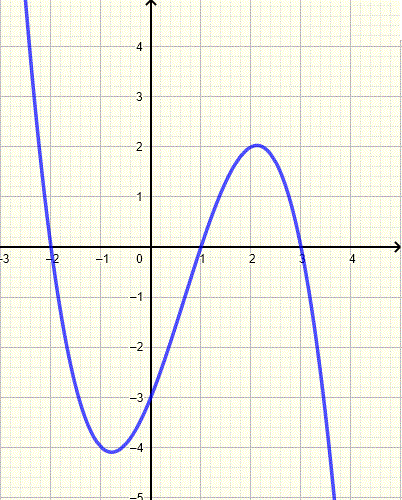
\( x + 2 \) , \( x - 1 \) and \( x - 3 \).
The polynomial \( P \) may be written as
\( P(x) = a (x+2)(x-1)(x-3) \)
We now need to find the leading coefficient \( a \) using the y - intercept given \( P(0) = - 3 \)
\( P(0) = a (0+2)(0-1)(0-3) = - 3 \)
Simplify
\( 6 a = - 3 \)
\( a = -0.5 \)
\( P(x) = -0.5 (x+2)(x-1)(x-3) \)
Answer: B
- Solution to Question 23
By definition
\( (f_o g)(0) = f(g(0)) \)
\( g(0) = \dfrac{1}{0-2} = - 1/2 \)
Hence
\( (f_o g)(0) = f(g(0)) = f(-1/2) = (-1/2) ^2 - 1 = -3/4 \)
Answer: A
- Solution to Question 24
From 8:00 am, the amount of caffeine halved after 5 hours which is at 1:00 pm. The amount of caffeine became
60 milligrams
From 1:00 pm to 6:00 pm there are 5 hours over which the amount of caffeine will halve again, because of the exponential behavior, and become
30 milligrams
Answer: D
- Solution to Question 25
Two things to consider carefully: the coefficient of \( z \) in the second equation is \( 0 \) and the order of the variables in the third equation has to be changed and the equation rewritten as
\( -2x - 4 y + 2 z = 0 \)
and according to the multiplication of matrices, the answer is B.
Geometry Questions to Practice for the EmSAT
- Solution to Question 26
Given \( \quad 2(x - 2)^2 + 2(y + 2 )^2 = 32 \)
We first divide all terms of the equation by \( 2 \) so that the equation is written in standard form
\( (x - 2)^2 + (y + 2 )^2 = 16\)
Rewrite in standard form \( \quad (x - h)^2 + (y - k )^2 = r^2 \)
\( (x - 2)^2 + (y - (-2) )^2 = 4^2 \)
compare to the standard form to determine the center \( (h,k) = (2,-2) \) and radius \( r = 4 \)
Answer: C
- Solution to Question 27
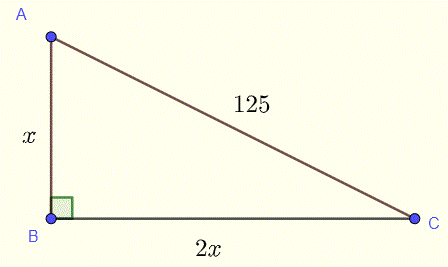
Use the Pythagorean theorem to write
\( x^2 + (2x)^2 = 125^2 \)
Simplify and group
\( 5x^2 = 125^2 \)
Divide both sides by \( 5 \)
\( x^2 = 125^2 / 5 \)
Take the square root of both sides
\( x = 25\sqrt{5} \)
Answer: D
- Solution to Question 28
Since AB is parallel to CD, the two triangles OCD and OBA are similar, hence the proportionality of the sides
\( \dfrac{ \overline{OC}}{\overline{OB}} = \dfrac{\overline{OD}}{\overline{OA}} = \dfrac{\overline{CD}}{\overline{AB}} \)
Substitute
\( \dfrac{ 2.5}{y} = \dfrac{x}{5} = \dfrac{4}{6} \)
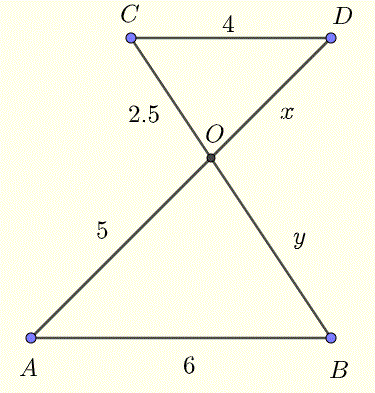
Deduce two equations
\( \dfrac{x}{5} = \dfrac{4}{6} \)
\( \dfrac{ 2.5}{y} = \dfrac{4}{6} \)
Solve each equation to find
\( x = 10/3 \) and \( y = 15/4 \)
Answer: B
- Solution to Question 29
Let \( x \) be the side of the square and \( r \) be the radius of the circle. Any two opposite vertices of the square such as \( A \) and \( B \) shown in the figure below are distant by a diameter which is equal to \( 2r \).

The area of the the shaded region is equal to the difference of the area of the circle \( \pi r^2 \) and the area of the square \( x^2 \) . hence
\( \pi r^2 - x^2 = 10\)
Also the use of the Pythagorean theorem to one of the two triangles gives
\( x^2 + x^2 = (2r)^2 \)
The above equation gives
\( x^2 = 2 r^2 \)
Substitute \( x^2 \) by \( 2r^2 \) in the equation \( \pi r^2 - x^2 = 10\) to obtain
\( \pi r^2 - 2r^2 = 10\)
Fcator \( r^2 \) out
\( r^2(\pi - 2) = 10\)
Solve for \( r \)
\( r = \sqrt{\dfrac{10}{\pi - 2}} \approx 3.0 \)
Answer: B
- Solution to Question 30
The area \( A_r \)of the right triangle ABC may be calculated in two ways
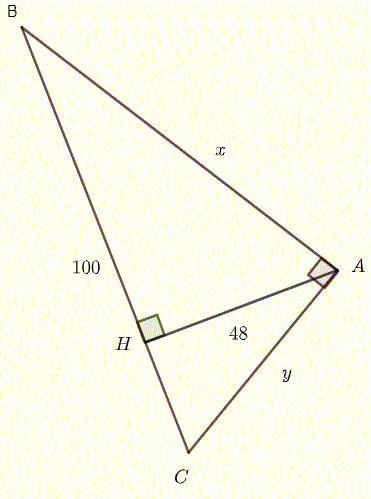
\( A_r = 0.5 x y = 0.5 (48)(100) \)
hence
\( x y = 4800 \) (eq 1)
The use of the Pythagorean theorem gives
\( x^2 + y^2 = 100^2 \) (eq 2)
Equation (1) gives
\( y = 4800 / x \)
Substitute y by 4800 / x in equation (2) to obtain
\( x^2 + (4800/x)^2 = 100^2 \)
which may be written as
\( x^2 + 23040000 / x^2 = 100^2 \)
Multiply all terms by \( x^2\) and simplify to obtain
\( x^4 + 23040000 = 10000 x^2 \)
Let \( u = x^2 \) and rewrite the above equation in terms of \( u \)
\( u^2 - 10000 u + 23040000 = 0\)
Solve by any method to find two solutions
\(u_1 = 6400 \) and \(u_2 = 3600 \)
Find \( x \) using
\( u = x^2 = 6400 \)
which gives
\( x = 80 \) and \( y = 4800 / x = 4800 / 80 = 60 \)
\( u = x^2 = 3600 \) which gives
\( x = 60 \) and \( y = 3600 / x = 4800 / 60 = 80 \)
We have two solutions but we need to select the one where \( x \gt y \); hence
\( x = 80 \) and \( y = 60 \)
- Solution to Question 31
If the area of the parallelogram is 300, the area of triangle DAB is half the total 300 which is 150.

Hence
Area of triangle DAB \(= 0.5 \sin(\angle A) \times \overline{AD} \times \overline{AB} = 0.5 \times \sin(\angle A) \times 30 \times 20 = 150 \)
which gives
\( \sin(\angle A) = 0.5 \)
\( \angle A =\arcsin(0.5) = 30^{\circ} \) or \( \angle A = 180^{\circ} - 30^{\circ} = 150^{\circ} \)
We select the size of \( \angle A \) to be obtuse
\( \angle A = 150^{\circ} = \angle C \)
\( \angle B = 180^{\circ} - 150^{\circ} = 30^{\circ} = \angle D \)
Answer: A
- Solution to Question 32

The U-shaped structure may be considered as a large rectangular bloc 10 by 3 by 8 from which was a smaller rectangular bloc x by x by 3 was cut.
The given volume may be written as
\( 165 = 10 \times 3 \times 8 - x \times x \times 3 \)
\( 165 = 240 - 3x^2 \)
which may be written as
\( 3x^2 = 75 \)
Solve for \( x \)
\( x = 5 \) cm
Answer: C
Statistics and Probabilities Questions to Practice for the EmSAT
- Solution to Question 33
The probability that Saef will watch the match on TV is 0.7 and the probability that his team wins is 0.5. What is the probability that Saef will not watch the match and his team wins the match?
Let event A be: "Saef will not watch the match"
Let event B be: "his team wins the match"
\( P(A) = 1 - 0.7 = 0.3 \) rule of the complement
\( P(B) = 0.5 \)
The two events are independent, hence
\( P (\text{Saef will not watch the match and his team wins the match}) = P(A \; and \; B) = P(A) \times P(B) = 0.3 \times 0.5 = 0.15 \)
Answer: D
- Solution to Question 34
Given the set: \( \{ -4,-1,0,2,5,6,7,10\} \).
Let \( S \) be the sample space, hence
\( S = \{ -4,-1,0,2,5,6,7,10 \} \)
Let \( n(S) \) be the number of elements in \( S \), hence
\( n(S) = 8 \)
Let \( E \) be the event: "select a number that is either negative or greater than 6 ", hence
\( E = \{ -4,-1,7,10 \} \)
Let \( n(E) \) be the number of elements in \( E \), hence
\( n(E) = 4 \)
\( P(\text{number is either negative or greater than 6}) = P(E) = n(E) / n(S) = 4/8 = 1/2 \)
Answer: C
- Solution to Question 35
Let event A: Sultan travels to Spain
Let event B|A: Sultan travels to England knowing that he has traveled to Spain (conditional probability)
Hence
\( P(B|A) = \dfrac{P(A \cap B) }{P(A)} = \dfrac{0.3}{0.5} = 0.6 \)
Answer: A
- Solution to Question 36
Let \( A \) be the total grades for the two brothers, hence
\( A = 2 \times 89 = 178 \)
Let B be the total grades for the four sisters, hence
\( B = 4 \times 92 = 368 \)
The average of all the 6 brothers and sisters is given by
\( \dfrac{\text{total grades}}{6} = \dfrac{178+368}{6} = 91 \)
Answer: B
- Solution to Question 37
Z-score normalization of \( 590 \) is given by
\( Z = \dfrac{590 - 500}{100} = 0.9 \)
We now use the table of values of the normal distribution to find the percentage \( P \) as
\( P = 0.81594 = 81.594\% \approx 82\%\)
Answer: C
- Solution to Question 38
The tree diagram is shown below shows all possible outcomes of the three games to be played by Majed.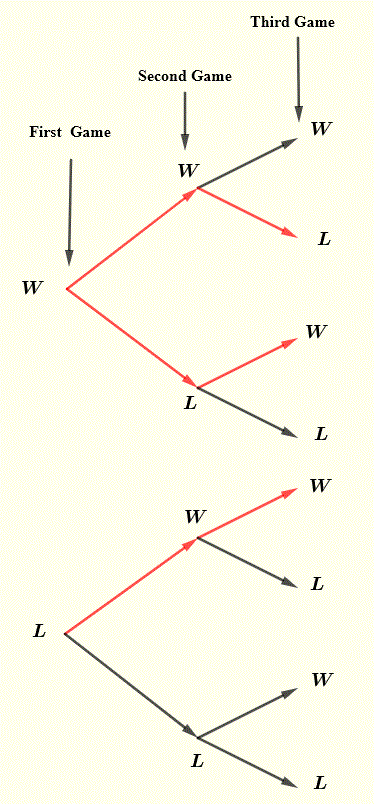
All the "red" outcomes have exactly two wins.
\( P(\text{Majed wins exactly 2 out of the next three games}) = P \left( (WWL) or (WLW) or (LWW) \right) = P(WWL) + P(WLW) + P(LWW) \) (addition rule of probabilities)
\( P(\text{Majed wins}) = P(W) = 3/4 \)
\( P(\text{Majed looses}) = 1 - P(W) = 1 - 3/4 = 1/4\) rule of the complement
The results of the 3 games are independent; hence
\( P(WWL) = P(W) \times P(W) \times P(L) = \dfrac{3}{4} \cdot \dfrac{3}{4} \cdot \dfrac{1}{4} = 9/64 \) (multiplication rule of probabilities)
\( P(WLW) = P(W) \times P(L) \times P(W) = \dfrac{3}{4} \cdot \dfrac{1}{4} \cdot \dfrac{3}{4} = 9/64 \)
\( P(LWW) = P(L) \times P(W) \times P(W) = \dfrac{1}{4} \cdot \dfrac{3}{4} \cdot \dfrac{3}{4} = 9/64 \)
\( P(\text{Majed wins exactly 2 out of the next three games}) = 9/64 + 9/64 + 9/64 = 27/64 \)
Answer: D
Calculus Questions to Practice for the EmSAT
- Solution to Question 39
Given function \( f(x) = - 4x^3 + 3x^2 - 2x - 2 \).
Use the power rule of derivative \( (x^n)' = n x^{n-1} \) and the addition of functions rule
\( f'(x) = -12x^2+6x-2 \)
- Solution to Question 40
Given the function \( f(x) = (x^3 - 2x^2 + x)(2x - 7) \).
Use the product rule of derivative \( (U V)' = U' V + U V' \)
\( f'(x) = (3x^2 - 4x + 1) (2x - 7) + (x^3 - 2x^2 + x)(2) \)
Expand and group like terms
\( f'(x) = 8x^3-33x^2+32x-7 \)
- Solution to Question 41
Given the function \( f(x) = \sqrt{-3x+3} \).
Let \( u = -3x+3 \) and rewrite \( f \) as
\( f(x) = \sqrt{-3x+3} = \sqrt{u(x)} = u(x)^{1/2}\)
Use the chain rule and power rule
\( f'(x) = u'(x) (1/2) u^{1/2-1} \\\\ = -3 (1/2)u^{-1/2} \\ = - \dfrac{3}{2\sqrt{-3x+3}} \).
- Solution to Question 42
Given the function \( f(x) = (x^2+1)^5 \).
Let \( u = x^2+1 \) and rewrite \( f \) as follows
\( f(x) = u^5 \)
Use the chain rule and power rule
\( f'(x) = u'(x) 5 u^{5-1} = 5 (2x) u^4 = 10 x u^4 \)
Use the product rule of the derivative \( (U V)' = U' V + U V' \) to find the second derivative
\( f''(x) = 10 u^4 + 10 x u' 4 u^3 \\ = 10 (x^2+1)^4 + 10 x (2x) 4 (x^2+1)^3 \\ = 10 ( (x^2+1)^4 + 8 x^2 (x^2+1)^3 ) \)
- Solution to Question 43
Given the function \( f(x) = \dfrac{1}{x-1} \).
Use the quotient rule of derivative \( (u/v)' = (u'v - uv') / v^2 \)
\( f'(x) = \dfrac{(1)'(x-1) - (1)(1)} {(x-1)^2} = \dfrac{-1} {(x-1)^2} \)
- Solution to Question 44
Given \( f(x) = \dfrac{x-1}{x+3} \).
Use the quotient rule of derivative \( (u/v)' = (u'v - uv') / v^2 \)
\( f'(x) = \dfrac{(1)(x+3) - (x-1)(1)}{(x+3)^2} \)
\( f'(2) = \dfrac{(1)(2+3) - (2-1)(1)}{(2+3)^2} = 4/25\)
Answer: B
- Solution to Question 45
Given \( f(x) = \cos(2x - 2) \).
Let \( u = 2x - 2 \) and write \( f \) as
\( f(x) = \cos(u(x)) \)
Use the chain rule to find \(f'\)
\( f'(x) = u'(x) (-\sin(u(x))) = - 2 \sin(2x-2) \)
Answer: D
- Solution to Question 46
Given \( f(x) = k x^2 + 2x -1 \).
Find the derivative of \( f \)
\( f'(x) = 2 k x + 2 \)
\( f'(1) = 2 k (1) + 2 = 2 k + 2 = 0 \)
Solve for \( k \)
\( 2 k + 2 = 0 \)
\( k = - 1 \)
Answer: A
- Solution to Question 47
Given \( f(x) = \dfrac{2x^2 + x}{x^2-1} \).
Find \( f' \)
\( f'(x) = \dfrac{-x^2-4x-1}{\left(x^2-1\right)^2} \)
\( \dfrac{-x^2-4x-1}{\left(x^2-1\right)^2} = 0 \)
For the above to be zero, the numerator must be equal to zero. Hence
\( -x^2-4x-1 = 0 \)
Two solutions
\( x=-2-\sqrt{3} \; , \; x=-2+\sqrt{3} \)
Answer: C
- Solution to Question 48
Find the limit \( \lim_{x\to\infty} \dfrac{x^3-2x+4}{-2x^3+x^2-1} \).
Divide all terms in the numerator and all terms in the denominator by the terms with the highest power which is \( x^3 \)
\( \lim_{x\to\infty} \dfrac{x^3-2x+4}{-2x^3+x^2-1} = \lim_{x\to\infty} \dfrac{x^3/x^3-2x/x^3+4/x^3}{-2x^3/x^3+x^2/x^3-1/x^3} \)
Simplify
\( = \lim_{x\to\infty} \dfrac{1-2/x^2+4/x^3}{-2 + 1/x-1/x^3} \)
Any term of the form \( k / x^n \) where \( k \) is a constant and \( n \ge 1 \) will have a limt equal to zero as \( {x\to\infty} \); hence
\( = \lim_{x\to\infty} \dfrac{1-0+0}{-2 + 0 - 0} = -1/2 \)
Answer: B
- Solution to Question 49
\( \lim_{x\to + 4} \dfrac{\sqrt{x} - 2}{x - 4} = \dfrac{\sqrt{4} - 2}{4 - 4} = 0 / 0 \)
It is an indeterminate form and we need to find another way.
Multiply numerator and denominator by \( (\sqrt{x} + 2) \)
\( \lim_{x\to + 4} \dfrac{\sqrt{x} - 2}{x - 4} = \lim_{x\to + 4} \dfrac{(\sqrt{x} - 2)(\sqrt{x} + 2)}{(x - 4)(\sqrt{x} + 2)} \)
Expand the numerator
\( = \lim_{x\to + 4} \dfrac{x-4}{(x - 4)(\sqrt{x} + 2)} \)
Simplify the common factor \( x-4 \)
\( = \lim_{x\to + 4} \dfrac{1}{(\sqrt{x} + 2)} = \dfrac{1}{(\sqrt{4} + 2)} = 1/4\)
Answer: D
- Solution to Question 50
\( \lim_{x\to - 3} \dfrac{x^2 + 4x + 3}{x^2 - 9} = \dfrac{(-3)^2 + 4\times(-3) + 3}{(-3)^2 - 9} = 0 / 0 \)
It is an indeterminate form and we need to find another way.
Factor the numerator and denominator
\( \lim_{x\to - 3} \dfrac{x^2 + 4x + 3}{x^2 - 9} = \lim_{x\to - 3} \dfrac{(x+3)(x+1)}{(x-3)(x+3)} \)
Simplify the common factor \( x + 3 \)
\( = \lim_{x\to - 3} \dfrac{(x+1)}{(x-3)} = \dfrac{-3+1}{-3-3} = 1/3\)
Answer: C
Algebra Questions to Practice for the EmSAT
\( \) \( \) \( \) \( \)More References and links
EmSAT practice questionsAlgebra Questions and Problems with Solutions.
Geometry Problems with Solutions.
elementary statistics and probabilities.
Calculus Questions and Problems with Solutions.
Home Page