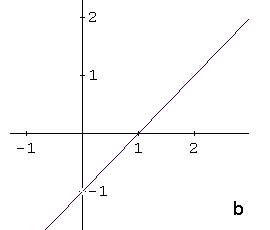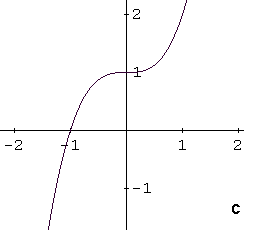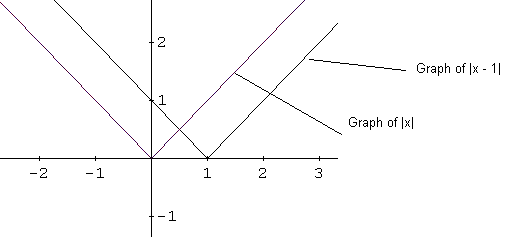Identify Graphs of Functions - Tutorials

SEARCH THIS SITE

Enter your search terms

Web www.analyzemath.com Submit search form

You may want to go through tutorials on graphing before starting this tutorial.

Example 1 : Identify the graph of function    f(x) = -x² - 1

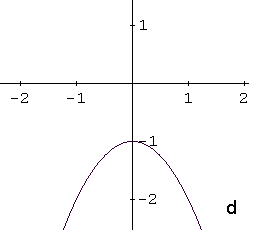
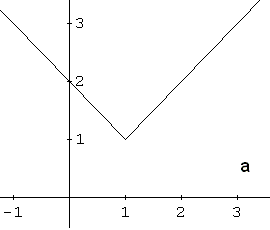
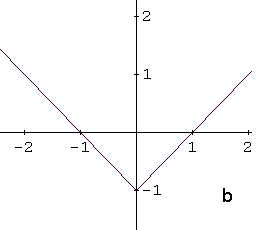
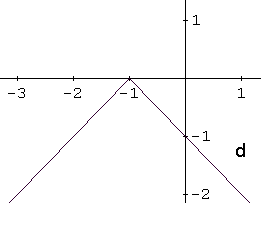
Solution to Example 1:

The given function f(x) = -x² - 1 is a quadratic one and its graph is a parabola. Writing function f in the form f(x) = a(x - h)² + k   makes it easy to graph. The x and y coordinates of the  vertex are given respectively by h and k. When coefficient a is positive the parabola opens upward. If a is negative the parabola opens downward. 

The given function f can be written as f(x) = -(x - 0)² - 1. Its vertex has x coordinate equal to 0 and y coordinate equal to -1. Its coefficient a is negative and the parabola opens downward. Also its y intercept  is at (0 , -1).

The above properties of the graph of function f shows that the graph corresponding to the given equation is graph d.

Example 2 : Identify the graph of function    f(x) = |x - 1| + 1

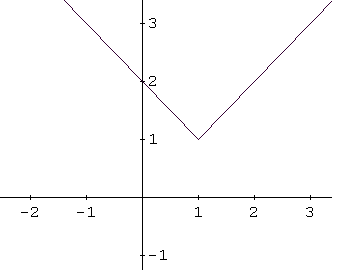
Solution to Example 2:

The given function f(x) = |x - 1| + 1 is related to the absolute value function |x|. We now use a different strategy from the one we used in example 1. The graph of |x - 1| is the graph of |x| that has been shifted one unit to the right as shown below.

The graph of |x - 1| + 1 is the graph of |x - 1| shifted one unit up as shown below.

The above graph correspond to graph a in example 2.

Now that you have worked on the above examples you may go to self test on graphs of functions and solve similar questions.