This is tutorial on finding the points of intersection of a hyperbola and a line given by their equations.

Example 1: Find the points of intersection of a hyperbola and a line given by their equations as follows:

Solution to Example 1:

• Solve the equation of the line for x to obtain:
  x = 3 - 5y
  
• We now substitute x by 3 - 5y into the equation of the hyperbola to obtain
  (3 - 5y)² / 9 - y² = 1
  
• Expand and group like terms and rewrite the equation as
  16y² -30y = 0
  
• Solve the quadratic equation for y to obtain two solutions
  y = 0 and y = 15/8
  
• We now substitute the values of y already obtained into x = 3 - 5y to obtain
  for y = 0, x = 3 and for y = 15/8, x = -51/8
  
• The 2 points of intersection of the the hyperbola and the line are
  ( 3 , 0) ; ( -51/8 , 15/8)

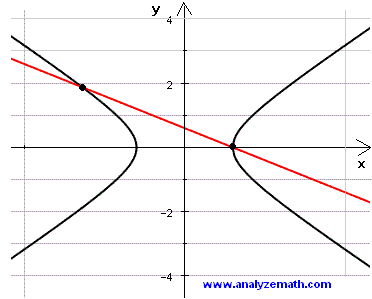
Shown below is the graph of a hyperbola, a line and their points of intersection.

More References and Links to Hyperbolas