Grade 10 questions on how to add and subtract expressions with radicals and their solutions are presented.

Definition Radical expressions are like if they have the same index and the same radicand. Examples are like radicals because they have the same index (root number which is 3) and the same radicand (number under the radical which is 5. are not like radicals because they have different radicands 8 and 9. are like radicals because they have the same index (2 for square root) and the same radicand 2 x. Add and Subtract Like Radicals Only like radicals may be added or subtracted. Examples Simplify the following expressions Solutions to the Above Examples The above expressions are simplified by first factoring out the like radicals and then adding/subtracting. 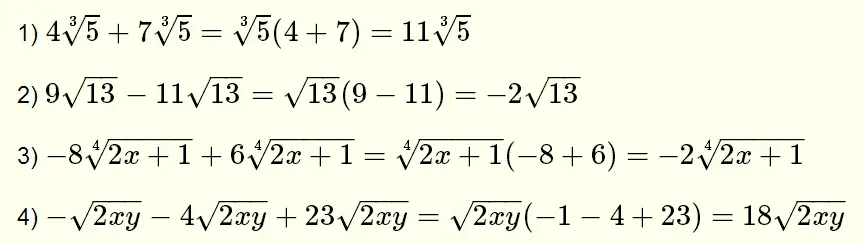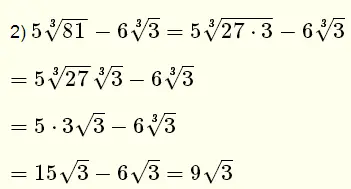More Examples Simplify the following expressions Solutions to Above Examples The above expressions are simplified by first transforming the unlike radicals to like radicals and then adding/subtracting When it is not obvious to obtain a common radicand from 2 different radicands, decompose them into prime numbers. Decompose 12 and 108 into prime factors as follows. 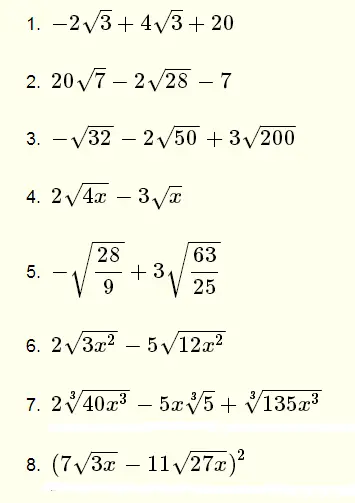We now substitute 12 and 108 by their prime factors and simplify Questions With Solutions Simplify the following expressions Solutions 3. The 3 radicands in the given expression -√ 32 - 2√ 50 + 3√ 200 are different but note that 32, 50 and 200 may be written as 2 times a number that is a perfect square as follows: 32=2 * 16, 50=2 * 25 and 100=2 * 100. Substitute in the given expression and simplify. Decompose 28 and 63 into prime factors as follows: 28=2 2 * 7 , 63=3 2 * 7 and substitute into the given expression and simplify 7.