Solve Trigonometric Equations
How to solve trigonometric equations? Questions are presented along with detailed solutions and explanations.
Examples with Solutions
Example 1
Find all the solutions of the trigonometric equation \[ \sqrt{3} \sec(\theta) + 2 = 0\]
Solution to Example 1
Using the identity \(\sec(\theta) = \dfrac{1}{\cos(\theta)}\), we rewrite the equation in the form
\[
\cos(\theta) = -\dfrac{\sqrt{3}}{2}
\]
Find the reference \(\theta_r\) angle by solving the above equation without the minus sign:
\[
\cos(\theta_r) = \dfrac{\sqrt{3}}{2}
\]
where \(\theta_r\) is acute and equal to the
reference angle .
The solution to the equation \( \cos(\theta_r) = \dfrac{\sqrt{3}}{2} \) is given by:
\[
\theta_r = \dfrac{\pi}{6}
\]
Use the reference angle \(\theta_r\) to determine the solutions \(\theta_1\) and \(\theta_2\) in the interval \([0 , 2\pi)\) of the given equation. The equation \(\cos(\theta) = -\dfrac{\sqrt{3}}{2}\) suggests that \(\cos(\theta)\) is negative and that means the terminal side of angle \(\theta\) solution to the given equation is either in quadrants II or III as shown below using the unit circle.
Hence the solutions in the interval\( [0 , 2 \pi) \):
\[ \theta_1 = \pi - \theta_r = \pi - \dfrac{\pi}{6} = \dfrac{5\pi}{6}\]
\[ \theta_2 = \pi + \theta_r = \pi + \dfrac{\pi}{6} = \dfrac{7\pi}{6}\]
Use the solutions in the interval [0 , 2 \pi) to find all solutions by adding multiples of \( 2 \pi \) as follows:
\[ \theta_1 = \dfrac{5\pi}{6} + 2n\pi ,\quad n = 0, \pm 1 , \pm 2, \ldots\]
\[ \theta_2 = \dfrac{7\pi}{6} + 2n\pi ,\quad n = 0, \pm 1 , \pm 2, \ldots\]
Below are shown the graphical solutions on the interval \( [0 , 2 \pi) \)
.
Example 2
Solve the trigonometric equation \[ 2 \sin(\theta) = -1 \]
Solution to Example 2
Rewrite the above equation in simple form as shown below.
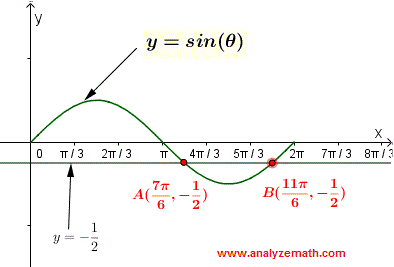
\[\sin(\theta) = -\dfrac{1}{2}\]
Find the reference angle \(\theta_r\) angle by solving equation without the minus sign:
\[ \sin(\theta_r) = \dfrac{1}{2}\]
\( \theta_r\) is acute and equal to
\[\theta_r = \dfrac{\pi}{6}\]
Use the reference angle \(\theta_r\) to determine the solutions \(\theta_1\) and \(\theta_2\) on the interval \([0 , 2\pi)\) of the given equation. The equation \(\sin(\theta) = -\dfrac{1}{2}\) suggests that \(\sin(\theta)\) is negative and that means the terminal side of angle \(\theta\) is either in quadrants III or VI as shown in the unit circle below.
Hence the solutions:
\[\theta_1 = \pi + \theta_r = \pi + \dfrac{\pi}{6} = \dfrac{7\pi}{6}\]
\[ \theta_2 = 2\pi - \theta_r = 2\pi - \dfrac{\pi}{6} = \dfrac{11\pi}{6}\]
Use the solutions on the interval \([0 , 2\pi)\) to find all solutions by adding multiples of \(2\pi\) as follows:
\[\theta_1 = \dfrac{7\pi}{6} + 2n\pi , \quad n = 0, \pm 1 , \pm 2, \ldots\]
\[\theta_2 = \dfrac{11\pi}{6} + 2n\pi , \quad n = 0, \pm 1 , \pm 2, \ldots\]
.
Example 3
Solve the trigonometric equation \[
\sqrt{2} \cos\left(3x + \dfrac{\pi}{4}\right) = -1
\]
Solution to Example 3
Let \( \theta = 3x + \dfrac{\pi}{4} \) and rewrite the equation in simple form.
\[ \sqrt{2} \cos(\theta) = -1 \]
\[ \cos(\theta) = -\dfrac{1}{\sqrt{2}} \]
Find the reference angle \( \theta_r \) by solving \( cos(\theta_r) = \dfrac{1}{\sqrt{2}} \) for \( \theta_r \) acute.
\[ \theta_r = \dfrac{\pi}{4} \]
Use the reference angle \(\theta_r\) to determine the solutions \(\theta_1\) and \(\theta_2\) on the interval \([0 , 2\pi)\) of the given equation.
The equation \( \cos(\theta) = - \dfrac{1}{\sqrt{2}}\) suggests that \(\cos(\theta)\) is negative and that means the terminal side of angle \(\theta\) is either in quadrants II or III. Hence the two solutions of the equation \(\cos(\theta) = - \dfrac{1}{\sqrt{2}}\) on the interval \([0 , 2\pi)\) are given by
\[ \theta_1 = \pi - \theta_r = \pi - \dfrac{\pi}{4} = \dfrac{3\pi}{4}\]
\[ \theta_2 = \pi + \theta_r = \pi + \dfrac{\pi}{4} = \dfrac{5\pi}{4}\]
We now write the general solutions by adding multiples of \( 2\pi \) as follows:
\[
\theta_1 = \dfrac{3\pi}{4} + 2n\pi, \quad n = 0, \pm 1, \pm 2, \ldots
\]
\[
\theta_2 = \dfrac{5\pi}{4} + 2n\pi, \quad n = 0, \pm 1, \pm 2, \ldots
\]
We now substitute \( \theta_1 \) and \( \theta_2 \) by the expression \( 3x + \dfrac{\pi}{4} \)
\[
3x + \dfrac{\pi}{4} = \dfrac{3\pi}{4} + 2n\pi
\]
\[
3x + \dfrac{\pi}{4} = \dfrac{5\pi}{4} + 2n\pi
\]
and solve for \( x \) to obtain the solutions for \( x \).
\[
x_1 = \dfrac{\pi}{6} + \dfrac{2n\pi}{3}, \quad n = 0, \pm 1, \pm 2, \ldots
\]
\[
x_2 = \dfrac{\pi}{3} + \dfrac{2n\pi}{3}, \quad n = 0, \pm 1, \pm 2, \ldots
\]
Example 4
Solve the trigonometric equation \[ - 2 \sin^2 x - \cos x = - 1 \]
Solution to Example 4
The above equation may be factored if all trigonometric functions included in that equation are the same. So using the identity \( \sin^2 x = 1 - cos^2 x \), we can rewrite the above equation using the same trigonometric function cos x as follows:
\[ - 2 (1 - \cos^2) - \cos x = - 1 \]
Simplify and rewrite as
\[ 2 \cos^{2} x - \cos x - 1 = 0 \]
Factor the left hand side
\[ (2 \cos x + 1)(\cos x - 1) = 0 \]
Hence the two equations to solve
\[ (1) \quad 2 \cos x + 1 = 0 \quad \text{and} \quad (2) \quad \cos x - 1 = 0 \]
Solve equation (1) using the reference angle as was done in the examples above.
\[ \cos x = -\dfrac{1}{2} \]
\[ x_{1} = \dfrac{2\pi}{3} + 2n\pi , \quad n = 0, \pm 1 , \pm 2, \ldots \]
\[ x_{2} = \dfrac{4\pi}{3} + 2n\pi , \quad n = 0, \pm 1 , \pm 2, \ldots \]
Solve equation (2)
\[ \cos x = 1 \]
\[ x_{3} = 2n\pi , \quad n = 0, \pm 1 , \pm 2, \ldots \]
More References and links