Grade 12 Math Practice Test
Grade 12 math practice test questions are presented along with their solutions on videos.
-
Solve the inequality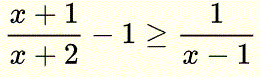
and present the solution set using intervals, number line and inequality symbols.
Solution on video at rational inequality, question 1
-
Solve the equation
Solution on video at trigonometric equation, question 2
-
Solve the equation
Solution on video at equation with logarithms, question 3
-
Solve the equation
Solution on video at equation with exponentials, question 4
-
Verify the identity :
Solution on video at verify trigonometric identities, question 5
\( \)\( \)\( \)\( \) -
Find the exact value of : \( \displaystyle \quad \tan \left(\frac{13\pi}{12}\right) \)
Solution on video at find exact value of trigonometric function, question 6
-
When polynomial \( P(x) \) is divided by \( x + 1 \), the remainder is equal to \( 4 \) and when \( P(x) \) is divided by \( x - 2 \) it gives a remainder equal to \( 4 \). Polynomial \( p(x) \) has degree \( 3 \) and has \( x - 1 \) as a factor. The leading coefficient of \( P(x) \) is equal to \( 1 \). Find \( P(x) \)
Solution on video at find polynomial given remainders and a factor, question 7
-
Function \( f \) is defined by \( f(x) = - x^4 - 5x^3 - 3x^2+9x \)
a) Factor \( f(x) \) completely.
b) Use the zeros to sketch the graph of \( f \).
Solution on video at Factor completely and sketch a polynomial, question 8
-
Find the equation of the polynomial function \( g \) whose degree is equal to \( 4 \) and whose graph is shown below and touches (does not cut) the x-axis at \( x = -1 \).
Solution on video at Find the equation of a polynomial given its graph, question 9
-
For the function \( y = - 0.5 \sin \left( 4(x+\frac{\pi}{16}) \right) + 2.5 \), make a table of values over 1 period and sketch the graph over 2 periods.
Solution on video at make a table of values and sketch , question 10
-
The velocity \( V \) in meters ( \( m \) ) of an object is given by the graph below. Write \( V \) as a function of time \( t \) in seconds ( \( s \) ) as a cosine function.
Solution on video at find an equation to a trigonometric equation given by its graph , question 11
-
Given the function \[ y = \frac{2 x - 4}{x+2} \]
a) Find the domain of the function
b) Find the x and y intercepts of the graph of the function
c) Find the equations of all the asymptotes of the function and any intercepts with the graph of the function
d) Make a table of signs and sketch the graph of the function
Solution on video at sketch the graph of the rational function y = (2x - 4) / (x + 2) , question 12
-
Given the function \[ y = \frac{x^2-9}{x+2} \]
a) Find the domain of the function
b) Find the x and y intercepts of the graph of the function
c) Find the equations of all the asymptotes of the function
d) Make a table of signs and sketch the graph of the function
-
Find the equation of the rational function \( h(x) \) whose graph is shown below and whose denominator has a polynomial of degree 2.
AN: (2x-4)/[(x-1)(x-2)] with hole
-
Given the function \( f(x) = -0.5 \log_2(x^2 - 1)-1 \)
a) Find the domain of the function
b) Find the x and y intercepts, if any, of the graph of the function
c) Find the equations of all the asymptotes, if any, of the function
d) Make a table of values and sketch the graph of the function
-
Given the function \( h(x) = 2 + e^{(x-2)} \)
a) Find the domain of the function
b) Find the x and y intercepts, if any, of the graph of the function
c) Find the equations of all the asymptotes, if any, of the function
d) Make a table of values and sketch the graph of the function
-
Given the function \( h(x) = \ln (2x - 1) + 2 \)
a) Find the domain and range of function \( h \).
b) Find the inverse of function \( h \) and specify its domain and range.
More References and links
