Grade 7 Math Practice Test

Online grade 7 math practice test questions are presented along with their solutions .
Note that no calculator is to be used except for questions 35,36 and 55.

1 - Integers

1. Which of the following statements is always true?
   a) The absolute value of a negative integer is a negative integer.
   b) The absolute value of a negative integer is a positive integer.
   c) The absolute value of a positive integer is a negative integer.
   d) The absolute value of an integer may be negative, positive or equal to zero.
   
   
2. Which of the following statements is true?
   
   
   
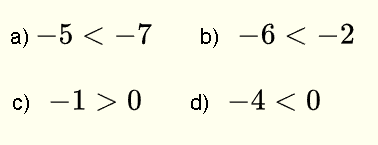
3. Evaluate the following expressions
   

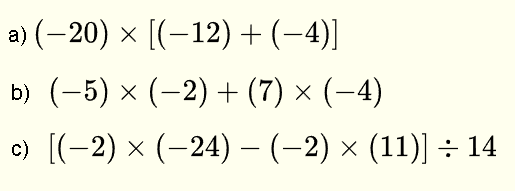
   2 - Decimals

4. Order the numbers from largest to smallest.
   
   
   
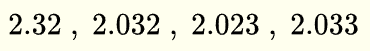
5. Round to the nearest whole number
   
   
   
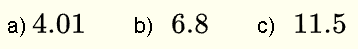
6. Evaluate the following expressions
   
   \( \)\( \)\( \)

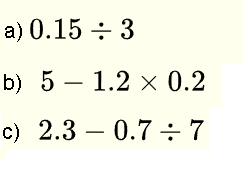   3 - Factors, Multiples and Divisibility of Numbers

7. What is the Greatest Common Factor (GCF) of \( 24 \) and \( 18 \) ?
   
8. What is the Least Common Multiple (LCM) of \( 8 \) and \( 18 \) ?
   
9. Which of the following numbers is divisible by \( 5 \) ?
   a)   \( 1234 \)        b)   \( 303090 \)        c)   \( 145055 \)
   
10. Which of the following numbers is divisible by \( 2 \) ?
    a)   \( 2798 \)        b)   \( 30675 \)        c)   \( 6476 \)
    
11.   Which of the following numbers is divisible by \( 3 \) ?
    a)   \( 9240 \)        b)   \( 4 909 \)        c)   \( 3 282 900 \)

    4 - Fractions and Mixed Numbers

12. Find the missing numerator or denominator that makes each of the following pairs of fractions equivalent.
    a)   \( \displaystyle \frac{10}{15} = \frac{?}{3} \)        b)   \( \displaystyle \frac{17}{3} = \frac{34}{?} \)        c)   \( \displaystyle \frac{11}{2} = \frac{?}{8} \)
    
    
13. Evaluate the following expressions
    a)   \( \displaystyle \frac{2}{5} + \frac{3}{10} - \frac{1}{10}\)        b)   \( \displaystyle \frac{5}{9} \times \frac{3}{4} \)        c)   \( \displaystyle \frac{11}{2} \div \frac{1}{8} \)
    
    d)   \( \displaystyle 4 \frac{3}{4} - 1 \frac{1}{2} \)        e)   \( \displaystyle 6 \frac{3}{4} \div 2 \)        f)   \( \displaystyle 3 \div \frac{3}{5} \)        g)   \( \displaystyle 2 \frac{3}{5} \div 3 \frac{3}{5} \)
    
    
14. Write the decimals as fractions or mixed numbers in reduced form
    a)   \( 0.2 \)        b)   \( 1.24 \)        c)   \( 2.326 \)
    
    
15. Write as a decimal
    a)   \( \displaystyle \frac{9}{100} \)        b)   \( \displaystyle \frac{17}{10000} \)        c)   \( \displaystyle 3 \frac{11}{100000} \)
    
    
16. Which of the following is true ?
    a)   \( \displaystyle \frac{2}{5} \lt \frac{3}{4} \)        b)   \( \displaystyle \frac{1}{3} \lt \frac{3}{10} \)

    5 - Exponents

17. Simplify the following expressions using exponents.
    a)   \( 3 \times 3 \times 3 \times 3 \)        b)   \( 7 \times 4 \times 4 \times 4 \times 5 \times 5 \)
    
18. Evaluate the following expressions
    a)   \( 2^4 \)        b)   \( 3^2 \times 4^2 \)        c)   \( 10^0 \times 4^2 \)

    6 - Ratios and Rates and Related

19.   There are \( 4 \) triangles and \( 7 \) squares. What is the ratio of
    a)   triangles to squares?        b)   squares to triangles?        c)   squares to total numbers of figures?
    
20.   School A has \( 1200 \) students including \( 400 \) boys. School B has \( 800 \) students including \( 300 \) boys. Which school has a higher ratio girls to boys?
    
21.   Sam bought \( 5 \) kilograms of tomatoes at the cost of \( \$15 \). Find the unit rate (or price) in dollars / kilogram.
    
22.   A car traveled \( 350 \) kilometers (km) in \( 5 \) hours (hrs). Find the unit rate in km / hrs.

    7 - Proportionality and Related Problems

23.   A car travels \(240\) kilometers in \( 3 \) hours at a constant speed. How many hours are needed to travel \( 400 \) kilometers in the same car at the same constant speed ?
    
24.   The money used in the UAE is called the Dirham with an exchange rate of 4 Dirhams to 1 US dollar. How many US Dollars are needed to buy 320 Dirhams at the given rate ?
    
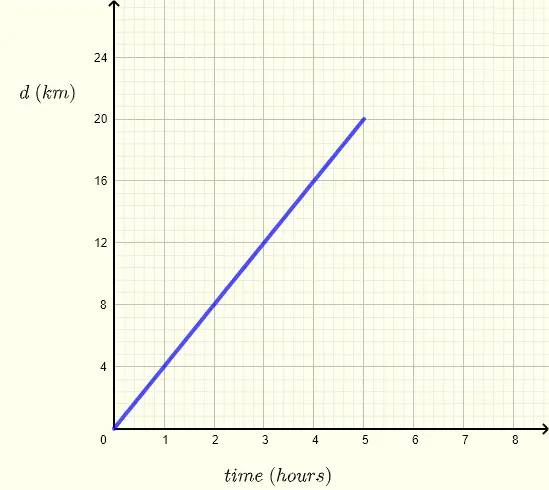
25.   Joan went for a 5 hours walk and and the graph below shows the distance (in km) walked after time (in hours).
    a)   Assuming that the distance walked is proportional to the time, what distance did she walk for the first 2 and half hours ?
    b)  What the walking speed (rate) of Joan ?
    c)   Two weeks later, she decided to go for a longer walk at the same rate. How many hours were needed to cover 32 kilometers ?
    
    
    
    
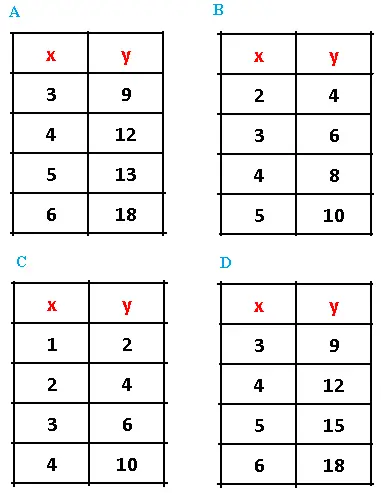
26.   Which of the following tables indicates that \( y \) is proportinal to \( x \) ?
    

    8 - Percent and Related Problems

27. What is \( 20\% \) of \( 10 \)?
    
28. What is \( 50\% \) of \( \displaystyle \frac{1}{4} \)?
    
29. Write the fraction \( \displaystyle \frac {3}{5} \) as a percentage.
    
30. Amanda has a monthly salary of \( $3000 \). She spends \( $600 \) per month on clothes. What percent of her monthly salary does Amanda spend on clothes ?
31. The price of an item changed from \( $120 \) to \( $100 \). What was the change in percent ?
32. The \( 10\% \) of a number is equal to 3. What is the number ?
33. A shirt initially costs $40. The price of the shirt is increased by 20% then the price of the same shirt is decreased by 20% (from the price after the increase). What is the final price of the shirt?

    9 - Convert Units of Measurement

34. How many many meters (m) are in \( 1.2 \) kilometers (km) knowing that \( 1 \text{ km} = 1000 \text{ m} \)?
    
35. How many US gallons (US gal) are in 120 liters (L) knowing that \( 1 \text{ US gal} = 3.78541 \text{ L} \) ?
    
36. How many square feet are in \( 0.3 \) square meters ( \( m^2 \) ) knowing that \( 1 \; m = 3.28084 \; ft \) ?
    
37. Convert the rate of \( 60 \) kilometers per hour into meters per minute ?

    10 - Evaluate Expressions

38. Evaluate the expression \( \; 2x - 2 \; \) for \( x = -2 \)
    
39. Evaluate the expression \( \; | -5 + b | \; \) for \( b = -10 \)
    
40. Evaluate the expression \( \; a - b \; \) for \( a = -5 \) and \( b = -8 \)

    11 - Algebra

41. Simplify the expressions
    a)   \( 3x - 2 + 4 x - 5 \)        b)   \( 3 (a + b + 2) + a + 4b - 12 \)        c)   \( \displaystyle \frac{1}{3}( 6 x + 9) + 3 \)        d)   \( 0.2 x + x \)

42.  Factor the expressions
    a)   \( 14 x - 2 \)        b)   \( 9 - 18 x \)        c)   \( 4 b - 16 a + 4 \)

    12 - Equation with One Variable and Related Problems

43.  Solve the equations
    a)   \( 3x - 2 = 4 \)        b)   \( 9 - 3 = - x + 5 \)       
    c)   \( \displaystyle \frac{x}{3} = - 7 \)        d)   \( 4 \left(x + \displaystyle \frac{1}{4} \right) = -15\)
    e)   \( \displaystyle \frac{x+2}{-3} = 3 \)        f)   \( 2(x-1) = 3(x+2)\)        g)   \( x - 2\displaystyle \frac{1}{4} = 3 \)
    
44. The perimeter of a rectangular garden is 340 m and its length is 120 m. Let \( x \) be the width of the garden.
    a) Write an equation in \( x \) to solve for the width.
    b) Solve the equation obtained in part a)
    c) Check your answer to the problem.

    13 - Inequality with One Variable

45.  Represent the inequalities on a number line
    a)   \( x \lt 6 \)        b)   \( x \ge 2 \)      c)   \( x \lt -4 \) or \( x \ge 0 \)
    
46.  Solve the inequalities
    a)   \( 4x - 2 \gt 18 \)        b)   \( 2(x - 1) \gt 6 \)

    14 - Two-Dimensional Figures

47.   A triangle has two angles that measure 36? and 54?. Which of the following is true
    a)   This is an isosceles triangle.        b)   This is a right triangle.        b)   This is an equilateral triangle.
    
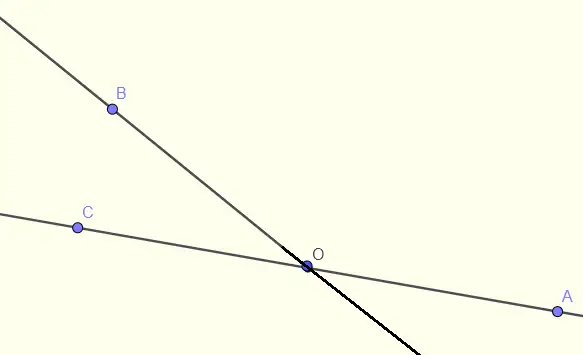
48.   Two straight lines intersect at point \( O \). What is the size of angle \( \angle AOB \) if the size of angle \( \angle COB \) is equal to \( 27^{\circ} \)
    
    
    
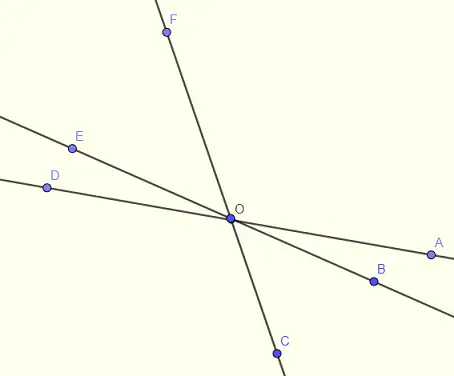
49.   Three straight lines intersect at point \( O \). List all the pairs of vertical angles in the figure below.
    
    
    
50.   Give the number of sides of each of the polygons listed below
    a)   Hexagon        b)   Pentagon        c)   Octagon
    
51.   How many lines of symmetry an equilateral triangle has ?

    15 - Perimeter and Area of Planar Figures

52.   Calculate the area of a circle with a diameter of \( 20 \) cm.
53.   Calculate the perimeter of a rectangle with a length of \( 10 \) inches and a width of \( 8 \) inches.
54.   Calculate the area of a triangle of height \( 10 \) cm and base \( 5 \) cm.
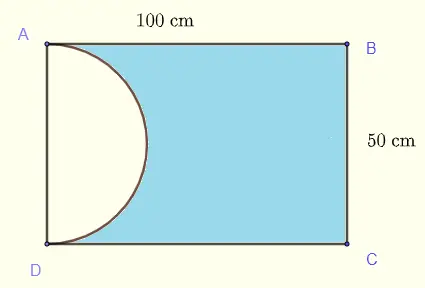
55.   ABCD is a rectangle bounded on the left side by a semicircle. Find the area of the shaded (in blue) surface.
    
    

    16 - Data and Interpretation of Graphs

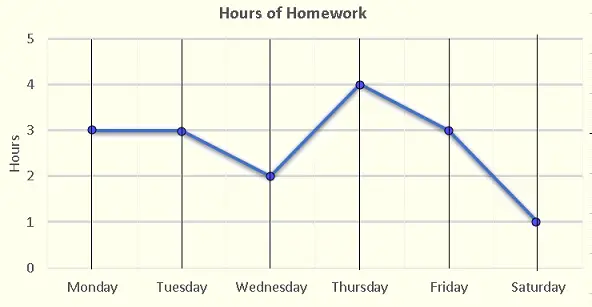
56. The line plot below shows the number of hours spent on homework by Mathew for 6 days preparing for his exam.
    a) On which day did Mathew spend the smallest number of hours on homework ?
    b) On which day did Mathew spend the largest number of hours on homework ?
    c) How many hours did Mathew spend on homework preparing for his exam?
    
    
    
57. The histogram below shows the range of scores (horizontal axis) and the number of students (vertical axis) who scored on that range for a class on a math test.
    a) How many students are in this class?
    b) How many students scored between 70 and 89 inclusive?
    c) Anyone who scored less than 60 is supposed to have failed. What percent of the total number of students failed the test ?
    
    

    17 - Statistics

58. Calculate the mean, mode and median of the data set: \( \{ 9 , 4 , 3 , 2 , 3 , 2 , 3 , 1 , 9 \} \)
    
59. Joel scored \( 78, 95\) and \( 92 \) on her first 3 quizzes. What should be the score on her fourth quiz so that the average of the 4 quizzes is 90 ?

    18 - Counting Principle

60. At a restaurant, lunch is offered with a choice of three salads, five main dishes and four desserts. In how many ways can one order his lunch ?
    
61. There are two car dealerships in town. The first one has 3 body styles, 4 colors and 3 models. The second one has 2 body styles, 5 colors and 4 models. Which car dealership has more choices ?

    19 - Probabilities

62.   Which of the following cannot be a measure of probability ?
      a) 1       b) -0.5     c) 2     d) 0     e) 0.0001
    
63.   How many outcomes are possible if you flip a coin and select one of five different cards at random ?
    
64.   A die, with numbers from 1 to 6 on the faces, is rolled. What is the probability that the number obtained is
    a) equal to 0 ?
    b) equal to 5 ?
    c) greater than 4 ?
    
65.   Linda surveyed 20 students in her school about their favorite color and 5 said blue was their favorite color. What is the probability that the next student surveyed will pick a color that is not blue ?
    

More References and links

1. Grade 7 Math Topics
2. Fractions Questions and Problems with Solutions