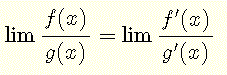
where \( \lim \) stands for \( \lim_{{x \to a}}, \lim_{{x \to a^+}}, \lim_{{x \to a^-}}, \lim_{{x \to +\infty}}, \) or \( \lim_{{x \to -\infty}} \).
\( f'(x) \) and \( g'(x) \) are the derivatives of \( f(x) \) and \( g(x) \) respectively.
L'Hopital's theorem allows us to replace a limit problem with another that may be simpler to solve. Several examples are presented along with their solutions and detailed explanations.
If \( \lim_{{x \to a}} f(x) = 0 \) and \( \lim_{{x \to a}} g(x) = 0 \) and if \( \lim_{{x \to a}} \dfrac{{f'(x)}}{{g'(x)}} \) has a finite value \( L \), or is of the form \( +\infty \) or \( -\infty \), then
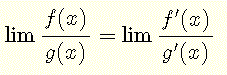
where \( \lim \) stands for \( \lim_{{x \to a}}, \lim_{{x \to a^+}}, \lim_{{x \to a^-}}, \lim_{{x \to +\infty}}, \) or \( \lim_{{x \to -\infty}} \).
\( f'(x) \) and \( g'(x) \) are the derivatives of \( f(x) \) and \( g(x) \) respectively.
Find the limit \( \lim_{{x \to 0}} \dfrac{{\sin x}}{{x}} \)
Solution to Example 1:
Since
\( \lim_{{x \to 0}} \sin x = 0 \)
and
\( \lim_{{x \to 0}} x = 0 \)
L'Hopital's rule can be used to evaluate the above limit as follows
\( \lim_{{x \to 0}} \dfrac{{\sin x}}{{x}} = \lim_{{x \to 0}} \dfrac{\dfrac{{d(\sin x)}}{{dx}}} {\dfrac{{d(x)}}{{dx}} } \)
\( = \lim_{{x \to 0}} \dfrac{{\cos x}}{{1}} = \dfrac{{\cos 0}}{{1}} = 1 \)
Find the limit \( \lim_{{x \to 0}} \dfrac{{e^x - 1}}{{x}} \)
Solution to Example 2:
Note that
\( \lim_{{x \to 0}} (e^x - 1) = 0 \)
and
\( \lim_{{x \to 0}} x = 0 \)
We can use L'Hopital's rule to calculate the given limit as follows
\( \lim_{{x \to 0}} \dfrac{{e^x - 1}}{{x}} = \lim_{{x \to 0}} \dfrac{\dfrac{{d(e^x - 1)}}{{dx}}} { \dfrac{{d(x)}}{{dx}}} \)
\( = \lim_{{x \to 0}} \dfrac{{e^x}}{{1}} = \dfrac{{e^0}}{{1}} = 1 \)
Find the limit \( \lim_{{x \to 1}} \dfrac{{x^2 - 1}}{{x - 1}} \)
Solution to Example 3:
Since the limit of the numerator
\( \lim_{{x \to 1}} (x^2 - 1) = 0 \)
and that of the denominator
\( \lim_{{x \to 1}} (x - 1) = 0 \)
are both equal to zero, we can use L'Hopital's rule to calculate the limit
\( \lim_{{x \to 1}} \dfrac{{x^2 - 1}}{{x - 1}} = \lim_{{x \to 1}} \dfrac{\dfrac{{d(x^2 - 1)}}{{dx}}}{ \dfrac{{d(x - 1)}}{{dx}}} \)
\( = \lim_{{x \to 1}} \dfrac{{2x}}{{1}} = \dfrac{{2(1)}}{{1}} = 2 \)
Note that the same limit may be calculated by first factoring as follows
\( \lim_{{x \to 1}} \dfrac{{x^2 - 1}}{{x - 1}} = \lim_{{x \to 1}} \dfrac{{(x - 1)(x + 1)}}{{x - 1}} \)
\( = \lim_{{x
\to 1}} (x + 1) = 2 \)
Find the limit \( \lim_{{x \to 2}} \dfrac{{\ln(x - 1)}}{{x - 2}} \)
Solution to Example 4:
Limit of numerator
\( \lim_{{x \to 2}} \ln(x - 1) = 0 \)
Limit of denominator
\( \lim_{{x \to 2}} (x - 1) = 0 \)
Both limits are equal to zero, L'Hopital's rule may be used
\( \lim_{{x \to 2}} \dfrac{{\ln(x - 1)}}{{x - 2}} = \lim_{{x \to 2}} \dfrac{\dfrac{{d(\ln(x - 1))}}{{dx}}} { \dfrac{{d(x - 2)}}{{dx}} } \)
\( = \lim_{{x \to 2}} \dfrac{{1/(x-1)}}{{1}} = \dfrac{{1/(2 -1)}}{{1}} = 1 \)
Find the limit \( \lim_{{x \to 0}} \dfrac{{1 - \cos x}}{{6x^2}} \)
Solution to Example 5:
Limit of numerator and denominator
\( \lim_{{x \to 0}} (1 - \cos x) = 0 \)
\( \lim_{{x \to 0}} 6x^2 = 0 \)
L'Hopital's rule may be used
\( \lim_{{x \to 0}} \dfrac{{1 - \cos x}}{{6x^2}} = \lim_{{x \to 0}} \dfrac{{\sin x}}{{12x}} \)
The new limit is also indeterminate \( \dfrac{0}{0} \) and we may apply L'Hopital's theorem a second time
\( = \lim_{{x \to 0}} \dfrac{{\cos x}}{{12}} = \dfrac{{\cos 0}}{{12}} = \dfrac{1}{12} \)
Find the limits
1. \( \lim_{{x \to 0}} \dfrac{{\sin 4x}}{{\sin 2x}} \)
2. \( \lim_{{x \to 0}} \dfrac{{\tan x}}{{x}} \)
3. \( \lim_{{x \to 1}} \dfrac{{\ln x}}{{3x - 3}} \)
4. \( \lim_{{x \to 0}} \dfrac{{e^x - 1}}{{\sin 2x}} \)
1. \( 2 \)
2. \( 1 \)
3. \( \dfrac{1}{3} \)
4. \( \dfrac{1}{2} \)