A Level Maths Practice Questions with Solutions - Sample 1

Question 1
A circle has the equation \( x^2-4x+y^2+6y = 12 \) and center \( C \).
a) Find the area of the circle.
b) Find the coordinates of the center \( C \) of the circle.
c) Point \( A(6,-6) \) is on the circle. Find the coordinates of point \( B \) such that \( AB \) is a diameter of circle.
Solution
a)
We first write the equation of the given circle in standard form \( (x-h)^2 + (y-k)^2 = r^2 \) with center at the point \( (h,k) \) and radius \( r \).
Use completing the square for the terms in \( x \) and the terms in \( y \) in the given equation as follows
\( (x-2)^2 - 4 + (y+3)^2 - 9 = 12 \)
Write in standard form leaving the terms with the square on the left side
\( (x-2)^2 + (y+3)^2 = 25 \)         (I)
Compare the above equation to the general standard equation \( (x-h)^2 + (y-k)^2 = r^2 \) to determine the radius \( r \)
\( r^2 = 25 \)
which gives
\( r = 5 \)
Area of circle = \( \pi r^2 = 25 \pi \) square units
b)
Compare equation (I) to the general standard equation given above to determine the center at \( (h,k) \)
\( h = 2 \) and \( k = - 3 \)
The center \( C \) of the circle is at \( (2,-3) \)
c)
Let \( (x_0 , y_0) \) be the coordinates of point \( B \). The center \( C \) of the circle is also the center of segment (diameter) \( AB \).
The formula of the coordinates of a center of a segment gives the equations:
\( \dfrac{x_0+6}{2} = 2\) and \( \dfrac{y_0+(-6)}{2} = -3\)
Solve the above equations to obtain
\( x_0 = -2 \) and \( y_0 = 0 \)
Point \( B \) such that \( AB \) is a diameter has the coordinates
\( (x_0 , y_0) = (-2,0) \)
Below is shown the graph of the given circle, points \( A \) and \( B \) and center \( C \) in order to check the calculations above.

Question 2
Prove that \( \dfrac{ 2 \cos x + \sin (2x)}{2\sin x + 2 \sin^2 x} = \cot x \)
Solution
We start with the left hand side of the given identity and show that it is equal to the right side using known identities.
Use the double angle trigonometric identity \( \sin (2x) = 2 \sin x \cos x \) in the numerator to rewrite the left hand side of the given identity as
\( \dfrac{ 2 \cos x + \sin (2x)}{2\sin x + 2 \sin^2 x} = \dfrac{ 2 \cos x + 2 \sin x \cos x}{2\sin x + 2 \sin^2 x} \)
Factor out \( 2 \cos x \) in the numerator and \( 2 \sin x \) in the denominator and rewrite the above as
\( = \dfrac{ 2 \cos x (1 + \sin x)}{2\sin x ( 1 + \sin x)}\)
Divide numerator and denominator by \( 1 + \sin x \) and simplify to obtain
\( = \dfrac{ \cos x } {\sin x} = \cot x\)

Question 3
The graph of \( f \) given by \( f(x) = x^3-2x^2-5x+6 \) intersects the x axis at \( x = 1 \)
a) Write \( f(x) \) as a product of linear factors.
b) Solve the equation \( x^3-2x^2-5x + 6 = 0 \).
c) Use the results of part b) to solve the equation \( e^{-6x} - 2 e^{-4x} - \dfrac{5}{e^{2x}} + 6 = 0 \).
Solution
a)
Since the graph of \( f \) intersects the \(x\)-axis at \( x = 1 \), \( x - 1 \) is a factor of \( f(x) \) which may be written as
\( f(x) = (x - 1) Q(x) \)
where
\( Q(x) = \dfrac{f(x)}{x - 1} = \dfrac{x^3-2x^2-5x+6}{x-1} \)
Use polynomial division to \( \dfrac{x^3-2x^2-5x+6}{x-1} \) and obtain
\( Q(x) = x^2 - x -6 \)
which may be factored as
\( Q(x) = (x-3)(x+2) \)
Hence \( f(x) \) as the product of linear factors is given by
\( f(x) = (x - 1) Q(x) = (x-1) (x-3)(x+2) \)
b)
The left hand side of the equation \( x^3-2x^2-5x + 6 \) is equal to \( f(x) \) which has been factored above. Hence the equation to solve may be written in factored form as
\( (x-1) (x-3)(x+2) = 0 \)
The solutions of the above equation are found by setting each factor equal to zero and then solving it which gives the solutions:
\( x = 1 \) , \( x = 3 \) and \( x = -2 \).
c)
Let \( z = e^{-2x} \) which gives \( z^2 = (e^{-2x})^2 = e^{-4x} \) and \( z^3 = (e^{-2x})^3 = e^{-6x} \)
Substitute the above in the given equation \( e^{-6x} - 2 e^{-4x} - \dfrac{5}{e^{2x}} + 6 = 0 \) to obtain the equation in z:
\( z^3 - 2 z^2 - 5 z + 6 = 0 \)
The above equation has been solved in part b) above, hence the solutions
\( z = 1 = e^{-2x} \) which gives the solution \( x = 0 \)
\( z = 3 = e^{-2x} \) which gives the solution \( x = - \dfrac{\ln 3}{2} \approx -0.54930 \)
\( z = - 2 = e^{-2x} \) which has no real solution in \( x \) since \( e^{-2x} \) is always positive.
The solution set of the given equation is: \( \left\{ 0, - \dfrac{\ln 3}{2} \right\} \)
Below is shown the graph of the right hand side of the equation in part c) and the \( x \) intercepts whose \( x \) coordinates are the solution to the given equation.

Question 4
a) Solve the equation \( x^2 + \dfrac{\sqrt 2 - 1}{2} x - \dfrac{\sqrt 2}{4} = 0 \)
b) Prove that \( \cos (2 x) + 1 = 2 - 2 \sin^2 x \)
c) Use the results of parts a) and b) to find the solutions of the equation \( 4 - 2 \left( \cos (2 x) + 1\right) + 2(\sqrt 2 - 1) \sin x - \sqrt 2 = 0 \) over the interval \( [0, 2\pi) \).
Solution
a)
Factor the left side of the given equation
\( \left( x - \dfrac{1}{2} \right) \left(x + \dfrac{\sqrt 2}{2} \right) = 0 \)
Solve
\( x_1 = \dfrac{1}{2}\)
\( x_2 = -\dfrac{\sqrt 2}{2} \)
b)
Write \( \cos (2 x) \) as \( \cos (x + x) \) in the left hand side of the given identity
\( \cos (2 x) + 1 = \cos(x + x) + 1 \)
Expand \( \cos(x + x) \) using the trigonometric identity \( \cos ( x + y ) = \cos x \cos y - \sin x \sin y \)
\( = \cos^2 x - \sin^2 x + 1 \)
Use the identity \( \cos^2 x = 1 - \sin^2 x \)
\( = 1 - \sin^2 x - \sin^2 x + 1 \)
Simplify
\( = 2 - 2 \sin^2 x \)
c)
Substitute \( \cos (2 x) + 1 \) in the given equation by \( 2 - 2 \sin^2 x \) as proved above
\( 4 - 2 \left( 2 - 2 \sin^2 x \right) + 2(\sqrt 2 - 1) \sin x - \sqrt 2 = 0 \)
Group and simplify the left hand side in the above
\( 4 \sin^2 x + 2(\sqrt 2 - 1) \sin x - \sqrt 2 = 0 \)
Divide all terms in the above equation by \( 4 \)
\( \sin^2 x + \dfrac{\sqrt 2 - 1}{2} \sin x - \dfrac{\sqrt 2}{2} = 0 \)
Let \( z = \sin x \) and substitute in the above equation to obtain an equation in \( z \)
\( z^2 + \dfrac{\sqrt 2 - 1}{2} z - \dfrac{\sqrt 2}{2} = 0 \)
The above equation has already been solved in part a) and has the solutions
\( z_1 = \dfrac{1}{2}\) and \( z_2 = -\dfrac{\sqrt 2}{2} \)
Substitute \( z_1 \) and \( z_2 \) by \( \sin x \) and solve both equations for \( x \) in the interval \( [0, 2\pi) \)
\( z_1 = \dfrac{1}{2} = \sin x\) gives the solutions : \( x_1 = \dfrac{\pi}{6} \) and \( x_2 = \dfrac{ 5 \pi}{6} \)
\( z_2 = -\dfrac{\sqrt 2}{2} = \sin x\) gives the solutions : \( x_3 = \dfrac{5\pi}{4} \) and \( x_4 = \dfrac{ 7 \pi}{4} \)
The solution set of the given equation is: \( \left\{ \dfrac{\pi}{6} , \dfrac{ 5 \pi}{6} , \dfrac{5\pi}{4} , \dfrac{ 7 \pi}{4} \right\} \)
Below is shown the graph of the left side of the equation with the x-intercepts in the interval \( [0, 2\pi) \) corresponding to the solutions found analytically above.

Question 5
a) Sketch the graph of \( f(x) = \sin (2x) \) over the interval \( [0 , \pi ] \)
b) Solve the equation \( \sin(2x) = \dfrac{1}{2} \) over the interval \( [0 , \pi ) \)
c) Use the results of parts a) and b) to find the solution set to the inequality \( \dfrac{1}{2} + \sin(2x) \ge 1 \) over the interval \( [0 , \pi) \).
Solution
a)
The period of \( f(x) = \sin (2x) \) is equal to \( 2\pi/2 = \pi \) The five key points are included in the table blow

Question 6
Function \( f \) has zeros at \( x = 1 \) and \( x = - 3 \). Find the zeros of \( f(-2x^2 +x+ 3) \)
Solution
Since \( x = 1 \) is a zero of \( f \), then \( f(1) = 0 \) and therefore \( f(-2x^2 +x+ 3) = 0 \) for \( -2x^2 +x+ 3 = 1 \)
Solve the above equation to obtain the solutions
\( x_1 = \dfrac{-1+\sqrt{17}}{4} \) and \( x_2 =\dfrac{1+\sqrt{17}}{4} \)
Since \( f(-3) = 0 \), then \( f(-2x^2 +x+ 3) = 0 \) for \( -2x^2 +x+ 3 = -3 \)
Solve the above equation to obtain the solutions
\( x_3 = - \dfrac{3}{2} \) and \( x_4 = 2 \)
The zeros of \( f(-2x^2 +x+ 3) \) are: \( - \dfrac{3}{2} , 2 , \dfrac{-1+\sqrt{17}}{4} , \dfrac{1+\sqrt{17}}{4} \)

Question 7
Function \( f \) is given by \( f(x) = x^4+a x^3+b x^2 + 4 \).
a) Find the constants \( a \) and \( b \) so that the graph of \( f \) intersects the x-axis at \( x = 1 \) and \( x = - 1\).
b) Write \( f(x) \) as the product of four linear terms and graph \( f \)
Solution
a)
Graph of \( f \) intersects the x-axis at \( x = 1 \) gives the equation
\( f(1) = (1)^4+a (1)^3+b (1)^2 + 4 = 0\)
Graph of \( f \) intersects the x-axis at \( x = - 1 \) gives the equation
\( f(-1) = (-1)^4+a (-1)^3+b (-1)^2 + 4 = 0\)
The above give a system of two equations in \( a \) and \( b \) as follows:
\( a + b + 5 = 0 \) and \( -a + b + 5 = 0 \)
Solve the above system to obtain
\( a = 0\) and \( b = - 5 \)
b)
Substitute \( a \) and \( b \) by their values to obtain
\( f(x) = x^4 - 5 x^2 +4 \)
Since \( x = 1 \) and \( x = - 1\) are x-intercepts, \( x - 1 \) and \( x + 1 \) are factors of \( f(x) \). Hence
\( f(x) = (x-1)(x+1) Q(x) \)
\( Q(x) \) is obtained by division
\( Q(x) = \dfrac{f(x)}{(x-1)(x+1)} = \dfrac{x^4 - 5 x^2 +4}{x^2 - 1} \)
Use polynomial division to obtain
\( Q(x) = x^2 - 4 = (x-2)(x+2) \)
Hence
\( f(x) = (x-1)(x+1) (x-2)(x+2) \)
Notice that \( f(-x) = (-x)^4 - 5 (-x)^2 +4 = x^4 - 5 x^2 +4 = f(x) \) ; hence \( f \) is an even function and its graph is symmetric with respect to the y-axis.
The graph of \( f \) has 4 x-intercepts at: \( x = 1, -1,2,-2 \)
The y-intercept of the graph of \( f \) is at \( (0,4) \)
Function \( f \) is a polynomial degree of 4 with positive leading coefficient and therefore the graph of \( f \) rises on the left and on the right.
The 4 x-intercepts split the number line into 5 intervals with different signs of the polynomial
Let \( x = -3 \) be a test value on the interval \( (-\infty , - 2) \). \( f(-3) = 40 \), hence \( f(x) \) is positive on the interval \( (-\infty , - 2) \)
Let \( x = -1.5 \) be a test value on the interval \( (- 2 , - 1) \). \( f(-1.5) \approx -2.2 \), hence \( f(x) \) is negative on the interval \( (- 2 , - 1) \)
Let \( x = 0 \) be a test value on the interval \( (- 1 , 1) \). \( f(0) = 4 \), hence \( f(x) \) is positive on the interval \( (- 1 , 1) \)
and by symmetry: \( f(x) \) is negative on the interval \( (1 , 2) \) and positive on the interval \( (2 , \infty) \)
Use all the information above including the intercepts, leading coefficient behavior and signs over the intervals to graph \( f \) as follows

Question 8
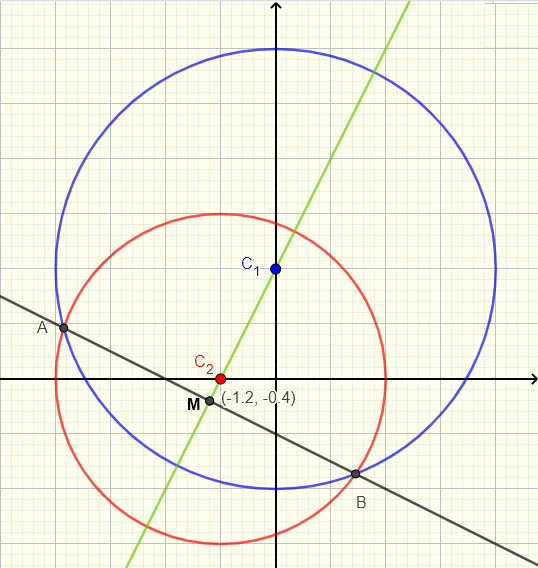
Two circles with centers at \( C_1 \) and \( C_2 \) have the equations \( x^2 + (y-2)^2 = 16 \) and \( (x+1)^2 + y^2 = 9 \) respectively and intersect at the points \( A \) and \( B \).
Find the point of intersection \( M \) of the line through \( A \) and \( B \) and the line through \( C_1 \) and \( C_2 \).
Solution
The points of intersection \( A \) and \( B \) are found by solving the system of equations of the two circles. We first expand the equations of the two circles
\( x^2 + y^2 - 4 y + 4 = 16 \)
\( x^2 + 2x + 1 + y^2 = 9 \)
Subtract the left and righe hand sides of the two equations
\( (x^2 + y^2 - 4 y + 4 ) - (x^2 + 2x + 1 + y^2) = 16 - 9 \)
Simplify to obtain
\( - 2 y - x = 2 \) or \( y = - \dfrac{1}{2} x -1 \)         (I)
The above is a linear equation obtained from the two equations to solve and is therefore the equation of the line through points \( A \) and \( B \).
From the standard equations of the two circles, the center \( C_1 \) is at the point (0,2) and the center \( C_2 \) is at the point (-1,0)
The slope \( m \) of the line through \( C_1 \) and \( C_2 \) is given by
\( m = \dfrac{2-0}{0-(-1)} = 2\)
The equation of the line through \( C_1 \) and \( C_2 \) is given by
\( y - 0 = 2 (x +1) \)         (II) , point slope form using the slope found and the coordinates of point \( C_2 \)
The point of intersection \( M \) of the lines through \( A , B \) and \( C_1 , C_2 \) is found by solving the system of equations made up of the equations (I) and (II) of two lines
\( y = - \dfrac{1}{2} x -1 \) and \( y = 2x + 2 \)
Which gives the solution
\( \left( - \dfrac{6}{5} , -\dfrac{2}{5} \right) \)
Below are shown the graphs of the two circles, line \( A , B \) , line \( C_1 , C_2 \) and their point of intersection \( M \) with its coordinates.

Question 9
Let \( f(x) = \sqrt {x^2-2x-3} \).
a) Write \( x^2-2x-3 \) in the form \( a(x-h)^2 + k\)
b) Find the inverse of function \( g \) defined by \( g(x) = \sqrt {x^2-2x-3} \quad , \quad x \ge 3\)
c) Find the range of the inverse of \( g \) defined above.
Solution
a)
Complete the square of the given expression
\( x^2-2x-3 = (x-1)^2 - 1 - 3 = (x-1)^2 - 4 \)
b)
Let \( y = \sqrt {x^2-2x-3} \)
Substitute the radicand \( x^2-2x-3 \) by \( (x-1)^2 - 4 \)
\( y = \sqrt {(x-1)^2 - 4} \)
Square both sides
\( y^2 = (x-1)^2 - 4 \)
Solve the above for \( x \) to find the two solutions
\( x = 1 \pm \sqrt {y^2 + 4} \)
Since \( x \ge 3\) (see definition of \( g \) above), we select the solution
\( x = 1 + \sqrt {y^2 + 4} \)
The inverse of \( g \) is given by
\( g^{-1}(x) = 1 + \sqrt {x^2 + 4} \)
c)
One of the properties of inverse functions is that range of \( g^{-1} \) is the domain of \( g \) which is given by the interval
\( [3 , \infty) \)

Question 10
A curve has the equation \( -2 x^2 + 3 y^2 - 2 x = 1 \). Find all points where \( \dfrac{dy}{dx} = 0 \)
Solution
Use implicit differentiation on both sides of the given equation with respect to \( x \)
\( \dfrac{d(-2 x^2 + 3 y^2 - 2 x)}{dx} = \dfrac{d( 1 )}{dx} \)
Simplify
\( - 4 x + 6 y \dfrac{dy}{dx}- 2 = 0 \)
Substitute \( \dfrac{dy}{dx} \) by zero in the above equation to obtain
\( - 4 x - 2 = 0 \)
Solve for \( x \)
\( x = - \dfrac{1}{2} \)
Substitute \( x \) by \( - \dfrac{1}{2} \) in the equation of the curve
\( -2 (- \dfrac{1}{2})^2 + 3 y^2 - 2 (- \dfrac{1}{2}) = 1 \)
Solve the above for \( y \)
\( y_1 = \sqrt{\dfrac{1}{6}}\) and \( y_2 = - \sqrt{\dfrac{1}{6}}\)
The points on the curve where \( \dfrac{dy}{dx} = 0 \) are given by
\( \left(- \dfrac{1}{2} , \sqrt{\dfrac{1}{6}} \right) \) and \( \left(- \dfrac{1}{2} , - \sqrt{\dfrac{1}{6}} \right) \)

Question 11
a) Expand the expression \( \sin \left(x-\dfrac{\pi }{3}\right) \) and write it in terms of \( \sin x \) and \( \cos x \)
b) Use the results in part a) to write the equation \( 2 \sin(x) + 3 \cos(x) = \sin\left(x-\frac{\pi }{3}\right) \) in the form \( A \sin x = B \cos x\).
c) Find the solutions of the equation \( 2 \sin(x) + 3 \cos(x) = \sin \left(x-\dfrac{\pi }{3}\right) \) included in the interval \( [0 , 2\pi ) \).
Solution
a)
Use the trigonometric identity \( \sin(A - B) = \sin A \cos B - \cos A \sin B \) to write
\( \sin \left(x-\dfrac{\pi }{3}\right) = \sin x \cos \dfrac{\pi }{3} - \cos x \sin \dfrac{\pi }{3} \)
Simplify the right side
\( \sin \left(x-\dfrac{\pi }{3}\right) = \dfrac{ 1 }{2} \sin x - \dfrac{\sqrt 3 }{2} \cos x \)
b)
Substitute \( \sin \left(x-\dfrac{\pi }{3}\right) \) in the right side of the given equation by the expression found above
\( 2 \sin(x) + 3 \cos(x) = \dfrac{ 1 }{2} \sin x - \dfrac{\sqrt 3 }{2} \cos x \)
Rewrite the above with \( \sin x \) terms on one side and \( \cos x \) terms on the other side.
\( 2 \sin(x) - \dfrac{ 1 }{2} \sin x = - 3 \cos(x) - \dfrac{\sqrt 3 }{2} \cos x \)
Factor and simplify
\( \dfrac{ 3 }{2} \sin x = (- 3 - \dfrac{\sqrt 3 }{2} ) \cos x \)
c)
Divide both sides of the equation by \( \cos x \) and rewrite the above equation as
\( \tan x = \dfrac{- 3 - \dfrac{\sqrt 3 }{2}}{\dfrac{ 3 }{2}} \)
Simplify the right side
\( \tan x = - 2 - \dfrac{\sqrt 3 }{3} \)
Solve the above trigonometric equation
\( x = \arctan \left(- 2 - \dfrac{\sqrt 3 }{3} \right) + n \pi , n = 0 , \pm 1 , \pm 2 , .... \)
\( x \approx - 1.2 + n \pi , n = 0 , \pm 1 , \pm 2 , .... \)
We now need to select values for the integer \( n \) such that the solutions are in the interval \( [0 , 2\pi ) \).
\( n = 1 \) gives the solution \( x \approx - 1.2 + \pi = 1.94 \)
\( n = 2 \) gives the solution \( x \approx - 1.2 + 2\pi = 5.08\)
The graphical solutions of the equation in part c) are shown below.

Question 12
a) Write \( \sin^2 x \) in terms of \( \cos(2x) \)
b) Use the results in part a) and the the rule of integration by parts: \( \displaystyle \int u v' dx = u v - \int u' v dx \) to evaluate the integral \( \displaystyle \int_0^{\pi} x \sin^2 x dx \)
Solution
a)
Write
\( \cos(2x) = \cos (x + x) \)
Expand the right side using the trigonometric identity \( \cos ( A + B ) = \cos A \cos B - \sin A \sin B \)
\( \cos(2x) = \cos^2 x - \sin^2 x \)
Use identity \( \cos^2 x = 1 - \sin^2 x \)
\( \cos(2x) = 1 - \sin^2 x - \sin^2 x \)
Group
\( \cos(2x) = 1 - 2 \sin^2 x\)
Hence
\( \sin^2 x = \dfrac {1 - \cos(2x)}{2} \)
b)
Substitute \( \sin^2 x \) by \( \dfrac {1 - \cos(2x)}{2} \) in the given integral
\( I = \displaystyle \int_0^{\pi} x \sin^2(x) dx = \displaystyle \int_0^{\pi} x \left( \dfrac {1 - \cos(2x)}{2} \right) dx \)

\( = \displaystyle \dfrac{1}{2} \int_0^{\pi} x dx - \dfrac{1}{2}\displaystyle \int_0^{\pi} x \cos(2x) dx \)
Evaluate the integral on the left
\( I = \displaystyle \dfrac{1}{4} \left[x^2 \right]_0^{\pi} - \dfrac{1}{2} \int_0^{\pi} x \cos(2x) dx \)           (I)
Let \( u = x \) and \( v = \sin(2x) \) which gives \( u'= 1 \) and \( v' = 2 \cos (2x) \)
\( v' = 2 \cos (2x) \) may also be written as \( \cos (2x) = \dfrac{v'}{2} \).
We now substitute \( x \) by \( u \) and \( cos (2x) \) by \( \dfrac{v'}{2} \) in the integral in (I) and rewrite it as
\( \displaystyle \int_0^{\pi} x \cos(2x) dx = \displaystyle \dfrac{1}{2} \int_0^{\pi} u v' dx \)
We now use the given rule \( \displaystyle \int u v' dx = u v - \int u' v dx \) to rewrite the above integral as
\( \displaystyle = \dfrac{1}{2} \left[ x \sin (2x) \right]_0^{\pi} - \dfrac{1}{2} \int_0^{\pi} (1) \sin(2x) dx \)
Evaluate the integral above
\( \displaystyle = \dfrac{1}{2} \left[ x \; \sin (2x) \right]_0^{\pi} + \dfrac{1}{2} \left[ \dfrac{1}{2} \cos(2x) \right]_0^{\pi} \)
We now substitute the above in (I) and evaluate the given integral
\( I = \displaystyle \dfrac{1}{4} \left[x^2 \right]_0^{\pi} - \dfrac{1}{4} \left[ \left[ x \; \sin (2x) \right]_0^{\pi} + \left[ \dfrac{1}{2} \cos(2x) \right]_0^{\pi} \right] \)
Evaluate
\( I = \displaystyle \dfrac{1}{4}[\pi^2 - 0^2 - \dfrac{1}{4} [ \pi \sin (2\pi) - 0 \sin(0) ] - \dfrac{1}{8} [ \cos(0) - \cos (2\pi) ] \)
Simplify
\( I = \dfrac{\pi^2}{4} \)

Question 13
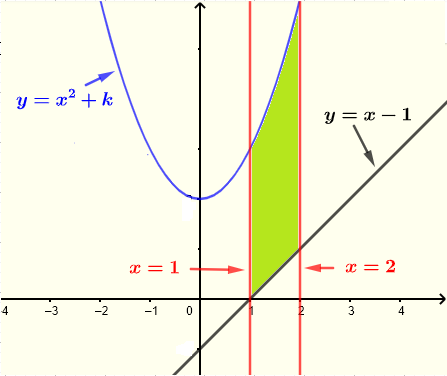
Find \( k \) so that the area of the shaded part (green) shown in the graph below is equal to \( 5 \).

Question 14
Use the first principle of differentiation to evaluate \( \sin'( \pi/4) \).
Solution
The first principle (or definition ) of differentiation gives
\( \displaystyle \sin'( \pi/4) = \lim_{h\to 0} \dfrac{\sin(\pi/4+h) - \sin(\pi/4)}{h} \)
Use the trigonometric identity \( \sin(A + B) = \sin A \cos B + \cos A \sin B \) to expand \( \sin(\pi/4+h) \) in the numerator
\( \displaystyle \sin'( \pi/4) = \lim_{h\to 0} \dfrac{ \sin (\pi/4) \cos h + \cos (\pi/4) \sin h - \sin(\pi/4)}{h} \)
Factor and rewrite as
\( \displaystyle \sin'( \pi/4) = \lim_{h\to 0} \dfrac{ \sin (\pi/4) (\cos h - 1) + \cos (\pi/4) \sin h }{h} \)
Write as sum of limits
\( \displaystyle \sin'( \pi/4) = \sin (\pi/4) \lim_{h\to 0} \dfrac{ (\cos h - 1)}{h} + \cos (\pi/4) \lim_{h\to 0} \dfrac{ \sin h }{h} \)         (I)
We use the limit of \( \lim_{h\to 0} \dfrac{\sin h}{h} = 1 \) to evaluate the limit \( \lim_{h\to 0} \dfrac{ (\cos h - 1)}{h} \).
Write
\( \cos h = \cos (h/2 + h/2) \)
and use the trigonometric identity \( \cos ( A + B ) = \cos A \cos B - \sin A \sin B \) to expand
\( \cos h = \displaystyle \cos^2(h/2) - \sin^2(h/2) \)
Use the trigonometric identity \( \cos^2(h/2) = 1 - \sin^2(h/2) \) in the above and group to obtain
\( \cos h = 1-2\sin^2(h/2) \)
Use the above to find the following limit
\( \displaystyle \lim_{h\to 0} \dfrac{ (\cos h - 1)}{h} = \lim_{h\to 0} \dfrac{ ( 1-2\sin^2(h/2) - 1)}{h} \)
Simplify numerator
\( \displaystyle \lim_{h\to 0} \dfrac{ (\cos h - 1)}{h} = \lim_{h\to 0} \dfrac{ ( -2\sin^2(h/2) )}{h} \)
Rearrange as
\( \displaystyle = - \lim_{h\to 0} \dfrac{ \sin (h/2) }{h/2} \sin(h/2) \)
Use the limits \( \lim_{h\to 0} \dfrac{ \sin (h/2) }{h/2} = 1 \) and \( \lim_{h\to 0} \sin(h/2) = 0 \) in the above to obtain
\( \displaystyle \lim_{h\to 0} \dfrac{ (\cos h - 1)}{h} = -(1)(0) = 0 \)
We now use the limits \( \lim_{h\to 0} \dfrac{ (\cos h - 1)}{h} = 0 \) and \( \lim_{h\to 0} \dfrac{\sin h}{h} = 1 \) to evaluate \( \sin'( \pi/4) \) in (I) above
\( \displaystyle \sin'( \pi/4) = \sin (\pi/4) (0) + \cos (\pi/4) (1) \)
\( = \cos (\pi/4) = \dfrac{\sqrt 2}{2} \)

Question 15
a) Let \( f(x) = \dfrac{1}{u(x)} \). Find \( f'(x) \) in terms of \( u(x) \) and \( u'(x) \) and write \( f(x) \) as an integral of an expression containing \( u(x) \) and \( u'(x) \) .
b) Use the results in part a) to evaluate \(\displaystyle \int_2^4 \dfrac{1}{x \ln^2(x)} dx \).
Solution
a)
Given: \( f(x) = \dfrac{1}{u(x)} \)
Use the rule of differentiation of the quotient to find \( f'(x) \) as follows
\( f'(x) = -\dfrac{u'(x)}{u^2(x)}\)
Take the integral of both sides to obtain
\(\displaystyle \int f'(x) dx = - \int \dfrac{u'(x)}{u^2(x)} dx + C \) , where \( C \) is the constant of integration.
The above gives
\( f(x) = - \displaystyle \int \dfrac{u'(x)}{u^2(x)} dx + C \)
b)
Let \( u(x) = \ln(x) \) which gives \( u'(x) = \dfrac{1}{x} \)
Hence the integrand in the given integral may be written as
\( \dfrac{1}{x \ln^2(x)} = \dfrac{1}{x} \dfrac{1}{\ln^2(x)} = \dfrac{u'(x)}{u^2(x)}\)
Hence
\(\displaystyle \int \dfrac{1}{x \ln^2(x)} dx = \int \dfrac{u'(x)}{u^2(x)} = - f(x) + C'\) with \( f(x) = \dfrac{1}{u(x)} \)
Hence
\(\displaystyle \int \dfrac{1}{x \ln^2(x)} dx = - \dfrac{1}{\ln x} + C' \)
We now introduce the limits of integration and evaluate the integral
\(\displaystyle \int_2^4 \dfrac{1}{x \ln^2(x)} dx = \left[- \dfrac{1}{\ln x} + C'\right]_2^4 \)
\( = -\dfrac{1}{\ln 4} + \dfrac{1}{\ln 2} = \dfrac{1}{2 \ln 2}\)

Question 16
Find all the points on the circle with radius equal to \( 2 \) and a center at \( (3,-1) \) such that the tangent to the circle at these points has a slope equal to \( 2 \)
Solution
The standard equation of a circle with a radius equal to \( 2 \) and a center at \( (3,-1) \) is given by:
\( (x-3)^2 + (y + 1)^2 = 2^2 \)         (I)
Take the derivative of both sides of equation (I)
\( 2(x-3) + 2 (y + 1) \dfrac{d y}{dx} = 0 \)         (II)
The slope of the tangent at a point on the circle is equal to the derivative \( \dfrac{dy}{dx} \) at that point.
Hence we substitute \( \dfrac{d y}{dx} \) by the given slope \( 2 \) in equation (II) above to obtain
\( 2(x-3) + 4 (y + 1) = 0 \)         (III)
We now need to solve the system of equations in (I) and (III) above
From equation (III) we may write
\( (x - 3) = - 2 (y + 1) \)         (IV)
Substitute \( (x - 3) \) by \( - 2 (y + 1) \) in equation (I) to obtain
\( (- 2 (y + 1))^2 + (y + 1)^2 = 2^2 \)
Simplify the above
\( 5 (y + 1)^2 = 2^2 \)
Solve the above for \( y \)
\( y = -1 \pm \dfrac{2}{\sqrt 5} \)
Substitute \( y \) by its values in (IV) and solve for \( x \)
\( x = 3 - 2 (-1 \pm \dfrac{2}{\sqrt 5} + 1) = 3 \mp \dfrac{4}{\sqrt 5} \)
The points on the circle at which the tangent to the circle has slope equal to \( 2 \) are
\( \displaystyle \left(3 - \dfrac{4}{\sqrt 5} , -1 + \dfrac{2}{\sqrt 5}\right) \) and \( \left( 3 + \dfrac{4}{\sqrt 5} , -1 - \dfrac{2}{\sqrt 5} \right) \)
The graphical solution to check the analytical solution given above is shwon below.