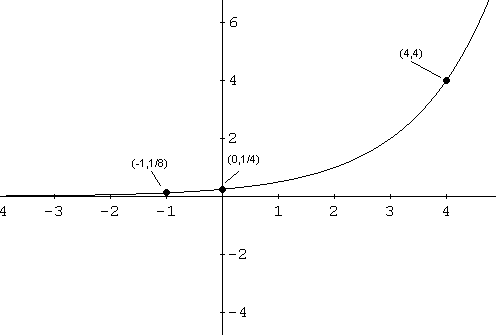Graphs of Exponential Functions

Graphing and sketching exponential functions: step by step tutorial. The properties such as domain, range, horizontal asymptotes and intercepts of the graphs of these functions are also examined in details. Free graph paper is available.


Review

We first start with the properties of the graph of the basic exponential function of base a,

f (x) = ax , a > 0 and a not equal to 1.
The domain of function f is the set of all real numbers. The range of f is the interval (0 , +?).
The graph of f has a horizontal asymptote given by y = 0. Function f has a y intercept at (0 , 1). f is an increasing function if a is greater than 1 and a decreasing function if a is smaller than 1 .

Example 1

f is a function given by
f (x) = 2(x - 2)

  1. Find the domain and range of f.
  2. Find the horizontal asymptote of the graph.
  3. Find the x and y intercepts of the graph. of f if there are any.
  4. Sketch the graph of f.

Answer to Example 1

  1. The domain of f is the set of all real numbers. To find the range of f,we start with
    2x > 0
    Multiply both sides by 2-2 (a positive number).
    2x 2-2 > 0

    Use exponential properties to rewrite the above as
    2(x - 2) > 0
    This last inequality suggests that f(x) > 0. Hence the range of f is given by the interval: (0, + ?).
  2. As x decreases without bound, f(x) = 2(x - 2) approaches 0. The graph of f has a horizontal asymptote at y = 0.
  3. To find the x intercept we need to solve the equation
    f(x) = 0
    2(x - 2)  = 0
    This equation does not have a solution, see range above, f(x) > 0. The graph of f does not have an x intercept. The y intercept is given by
    (0 , f(0)) = (0,2(0 - 2)) = (0 , 1/4).
  4. So far we have the domain, range, y intercept and the horizontal asymptote. We need extra points.
    (4 , f(4)) = (4, 2(4 - 2)) = (4 , 22) = (4 , 4)
    (-1 , f(-2)) = (-1, 2(-1 - 2)) = (-1 , 2-3) = (-1 , 1/8)
    Let us now use all the above information to graph f.

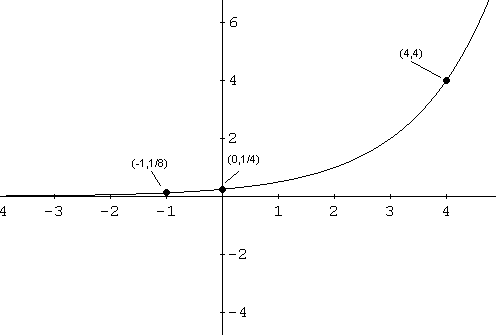
Matched Problem to Example1:   f is a function given by

f (x) = 2(x + 2)

  1. Find the domain and range of f.
  2. Find the horizontal asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Example 2

f is a function given by
f (x) = 3(x + 1) - 2
  1. Find the domain and range of f.
  2. Find the horizontal asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Answer to Example 2
  1. The domain of f is the set of all real numbers. To find the range of f, we start with
    3x > 0
    Multiply both sides by 3 which is positive.
    3x3 > 0
    Use exponential properties
    3(x + 1) > 0
    Subtract 2 to both sides
    3(x + 1) -2 > -2
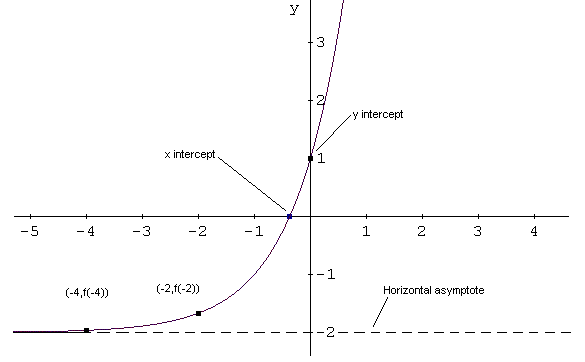
    This last statement suggests that f(x) > -2. The range of f is (-2, +?).
  2. As x decreases without bound, f(x) = 3(x + 1) -2 approaches -2. The graph of f has a horizontal asymptote y = -2.
  3. To find the x intercept we need to solve the equation f(x) = 0 3(x + 1) - 2  = 0
    Add 2 to both sides of the equation 3(x + 1) = 2
    Rewrite the above equation in Logarithmic form x +1 = log3 2
    Solve for x x = log3 2 - 1
    The y intercept is given by (0 , f(0)) = (0,3(0 + 1) - 2) = (0 , 1).
  4. So far we have the domain, range, x and y intercepts and the horizontal asymptote. We need extra points. (-2 , f(-2)) = (-2, 3(-2 + 1) - 2) = (4 , 1/3-2) = (4 , -1.67)
    (-4 , f(-4)) = (-4, 3(-4 + 1) - 2) = (-4 , 2-3) = (-4 , -1.99)
    Let us now use all the above information to graph f.

Matched Problem to Example2: f is a function given by

f (x) = 2(x - 2) + 1

  1. Find the domain and range of f.
  2. Find the horizontal asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

More References and Links to Exponential Functions and Graphing


Graphing Functions
Exponential Functions - Interactive Tutorial.
Tutorial on Exponential Functions (1)
Tutorial on Exponential Functions (2)
Graphs of Basic Functions.
Home Page