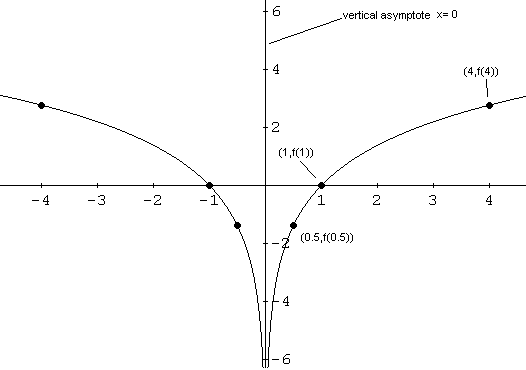Graphs of Logarithmic Functions

Graphing and sketching logarithmic functions: a step by step tutorial. The properties such as domain, range, vertical asymptotes and intercepts of the graphs of these functions are also examined in details. Free graph paper is available.


Review Properties of Logarithmic Functions

We first start with the properties of the graph of the basic logarithmic function of base a,
f (x) = log a (x) , a > 0 and a not equal to 1.
The domain of function f is the interval (0 , + ?). The range of f is given by the interval (- ? , + ?).
Function f has a vertical asymptote given by the vertical line x = 0. This function has an x intercept at (1 , 0) and f increases as x increases.
You may want to review all the above properties of the logarithmic function interactively .

Example 1

f is a function given by
f (x) = log2 (x + 2)
  1. Find the domain and range of f.
  2. Find the vertical asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Solution to Example 1
a - The domain of f is the set of all x values such that x + 2 > 0
Solve the above inequality to obtain the domain: x > - 2
The range of f is given by the interval (- ? , + ?).

b - The vertical asymptote is obtained by solving the equation: x + 2 = 0
which gives x = - 2
As x approaches -2 from the right (x > -2) , f(x) decreases without bound because there is a vertical asymptote. How do we know this?
Let us take calculate values of f as x approaches - 2 from the right (x > - 2).
f(-1) = log2 (-1 + 2) = log2(1) = 0
f(-1.5) = log2 (-1.5 + 2) = log2(1/2) = -1
f(-1.99) = log2 (-1.99 + 2) = log2(0.01) which is approximately equal to  -6.64
f(-1.999999) = log2 (-1.999999 + 2) = log2 (0.000001) which is approximately equal to  -19.93.
As we continue with values of x closer to -2, f(x) decreases without bound.

c - To find the x - intercept we need to solve the equation f(x) = 0 or log2 (x + 2) = 0
Rewrite the above equation in exponential form
x + 2 = 2 0
Then simplify and solve for x.
x + 2 = 1
x = - 1
The x intercept is at the point (-1 , 0)
The y intercept is at the point (0 , f(0)) = (0 , log2 (0 + 2)) = (0 , 1).

d - So far we have the domain, range, x and y intercepts and the vertical asymptote. We need more points.
Let us consider a point at x = -3/2 (half way between the x intercept and the vertical asymptote) and another point at x = 2.
f(-3/2) = log2 (-3/2 + 2) = log2(1/2) = log2 (2-1) = -1.
f(2) = log2 (2 + 2) = log2 (22) = 2.
We now have more information on how to graph f. The graph increases as x increases. Close to the vertical asymptote x = -2, the graph of f decreases without bound as x approaches -2 from the right. The graph never cuts the vertical asymptote. We now join the different points by a smooth curve.

Matched Problem to Example 1
f is a function given by
f (x) = log2 (x + 3)

  1. Find the domain of f and range of f.
  2. Find the vertical asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Example 2

f is a function given by
f (x) = - 3 ln (x - 4)

  1. Find the domain of f and range of f.
  2. Find the vertical asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Solution to Example 2
a - The domain of f is
the set of all x values such that x - 4 > 0
Solve the above inequality to obtain the domain
x > 4
The range of f is given by the interval (- ? , + ?).

b - The vertical asymptote is obtained by solving the equation
x - 4 = 0
Simplify and solve
x = 4
As x approaches 4 from the right (x > 4) , f(x) increases without bound. How do we know this?
Let us take some values:
f(5) = ln(5-4) = -3ln(1) = 0
f(4.001) = -3 ln(0.001) which is approximately equal to  20.72
f(4.000001) = - 3 ln(0.000001) which is approximately equal to  41.45

c - To find the x intercept we need to solve the equation f(x) = 0 or - 3 ln (x - 4) = 0
Divide both sides by -3 to obtain
ln(x - 4) = 0
Rewrite the above equation in exponential form
ln(x - 4) = e0
Then simplify
x - 4 = 1
x = 5
The x intercept is at the point (5 , 0)
The y intercept is given by (0 , f(0)). f(0) is undefined since x = 0 is not a value in the domain of f.
There is no y intercept.

d - So far we have the domain, range, x intercept and the vertical asymptote. We need extra points to be able to graph f.
f(4.5) = -3ln(4.5 - 4) approximately equal to 2.08
f(8) = -3ln(8 - 4) approximately equal to - 4.16
f(14) = -3 ln(14 - 4) approximately equal to - 6.91
Let us now sketch all the points and the vertical asymptote. Join the points by a smooth curve and f increases as x approaches 4 from the right.

Matched Problem to Example 2
f is a function given by

f (x) = 2ln (x + 5)

  1. Find the domain of f and range of f.
  2. Find the vertical asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Example 3

f is a function given by
f (x) = 2ln (| x |)

  1. Find the domain of f and range of f.
  2. Find the vertical asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

Solution to Example 3
a - The domain of f is the set of all x values such that | x | > 0.
Hence, the domain is the set of all real numbers except 0.
The range of f is the interval (- ? , + ?).

b - The vertical asymptote is obtained by solving |x | = 0
which gives
x = 0
As x approaches 0 from the right (x > 0) , f(x) decreases  without bound. How do we know this?
Let us take some values:
f(1) =2 ln(| 1 |) = 0
f(0.1) = 2 ln(0.1)  which is approximately equal to  -4.61.
f(0.0001) = 2 ln(0.0001) which is approximately equal to -18.42.
f(0.0000001) = 2 ln(0.0000001) which is approximately equal to -32.24.
As x approaches 0 from the left (x < 0) , f(x) decreases without bound. How do we know this?
Let us take some values:
f(-1) =2 ln(| -1 |) = 0
f(-0.1) = 2 ln(| -0.1 |) which is approximately equal to -4.61
f(-0.0001) = 2 ln(| -0.0001 |) which is approximately equal to -18.42
f(-0.0000001) = 2 ln(| -0.0000001 |) which is approximately equal to -32.24

c - To find the x intercept we need to solve the equation f(x) = 0 or 2 ln(| x |) = 0
Divide both sides by 2 to obtain
ln(| x |) = 0
Rewrite the above equation in exponential form
| x | = e0
Then simplify
| x | = 1
The solutions to the above equation are x = - 1 and x = 1. Hence two x intercepts at (1 , 0) and (-1 , 0)
The y intercept is given by (0 , f(0)). f(0) is undefined since x = 0 is not a value in the domain of f. There is no y intercept.

d - So far we have the domain, range, x intercept and the vertical asymptote.
By examining function f, it is easy to show that this is an even function and its graph is symmetric with respect to the y axis. Why?
f(- x) = 2 ln(| -x |) = 2 ln(| x |) = f(x)
We now find extra points.
f(4) = 2ln(| 4 |) approximately equal to 2.77
f(0.5) = 2ln(| 0.5 |) approximately equal to - 1.39
Since f is even f(-4) = f(4) and f(-0.5) = f(0.5)
Let us now plot all the points, the vertical asymptote and Join the points by a smooth curve.

Matched Problem to Example 3
f is a function given by
f (x) = -2ln (x2)

  1. Find the domain of f and range of f.
  2. Find the vertical asymptote of the graph of f.
  3. Find the x and y intercepts of the graph of f if there are any.
  4. Sketch the graph of f.

More References and Links to Logarithmic Functions and Graphing

Graphing Functions
Logarithmic Functions.
Graphs of Basic Functions.