Graphing Cubic Functions

A step by step tutorial on how to determine the properties of the graph of cubic functions and graph them. Properties, of these functions, such as domain, range, x and y intercepts, zeros and factorization are used to graph this type of functions. Free graph paper is available.

Properties of Cubic Functions

Cubic functions have the form
f (x) = a x3 + b x2 + c x + d

Where a, b, c and d are real numbers and a is not equal to 0.
The domain of this function is the set of all real numbers. The range of f is the set of all real numbers.
The y intercept of the graph of f is given by y = f(0) = d.
The x intercepts are found by solving the equation
a x3 + b x2 + c x + d = 0

The left hand side behaviour of the graph of the cubic function is as follows:
If the leading coefficient a is positive, as x increases f(x) increases and the graph of f is up and as x decreases indefinitely f(x) decreases and the graph of f is down.
If the leading coefficient is negative, as x increases f(x) decreases the graph of f is down and as x decreases indefinitely f(x) increases the graph of f is up.

Example 1

f is a cubic function given by
f (x) = x 3

  1. Find the x and y intercepts of the graph of f.
  2. Find the domain and range of f.
  3. Sketch the graph of f.

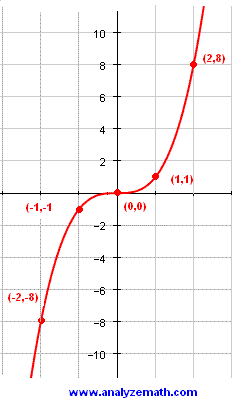
Solution to Example 1
  • a - The y intercept is given by
    (0 , f(0)) = (0 , 0)
  • The x coordinates of the x intercepts are the solutions to
    x3 = 0
  • The x intercept are at the points (0 , 0).
  • b - The domain of f (x) is the set of all real numbers.
  • Since the leading coefficient (of x3) is positive, the graph of f is up on the right and down on the left and hence the range of f is the set of all real numbers.
  • c - make a table of values and graph.

    x -2 -1 0 1 2
    f(x) = x 3 -8 -1 0 1 8
    Also since f(-x) = - f(x), function f is odd and its graph is symmetric with respect to the origin (0,0).

    plot graph of f(x) = x^3

Example 2

f is a cubic function given by
f (x) = - (x - 2) 3

  1. Find y intercepts of the graph of f.
  2. Find all zeros of f and their multiplicity.
  3. Find the domain and range of f.
  4. Use the y intercept, x intercepts and other properties of the graph of to sketch the graph of f.

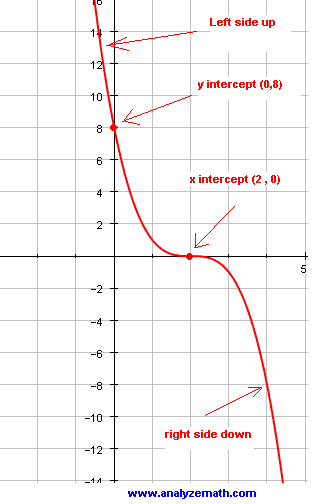
Solution to Example 2
  • a - The y intercept is given by
    (0 , f(0)) = (0 , 8)
  • b - The zeros of f are solutions to
    - (x - 2) 3 = 0
  • Function f has one zero at x = 2 of multiplicity 3 and therefore the graph of f cuts the x axis at x = 2.
  • c - The domain of f (x) is the set of all real numbers.
  • After expansion of f(x), we can see that the leading coefficient (of x3) is negative, the graph of f is down on the right and up on the left and hence the range of f is the set of all real numbers.
  • d - Properties and graph.
    At x = 2, the graph cuts the x axis. The y intercept is a point on the graph of f. Also the graph of f(x) = - (x - 2) 3
    is that of f(x) = x 3 shifted 2 units to the right because of the term (x - 2) and reflected on the x axis because of the negative sign in f(x) = - (x - 2) 3. Adding to all these properties the left and right hand behavior of the graph of f, we have the follwoing graph.

    plot graph of f(x) = f(x) = - (x - 2)^3

Example 3

f is a cubic function given by
f (x) = x 3 + 2 x 2 - x - 2

  1. Factor f(x).
  2. Find all zeros of f and their multiplicity.
  3. Find the domain and range of f.
  4. Use the y intercept, x intercepts and other properties of the graph of to sketch the graph of f.

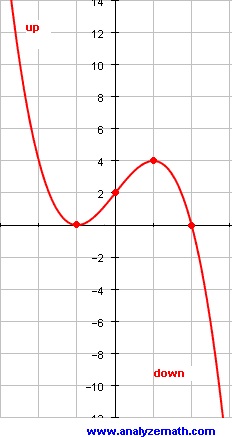
Solution to Example 3
  • a - f (x) = x 3 + 2 x 2 - x - 2 = x 2 (x + 2) - (x + 2) = (x + 2)(x 2 - 1)
  • b - The zeros of f are solutions to
    (x + 2)(x 2 - 1) = 0
  • Function f has zeros at x = - 2, at x = 1 and x = - 1. Therefore the graph of f cuts the x axis at all these x intercepts.
    c - The domain of f (x) is the set of all real numbers.
  • d - The leading coefficient f(x) is positive, the graph of f is down on the left and up on the right and hence the range of f is the set of all real numbers.
  • Properties and graph.
    The y intercept of the graph of f is at (0 , - 2). The graph cuts the x axis at x = -2, -1 and 1. Adding to all these properties the left and right hand behaviour of the graph of f, we have the following graph.
    plot graph of f(x) = x<sup> 3</sup> + 2 x<sup> 2</sup> - x - 2

Example 4

f is a cubic function given by
f (x) = - x 3 + 3 x + 2

  1. Show that x - 2 is a factor of f(x) and factor f(x) completely.
  2. Find all zeros of f and their multiplicity.
  3. Find the domain and range of f.
  4. Use the y intercept, x intercepts and other properties of the graph of to sketch the graph of f.

Solution to Example 4
  • a - The division of f(x) by x - 2 gives a quotient equal to -x2 - 2x -1 and a remainder is equal to 0. Hence
    f(x) = (x - 2)(-x2 - 2x -1) = - (x - 2)(x2 + 2x + 1) = -(x - 2)(x + 1)2
  • b - The zeros of f are solutions to
    -(x - 2)(x + 1)2 = 0
  • Function f has zeros at x = 2 and x = - 1 with multiplicity 2. Therefore the graph of f cuts the x axis at x = 2 and is tangent to the x axis at x = - 1 because the mutliplicity of this zero is even.
  • c - The domain of f (x) is the set of all real numbers.
  • The leading coefficient f(x) is negative, the graph of f is up on the left and down on the right and hence the range of f is the set of all real numbers.
  • d - Properties and graph.
    The y intercept of the graph of f is at (0 , 2). The graph cuts the x axis at x = 2 and is tangent to it at x = - 1. Adding to all these properties the left and right hand behaviour of the graph of f, we have the following graph.
    plot graph of f (x) = - x<sup> 3</sup> + 3 x + 2

More References and Links to Graphing

Graphing Functions.