Definition of the Slope of a Line

The slope of a straight line is a measure of how steep a line is and in general it is a measure of change. It is an extremely important concept in mathematics.
The three lines shown below have different properties: line \( L_1 \) is steeper than line \( L_2 \). Lines \( L_1 \)and \( L_2 \) rise but line \( L_3 \) falls. A parameter is needed to measure all these properties of a line quantitatively.



Let us consider the two points \( P_1(x_1,y_1) \) and \( P_2(x_2,y_2) \) on the line shown below. As we move from point \( P_1 \) to point \( P_2 \); to a change \( \Delta y = y_2 - y_1\) in the vertical direction corresponds a change \( \Delta x = x_2 - x_1\) in the horizontal direction.
The slope \( m \) of a line is defined as the ratio of the change along the y axis and the change of the x axis
\[ m = \dfrac{\Delta y}{\Delta x} = \dfrac {y_2 - y_1}{x_2 - x_1} \]

Properties of the Slope of a Line

Example 1 Find slopes
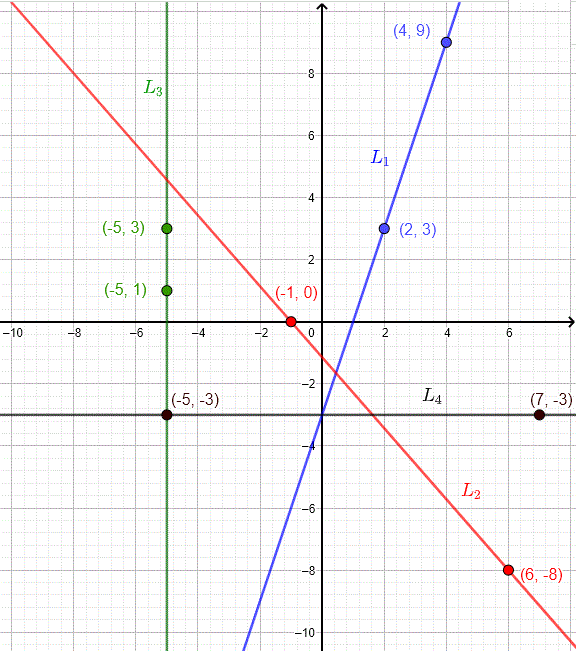
Find the slope of the lines \( L_1, L_2, L_3, L_4 \) through the given pair of points and describe the lines as rising, falling, horizontal or vertical and plot the given pair of points and the corresponding line in the same system of coordinates.

1. \( L_1: (2 , 3) , (4 , 9) \)
2. \( L_2: (-1 , 0) , (6 , -8) \)
3. \( L_3: (-5 , 1) , (-5 , 3) \)
4. \( L_4: (7 , -3) , (-5 , -3) \)

1. \( L_1: m_1 = \dfrac{9 - 3}{4 - 2} = 3\) , the line has a positive slope and therefore rises.
   
   
2. \( L_2: m_2 = \dfrac{-8 - 0}{6 - (-1)} = -\dfrac{8}{7} \) , the line has a negative slope and therefore falls.
   
   
3. \( L_3: m_3 = \dfrac{3 - 1}{- 5 - (-5) } = \dfrac{2}{0} = \text{undefined} \) , the slope is undefined and therefore the line is vertical.
   
   
4. \( L_4: m_3 = \dfrac{-3 - (-3)}{-5 - 7 } = \dfrac{0}{-12} = 0 \) , the slope is equal to 0 and therefore the line is horizontal.
   
   The graphs of the four lines and the pairs of points are shown below where all the above answers are confirmed.
   
   
   

Example 2 slopes of parallel and perpendicular lines
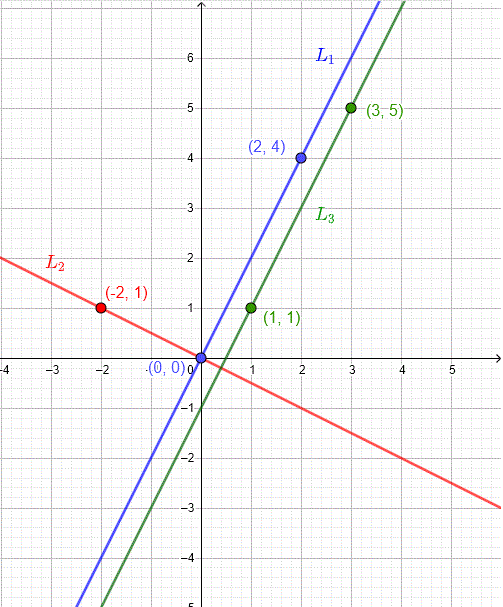
Find the slope of the lines \( L_1, L_2, L_3\) through the given pair of points and describe the lines as parallel or perpendicular. Graph the three lines in the same system of coordinates.

1. \( L_1: (0 , 0) , (2 , 4) \)
2. \( L_2: (0 , 0) , ( - 2 , 1) \)
3. \( L_3: (1 , 1) , (3 , 5) \)

1. \( L_1: m_1 = \dfrac{4 - 0}{2 - 0} = 2 \)
   
   
2. \( L_2: m_2 = \dfrac{1 - 0}{-2 - 0} = -\dfrac{1}{2} \)
   
   
3. \( L_3: m_3 = \dfrac{5 - 1}{3 - 1} = 2 \)
   
   Since \(m_1 = m_3 \) , lines \( L_1 \) and \( L_3 \) are parallel.
   Since \(m_1 \times m_2 = 2 \times (-1/2) = - 1 \) and \(m_3 \times m_2 = 2 \times (-1/2) = - 1 \), \( L_1 \) and \( L_2 \) are perpendicular and \( L_3 \) and \( L_2 \) are also perpendicular.
   The graphs of the three lines are shown below.
   
   
   

Example 3
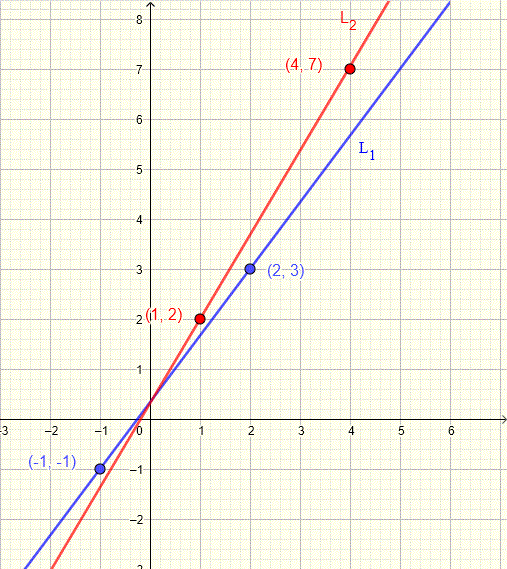
Two lines \( L_1 \) and \( L_2 \) are defined by the pair of points: \( L_1: (-1,-1), (2,3) \) and \( L_2: (1,2) , (4,7) \)
a) Find the slope of the two lines. Which of the two lines rises faster?
b) Graph the two lines and confirm graphically your answer to part a).

Solution to Example 3
a) \( L_1: m_1 = \dfrac{3 - (-1)}{2- (-1)} = \dfrac{4}{3} \)

\( L_2: m_2 = \dfrac{7 - 2}{4 - 1} = \dfrac{5}{3} \)

Both lines have positive slopes and therefore rise. But because the slope \( m_2 = \dfrac{5}{3} \) is larger than the slope \( m_1 = \dfrac{4}{3} \), line \( L_2 \) rises faster than line \( L_1 \).
b) The graphs of the two lines are shown below and the answer to part a) is confirmed.