Difference Quotient
What is the difference quotient in calculus ?
We start with the definition and then we calculate the difference quotient for different functions as examples with detailed explanations.
Note that a difference quotient calculator is included and may be used to check results and generate further practice.
Definition of Difference Quotient
Let \( f \) be a function whose graph is shown below.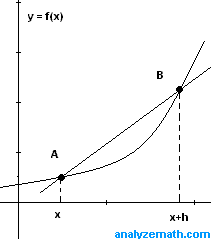
A and B are points on the graph of \( f\). A line passing trough the two points \( A ( x , f(x)) \) and \( B(x+h , f(x+h)) \) is called a secant line. The slope \( m \) of the secant line may be calculated as follows:
\[ m = \dfrac{f (x + h) - f(x)}{(x + h) - x} \]
Simplify the denominator to obtain
\[ m = \dfrac{f (x + h) - f(x)}{h} \]
The slope \( m \) is called the difference quotient . It is a very important concept in calculus where it is used to define the derivative of function \( f \) which in fact defines the local variation of a function in mathematics.
Examples with Solutions
In the examples below, we calculate and simplify the difference quotients of different functions.
Example 1
Find the difference quotient of function \( f \) defined by \[f(x) = 2x + 5\]
Solution to Example 1
-
We first need to calculate \( f(x + h) \).
\( f(x + h) = 2(x + h) + 5 \)
-
We now substitute \( f(x + h) \) and \( f(x) \) in the definition of the difference quotient by their expressions
\( \dfrac{f (x + h) - f(x)}{h} = \dfrac{2(x + h) + 5 - (2 x + 5) }{h} \) -
We simplify the above expression.
\( = \dfrac{2h}{2} = 2 \) -
The answer is 2 which also the slope of the line defined by function \( f \), why?
Example 2
Find the difference quotient of the following function\[ f(x) = 2x^2 + x - 2 \]
Solution to Example 2
-
We first calculate \( f(x + h) \).
\( f(x + h) = 2(x + h)^2 + (x + h) - 2 \) -
We now substitute \( f(x + h) \) and \( f(x) \) in the difference quotient
\( \dfrac{f (x + h) - f(x)}{h} = \dfrac{ 2(x + h)^2 + (x + h) - 2 - ( 2 x^2 + x - 2 )}{h} \) -
We expand the expressions in the numerator and group like terms.
\( = \dfrac{ 4 x h + 2 h^2 + h}{h} = 4 x + 2 h +1 \)
Example 3
Find the difference quotient of function \( f \) given by \[ f(x) = \sin x \] and write the result as a product.
Solution to Example 3
-
We first calculate \( f(x + h) \).
\( f(x + h) = \sin (x + h) \)
-
We now substitute \( f(x + h) \) and \( f(x) \) in the difference quotient
\( \dfrac{f (x + h) - f(x)}{h} = \dfrac{ \sin (x + h) - \sin x}{h} \) -
We use the trigonometric formula that transform a difference \( \quad \sin (x + h) - \sin x \quad \) into a product.
\( \sin (x + h) - \sin x = 2 \cos [ (2 x + h)/2 ] \sin (h/2) \)
-
We substitute the above expression for \( sin (x + h) - sin x \) in the difference quotient above to obtain.
\( \dfrac{f (x + h) - f(x)}{h} = \dfrac{ 2 \cos [ (2 x + h)/2 ] \sin (h/2)}{h} \)
More References and links
Difference Quotient Calculatordifferentiation and derivatives
Difference quotient
