A set of Calculus 1 questions with their detailed solutions to practice for tests, exams, placement exams, ... and gain deep understanding on the following topics:
Functions
Limits
Continuity
Derivatives
Applications of Derivatives
The questions were designed to cover the most important topics in calculus 1 and their detailed solutions include links to more practice in these topics.
Question 1
Find the domain of function
Question 2
Find the range of function
Question 3
Find the inverse of function
Question 4
Evaluate each of the following limits
\( \)\( \)\( \)\( \)
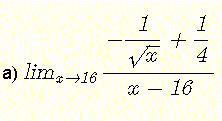
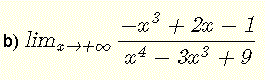
Question 5
a) Graph \( y = e^{x-1} \) and \( y = x \) in the same system of coordinates and then show that the two graphs are tangent at the point \( (1,1) \) and that \( e^{x-1} \ge x \)
b) Use the result obtained in part a) to determine the concavity of the function \( f(x) = \dfrac{x^3}{6} - e^{x-1} \) and inflection points if any.
Question 6
Find the derivative of the following functions and do not simplify the final answer.
Question 7
Find equation of the tangent line to the curve with equation \( \sin(y^2) = x^2 \) at the point \( ( 0,\sqrt{\pi}) \).
Question 8
Find the constants \( a \) and \( b \) so that the function \( f \) is continuous on \( (-\infty , +\infty ) \)
\( f(x) = \left\{
\begin{array}{ll}
2x - 1 & x\le 1 \\
a x^3 + b & 1 \lt x \lt 2 \\
x + 2 b & x\ge 2 \\
\end{array}
\right. \)
Question 9
Find the equation of the tangent line to the curve with equation \( y = x + \sin(x) \) at \( x = 0 \).
Question 10
Use the definition of the derivative as a limit to find the derivative \( f' \) where \( f(x) = \sqrt{x+2} \).
Question 11
Determine on what interval(s) is the function \( f(x) = e^x(x^2-5x+8)+\dfrac{x^4}{12}-\dfrac{x^3}{6} \) concave up and concave down and any point of inflection.
Question 12
Use Newton's method with initial approximation \( x_1 = 2 \) to find a second approximation to the solution of the equation \( e^x = x^3 \).
Question 13
Find the absolute maximum and minimum of the function \( f(x) = x^4 - x^3 \) on the interval \( [0,5] \).
Question 14
If the dimensions \( L \),\( W \) and \( H\) of a rectangular box are changing at the rates \( \dfrac{dL}{dt} = 0.1 \; cm/sec \), \( \dfrac{dW}{dt} = - 0.2 \; cm/sec\) and \( \dfrac{dH}{dt} = 0.3 \; cm/sec\), at what rate is the volume of the box
changing when \( L = 20 \; cm \), \( W = 8 \; cm \) and \( H = 5 \; cm \)?
Question 15
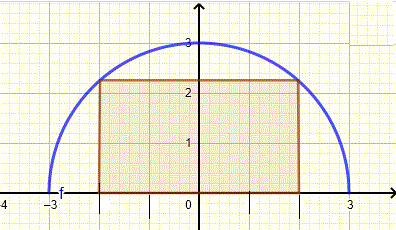
What are the dimensions of the rectangle with the largest area that can be inscribed in a semicircle of radius 3?
Detailed Solutions to the Above Questions
Solution to Question 1
For function \( f \) to take real values, the expression under the radical in the numerator must be non negative, and the expression under the radical in the denominator must be positive; hence the inequalities to solve
\( x - 1 \ge 0 \) and \( 4 - x^2 \gt 0 \)
The solution sets for the first and second inequalities are respectively
\( x \ge 1 \) and \( -2 \lt x \lt 2 \)
Both inequalities must be satisfied simultaneously, therefore the domain of the given function is the intersection of the sets \( x \ge 1 \) and \( -2 \lt x \lt 2 \) which is given by
\( 1 \le x \lt 2 \)
Solution to Question 2
According to the properties of inverse fucntions, one way to find the range of the given function is to find the domain of its inverse.
Let us first prove that \( f \) is a one to one function and then find its inverse. To prove that \( f \) is a one to one function and hence invertible, we use the contrapositive of the one to one function and start with
\( f(a) = f(b) \) and prove that \( a = b \). Hence
\( \dfrac{a - 1}{2-3 a} = \dfrac{b - 1}{2-3 b} \)
Cross multiply
\( (a - 1)(2-3 b) = (2 - 3 a)(b - 1) \)
Expand
\( 2a - 2 - 3 a b + 3 b = 2 b - 2 - 3 ab + 3 a \)
Group like terms
\( 2 a = 2 b \)
Solve for a
\( a = b\)
which proves that function \( f \) is a one to one function and therefore has an inverse
The inverse of \( f \) may be calculated starting with the equation
\( y = \dfrac{x - 1}{2-3x} \)
Cross multiply the above equation
\( 2 y - 3 x y = x - 1 \)
and solve for \( x \)
\( x = \dfrac{2 y + 1}{3y + 1} \)
Interchange \( x \) and \( y \) in the above equation to obtain the inverse \( f^{-1} \)
\( f^{-1}(x) = y = \dfrac{2 x + 1 }{3x + 1} \).
The domian of \( f^{-1} \) is the set of all real numbers except \( -\dfrac{1}{3} \). Hence the range of \( f \) is the set of all real numbers except \( -\dfrac{1}{3} \) which may be written in interval form as
\( (-\infty , - \dfrac{1}{3}) \cup (- \dfrac{1}{3} , +\infty) \)
Solution to Question 3
Write the function as an equation as follows
\( y = ln (2x - 3) + 2 \)
Solve the above for x
\( y - 2 = ln (2x - 3) \)
\( 2x - 3 = e^{y - 2} \)
\( 2 x = e^{y - 2} + 3 \)
\( x = \dfrac{1}{2} (e^{y - 2} + 3) \)
Interchange \( x \) and \( y \)
\( y = \dfrac{1}{2} (e^{x - 2} + 3) \)
The inverse of \( f \) is given by
\( f^{-1}(x) = \dfrac{1}{2} (e^{x - 2} + 3) \)
Solution to Question 4
a)
The limit is of the indeterminate form \( \dfrac{0}{0} \).
Multiply numerator and denominator by the conjugate of the numerator \( -\dfrac{1}{\sqrt x} - \dfrac{1}{4} \)
b)
The limit is of the indeterminate form \( \dfrac{\infty}{\infty} \).
Divide all terms in the numerator and all terms of the denominator by the term with the highest power which in \( x^4 \)
\( \lim_{x\to +\infty} \dfrac{-x^3+2x-1}{x^4 - 3 x^3 + 9 } = \lim_{x\to +\infty} \dfrac{\dfrac{-x^3}{x^4}+\dfrac{2x}{x^4}-\dfrac{1}{x^4}}{\dfrac{x^4}{x^4} - \dfrac{3 x^3}{x^4} + \dfrac{9}{x^4} }\).
Simplify rational terms
\( = \lim_{x\to +\infty} \dfrac{\dfrac{-1}{x}+\dfrac{2}{x^3}-\dfrac{1}{x^4}}{1 - \dfrac{3}{x} + \dfrac{9}{x^4} } = \dfrac{0+0-0}{1 - 0 + 0 } = \dfrac{0}{1} = 0\)
c)
The limit is of the indeterminate form \( \infty \cdot 0 \).
Let \( t = \dfrac{3}{x} \) and rewrite the limit in terms of t.
\( \lim_{x\to +\infty} x \sin(\dfrac{3}{x}) = \lim_{t\to 0} 3 \dfrac {\sin(t)}{t} \).
Using the well known result \( \lim_{t\to 0} \dfrac {\sin(t)}{t} = 1 \), the limit evaluates to
\( = 3 \times 1 = 3\)
d)
\( \lim_{x\to 0} \dfrac{\sin(x)+x}{2x^2+x} = \dfrac{0}{0}\), indeterminate form
Use the a L'Hospital's Rule to the indeterminate form, we can write
\( \lim_{x\to 0} \dfrac{\sin(x)+x}{2x^2+x} = \lim_{x\to 0} \dfrac{ d (\sin(x)+x) / dx }{ d(2x^2+x)/dx} = \lim_{x\to 0} \dfrac{ cos(x)+ 1 }{ 4x+1} = \dfrac{ cos(0)+ 1 }{ 4\times 0+1} = 2\)
e)
\( \lim_{x\to +\infty} \dfrac{\sin(x)+1}{x} \).
It is well known that the range of \( \sin(x) \) is given by
\( -1 \le \sin(x) \le 1 \)
Add 1 to all terms of the inequality to obtain the following inequality
\( -1 + 1 \le \sin(x) + 1 \le 1 + 1 \)
\( 0 \le \sin(x) + 1 \le 2 \)
Divide all terms of the above inequality by positive \( x \)
\( \dfrac{0}{x} \le \dfrac{\sin(x) + 1}{x} \le \dfrac{2}{x} \)
We have \( \lim_{x\to +\infty} \dfrac{0}{x} = 0 \) and \( \lim_{x\to +\infty} \dfrac{2}{x} = 0 \)
Using the squeezing (or Sandwich) theorem, we can evaluate the given limit as follows
\( \lim_{x\to +\infty} \dfrac{\sin(x)+1}{x} = 0 \)
Solution to Question 5
a)
The graph of \( y = e^{x-1} \) and \( y = x \) are shown below. The derivative of \( y = e^{x-1} \) is equal to \( y' = e^{x-1} \) and the slope \( m \) of the tangent at \( x = 1 \) is the value of the derivative at \( x = 1\). Hence
\( m = y'(1) = e^{1-1}= 1 \)
The equation of the tangent line at the point \( (1,1) \) is given by
\( y - 1 = 1 \times (x - 1) \)
Which simplifies to
\( y = x \)
Hence the graphs of \( y = e^{x-1} \) and \( y = x \) are tangent at the point \( (1,1) \) and we can therefore state graphically that \( e^{x-1} \ge x \).
b)
The first and second derivatives of \( f \) are given by
\( f'(x) = \dfrac{x^2}{2}-e^{x-1} \)
\( f''(x) = x-e^{x-1} \)
A point of inflection occurs at a value of \( x \) where \( f''(x) \) change sign. We have seen in part a) that \( e^{x-1} \ge x \) which may be written as
\( x-e^{x-1} \le 0 \)
and therefore \( f''(x) \) is negative and has a zero at \( x = 1 \). Hence the graph of \( f(x) \) is concave down and does not have a point of inflection because \( f''(x) \) does not change sign.
Solution to Question 6
a) Sum rule of derivatives gives: \( f'(x) = e^{x-1} + \dfrac{3}{3x-1} + 2 \cos(2x+1) \)
b) Product of derivatives rule: \( g'(x) = 4(2x-1)(\tan(x)-1) + (2x-1)^2(\sec^2(x)) \)
c) quotient of derivatives rule: \( h'(x) = \dfrac{ (1 + \sin(x))(x^2-2x+1) - (x - \cos(x))(2x-2) }{(x^2-2x+1)^2} \)
d) Let \( u = \sqrt{x^3 - \dfrac{1}{x} + 2} \) , write function \(m \) as \(m = \sin u \) then use the chain rule of derivatives
e)
Rewrite \( 3^{ 2x+3} \) and \( \log_3(2x-1) \) as
\( 3^{2x+3} = e^{(2x+3) \ln 3}\) , change of base of exponentials
\( \log_3(2x-1) = \dfrac{ \ln(2x-1)}{ \ln 3}\) , change of base of logarithms
Substitute and rewrite \( n(x) \) as
\( n(x) = 3^{ 2x+3} + \log_3(2x-1) = e^{(2x+3) \ln 3} + \dfrac{ \ln(2x-1)}{ \ln 3} \)
We now calculate the derivative
\( n'(x) = ( 2 \ln 3 ) e^{(2x+3) \ln 3} + \dfrac{1}{ \ln 3} \dfrac{2}{2x-1} = ( 2 \ln 3 ) 3^{2x+3} + \dfrac{2}{\ln 3(2x-1)} \)
Solution to Question 7
We first differentiate the given equation implicitly
\( 2 y \dfrac{d y}{d x} cos(y^2) = 2 x \)
\( \dfrac{d y}{d x} = \dfrac{ x}{ y cos(y^2)} \)
The slope \( m \) of the tangent line is given by the value of \( \dfrac{d y}{d x} \) at the point \( ( 0,\sqrt{\pi}) \).
\( m = \dfrac{(0)}{\sqrt{\pi} cos((\sqrt{{\pi}})^2)} = 0 \)
The equation, in point slope form, of the tangent line to the curve at the point \( ( 0,\sqrt{\pi}) \) is given by
\( y - \sqrt{\pi} = 0(x - 0) \)
It is a horizontal line given by
\( y = \sqrt{\pi} \)
Solution to Question 8
\( f(x) \) is continuous on the intervals \( (-\infty , 1) \) , \( (1,2) \) and \( (2 , +\infty) \). We need to find \( a \) and \( b \) so that it is also continous at \( x = 1 \) and \( x = 2 \) and therefore continuous on \( (-\infty , +\infty ) \).
\( f(1) = 1 \)
\( \lim_{x\to 1^-} f(x) = 1 \)
\( \lim_{x\to 1^+} f(x) = a(1)^3+b = a + b \)
The limits from the left and right of \( 1 \) must be equal
\( a + b = 1 \) (equation 1)
\( f(2) = 2 + 2 b \)
\( \lim_{x\to 2^-} f(x) = a(2)^3 + b = 8 a + b \)
\( \lim_{x\to 2^+} f(x) = 2 + 2 b \)
The limits from the left and right of \( 2 \) must be equal
\( 8 a + b = 2 + 2 b \) (equation 2)
Solve equations (1) and (2) simultaneously to find
\( a = \dfrac{1}{3} \) and \( b = \dfrac{2}{3} \)
Solution to Question 9
Find the derivative of \( y \).
\( y' = 1 + \cos(x) \)
The slope \( m \) of the tangent at \( x = 0 \) is equal to the value of \( y' \) at \( x = 0 \). Hence
\( m = 1 + \cos(0) = 2 \)
The y coordinate of the point of tangency \( P \) is given by the value of \( y \) at \( x = 0 \). Hence
\( P(0 , 0 + \sin(0)) = P(0,0) \)
The equation of the tangent line in point slope form is given by
\( y - 0 = 2(x - 0) \)
and in slope intercept form
\( y = 2 x \)
Solution to Question 10 Definition of the derivative \( f' \) of function \( f \) is given by the limit
\( f'(x) = \lim_{h\to 0} \dfrac{f(x+h)-f(x)}{h} \)
Substitute \( f(x) \) by \( \sqrt{x+2} \) in the above definition to obtain
\( f'(x) = \lim_{h\to 0} \dfrac{\sqrt{x + h +2}- \sqrt{x+2} }{h} \)
The above limit is of the indeterminate form \( \dfrac{0}{0} \). Multiply the numerator and denominator by the conjugate of the numerator
\( f'(x) = \lim_{h\to 0} \dfrac{ (\sqrt{x + h +2}- \sqrt{x+2} ) (\sqrt{x + h +2} + \sqrt{x+2} ) }{h(\sqrt{x + h +2} + \sqrt{x+2} )} \)
Expand the numerator and simplify
\( f'(x) = \lim_{h\to 0} \dfrac{ (x + h + 2)- (x + 2) ) }{h(\sqrt{x + h +2} + \sqrt{x+2} )} = \lim_{h\to 0} \dfrac{ h }{h(\sqrt{x + h +2} + \sqrt{x+2} )}\)
Divide numerator and denominator by \( h \) (or cancel \( h \))
\( f'(x) = \lim_{h\to 0} \dfrac{ 1 }{\sqrt{x + h +2} + \sqrt{x+2} }\)
Evaluate the limit and hence the derivative
\( f'(x) = \dfrac{ 1 }{ \sqrt{x + 0 + 2} + \sqrt{x + 2} } = \dfrac{ 1 }{2 \sqrt{x + 2} }\)
Solution to Question 11
Find the first and second derivatives
\( f'(x) = e^x (x^2-5x+8) + e^x (2 x -5) +\dfrac{x^3}{3}-\dfrac{x^2}{2} = e^x (x^2-3x+3)+\dfrac{x^3}{3}-\dfrac{x^2}{2} \)
\( f''(x) = e^x(x^2-3x+3)+e^x(2x-3) + x^2-x = e^x(x^2-x)+x^2-x = x (x - 1) e^x \)
\( f'' \) has two zeros: \( x = 0 \) and \( x = 1\) and \( e^x \) is always positive. Hence the table of signs of \( f'' \) has three intervals
1) \( (-\infty , 0 ) \) , test value \( x = -1 \) , \( f''(-1) = 2/e \) , hence \( f''(x) \) is positive on the interval \( (-\infty , 0 ) \).
2) \( (0 , 1 ) \) , test value \( x = 1/2 \) , \( f''(1/2) = -\dfrac{\sqrt 2}{4} \) , hence \( f''(x) \) is negative on the interval \( (0 , 1 ) \).
3) \( (1 , +\infty ) \) , test value \( x = 2 \) , \( f''(2) = 2e^2 \) , \( f''(x) \) is positive on the interval \( (1 , +\infty ) \).
\( f'' \) is concave up on the intervals \( (-\infty , 0 ) \) and \( (1 , +\infty ) \) , and concave down on the interval \( (0 , 1 ) \).
\( f'' \) changes sign at \( x = 0 \) and \( x = 1 \) and therefore has points of inflection at \( x = 0 \) and \( x = 1 \).
Solution to Question 12 Newton's method is based on the following algorithm: knowing an approximation \( x_n \) to the solution of an equation \( f(x) = 0 \), the next approximation \( x_{n+1}\) to the equation is given by
\( x_{n+1} = x_n - \dfrac{f(x_n)}{f'(x_n)} \).
The solution to the given equation \( e^x = x^3 \) is equal to the solution of the equation \( f(x) = e^x - x^3 = 0 \)
\( f'(x) = e^x - 3x^2 \)
We know a first approximation \( x_1 = 2 \); using Newton's algorithm we approximate \( x_2\) by
\( x_{2} = x_1 - \dfrac{f(x_1)}{f'(x_1)} = 2 - \dfrac{e^2 - 2^3}{e^2 - 3 \times 2^2} \approx 1.87 \) (rounded to two decimal places)
An approximation \(x_3\) may be obtained using \( x_2 \) found above and so on.
Solution to Question 13
Find the first derivative and factor it.
\( f'(x) = 4 x^3 - 3 x^2 = x^2(4x - 3)\)
\( f'(x) \) has two zeros at \( x = 0 \) and \( x = 3/4 \) and both are within the interval \( [0,5] \). The zeros of \( f'(x) \) are called critical points.
We now evaluate the function at the endpoints of the given interval and the zeros of \( f'(x) \).
\( f(0) = 0 \)
\( f(5) = 5^4 - 5^3 = 500 \)
\( f(3/4) = (3/4)^4 - (3/4)^3 = -\dfrac{27}{256} \)
Comparing these values, \( f(x) \) has an absolute maximum equal to \(500 \) at the endpoint \( x = 5 \) and an absolute minimum equal to \( -\dfrac{27}{256} \) at the critical point \( x = 3/4 \)
More questions on absolute minimum and maximum of a function included.
Solution to Question 14
The volume \( V \) of rectangular box with dimensions \( L \),\( W \) and \( H\) is given by
\( V = L(t) W(t) H(t) \)
where \( L(t)\) , \( W(t) \) and \( H(t) \) are functions of time \( t \).
Since the dimensions of the box change with time, the volume of the box is also changing with time at the rate of change of \( V\) is given by the first derivative \( \dfrac{dV}{dt} \).
\( \dfrac{dV}{dt} = W(t) H(t) \dfrac{d L}{dt } + L(t) H(t) \dfrac{d W}{dt } + L(t) W(t) \dfrac{d H}{dt } \)
Substitute the known quantities by their numerical values
\( \dfrac{dV}{dt} = 8 \times 5 \times 0.1 + 20 \times 5 \times (-0.2) + 20 \times 8 \times 0.3 = 32 \; cm^3 / sec \)
Solution to Question 15 Equation of a circle with radius \( 3 \) and center at \( (0 , 0) \)given by
\( x^2 + y^2 = 3^2 \)
Solve the above equation fo y
\(y^2 = 9 - x^2\)
\( y = \pm \sqrt{9 - x^2} \)
The equation for the upper semi circle is
\( y = \sqrt(9-x^2) \)
A point on the semi circle with x coordinate \( x \) has a y coordinate equal to \(\sqrt{9 - x^2} \) (see graph below)
The rectangle has a length \( L = 2x \) and a width (or height) \( W = \sqrt(9-x^2) \). The area \( A \) of the rectangle is given by
\( A(x) = L \times W = 2 x \sqrt(9-x^2) \) , \( 0 \le x \le 3 \)
Find first derivative of \( A \)
\( \dfrac{d A}{dx} = 2\sqrt{9-x^2} + (2x) (\dfrac{1}{2}) (-2x) (9-x^2)^{-1/2} =\dfrac{2\left(-2x^2+9\right)}{\sqrt{9-x^2}} \)
Zeros of \( \dfrac{d A}{dx} \) are given by the zeros of the numerator of \( \dfrac{d A}{dx} \)
\( -2x^2+9 = 0 \)
Gives two zeros: \( x = \sqrt{4.5} \) and \( x = - \sqrt{4.5} \)
We consider the zero at \( x = \sqrt{4.5} \) since it is within the interval \( [0 , 3 ] \)
We now evaluate the area \( A(x) \) at the endpoints \( x = 0 \) and \( x = 3 \) and at the critical point \( x = \sqrt{4.5} \).
\( A(0) = 0 \)
\( A(3) = 0 \)
\( A(\sqrt{4.5}) = 9 \)
The area A is maximum is for \( x = \sqrt{4.5} \)
The dimensions are:
\( L = 2 x = 2 \sqrt{4.5} \approx 4.24 \)
\( W = \sqrt(9-x^2) = \sqrt(9-\sqrt{4.5}^2) \approx 2.12 \)
More optimization problems are included.
