Solve Problems Using Trigonometric Ratios

A set of problems, that may be solved using the trigonometric ratios, is presented. Detailed solutions and explanations are included.

Definition of Trigonometric Ratios


right triangle ratios

In a right triangle, the six trigonometric ratios; the sine ratio, the cosine ratio, the tangent ratio, the cosecant ratio, the secant ratio and the cotangent ratio are defined as follows:
1 - The sine of angle A = sin (A) = side opposite angle A / hypotenuse = a / c
2 - The cosine of angle A = cos (A) = side adjacent to angle A / hypotenuse = b / c
3 - The tangent of angle A = tan (A) = side opposite angle A / side adjacent to angle A = a / b
4 - The secant of angle A = sec (A) = hypotenuse / side adjacent to angle A = c / b
5 - The cosecant of angle A = csc (A) = hypotenuse / side opposite to angle A = c / a
6 - The cotangent of angle A = cot (A) = side adjacent to angle A / side opposite angle A = b / a

Problems

Problem 1
Given the right triangle below, find
sin A, cos A, tan A, sec A, csc A and cot A.

right triangle in problem 1
Solution to Problem 1:
First we need to find the hypotenuse using Pythagora's theorem.
(hypotenuse) 2 = 8 2 + 6 2 = 100
and hypotenuse = 10
We now use the definitions of the six trigonometric ratios given above to find sin A, cos A, tan A, sec A, csc A and cot A.
sin A = side opposite angle A / hypotenuse = 8 / 10 = 4 / 5
cos (A) = side adjacent to angle A / hypotenuse = 6 / 10 = 3 / 5
tan (A) = side opposite angle A / side adjacent to angle A
= 8 / 6 = 4 / 3
sec (A) = hypotenuse / side adjacent to angle A = 10 / 6
= 5 / 3
csc (A) = hypotenuse / side opposite to angle A
= 10 / 8 = 5 / 4
cot (A) = side adjacent to angle A / side opposite angle A
= 6 / 8 = 3 / 4





Problem 2
Find c in the figure below.

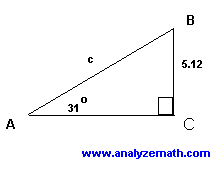right triangle in problem 2
Solution to Problem 2:
We are given angle A and the side opposite to it with c the hypotenuse. The sine ratio gives a relationship between the angle, the side opposite to it and the hypotenuse as follows
sin A = opposite / hypotenuse
Angle A and opposite side are known, hence
sin 31 o = 5.12 / c
Solve for c
c = 5.12 / sin 31 o
and use a calculator to obtain
c (approximately) = 9.94



Problem 3
If x is an acute angle of a right triangle and sin x = 3 / 7, find the exact value of the trigonometric functions cos x and cot x.
Solution to Problem 3:
If sin x = opposite / hypotenuse = 3 / 7, then we can say that opposite = 3 and hypotenuse = 7 and find the adjacent side using Pythagora's theorem.
hypotenuse 2 = adjacent 2 + opposite 2
7 2 = adjacent 2 + 3 2
adjacent = √ (40) = 2 √ (10)
We now use trigonometric ratios to find
cos x = adjacent / hypotenuse = 2 √ (10) / 7
cot x = adjacent / opposite = 2 √ (10) / 3



Problem 4
Find the exact values of x and y.

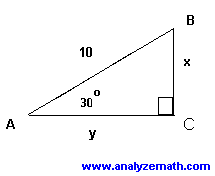right triangle in problem 2
Solution to Problem 4:
The sine function involves x and the hypotenuse as follows.
sin 30 o = x / 10
Use sin 30 o = 1 / 2 ( see table of special angles) to find x
x = 5
We now use Pythagora's theorem and write
10 2 = 5 2 + y 2
We now solve for y to obtain
y = √(75) = 5 √ (3)



Problem 5
If x is an acute angle and tan x = 5, find the exact value of the trigonometric functions sin x and cos x.
Solution to Problem 5:
If tan x = opposite / adjacent = 5 = 5 / 1, then we can say that opposite = 5 and adjacent = 1 and find the hypotenuse using Pythagora's theorem.
(hypotenuse) 2 = adjacent 2 + opposite 2
(hypotenuse) 2 = 1 2 + 5 2
hypotenuse = √ (26)
We now use trigonometric ratios to find
sin x = opposite / hypotenuse = 5 / √ (26)
cos x = adjacent / hypotenuse = 1 / √ (26)

.

More references on solving problems related to trigonometry and geometry